Vector addition is a fundamental concept in fields such as physics, engineering, and computer science, enabling the combination of multiple vectors into a single vector known as the resultant vector. The Vector Addition Calculator Magnitude and Angle is a specialized tool designed to effortlessly calculate the magnitude (length) and direction (angle) of this resultant vector, streamlining tasks ranging from simple homework problems to complex engineering projects.
Formula
Understanding the calculation process is crucial for anyone working with vectors. The formulas for determining the magnitude and angle of the resultant vector are straightforward yet powerful:
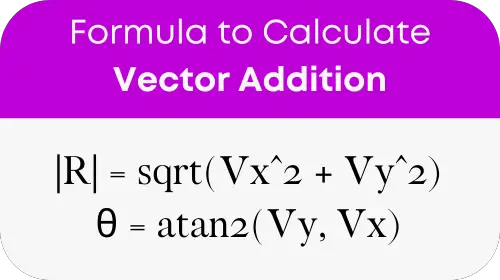
- Magnitude (|R|) of the resultant vector:
- Angle (θ) of the resultant vector:
In these formulas, Vx represents the sum of the x-components of the vectors, while Vy is the sum of the y-components. The atan2 function calculates the angle by finding the arctangent of the quotient of Vy and Vx, providing the direction of the resultant vector in radians or degrees, depending on your calculation settings.
Vector Addition Scenarios and Guidelines
| Scenario | Description | General Approach |
|---|---|---|
| Wind Vectors | Combining two wind vectors to find the resultant wind direction and speed. | Use vector addition to combine the magnitudes (speeds) and directions (angles) of the two wind vectors. |
| Force Vectors | Determining the net force on an object by adding multiple force vectors. | Sum up the components of all forces acting in the x and y directions separately, then use these sums for magnitude and angle calculations. |
| Displacement Vectors | Calculating the total displacement of an object moving along two or more paths. | Add the x and y components of each displacement vector, then find the resultant vector’s magnitude and direction. |
| Velocity Vectors | Finding the resultant velocity of an object when it has multiple velocity vectors due to different movements. | Combine the velocity vectors by adding their x and y components, then calculate the magnitude and direction of the resultant velocity. |
Example
Let’s apply the formulas to a real-world example. Imagine you have two vectors: A (3, 4) and B (1, 2). Using the magnitude and angle formulas, we can calculate the resultant vector’s magnitude and direction, illustrating the simplicity and efficiency of the Vector Addition Calculator.
Most Common FAQs
Vector addition combines two or more vectors into a single vector, called the resultant vector, which represents the cumulative effect of all vectors involved.
Input the components of your vectors into the calculator, and it will automatically provide the magnitude and angle of the resultant vector.
Yes, the method applies to any number of vectors. Sum all x and y components separately before applying the formulas.