The Leibniz Rule Calculator computes the nth derivative of the product of two functions using the Leibniz rule. This rule is essential in calculus for handling higher-order derivatives of products, making it a valuable resource for solving complex mathematical problems.
Formula of Leibniz Rule Calculator
The Leibniz rule is used to find the nth derivative of a product of two functions. The formula is:
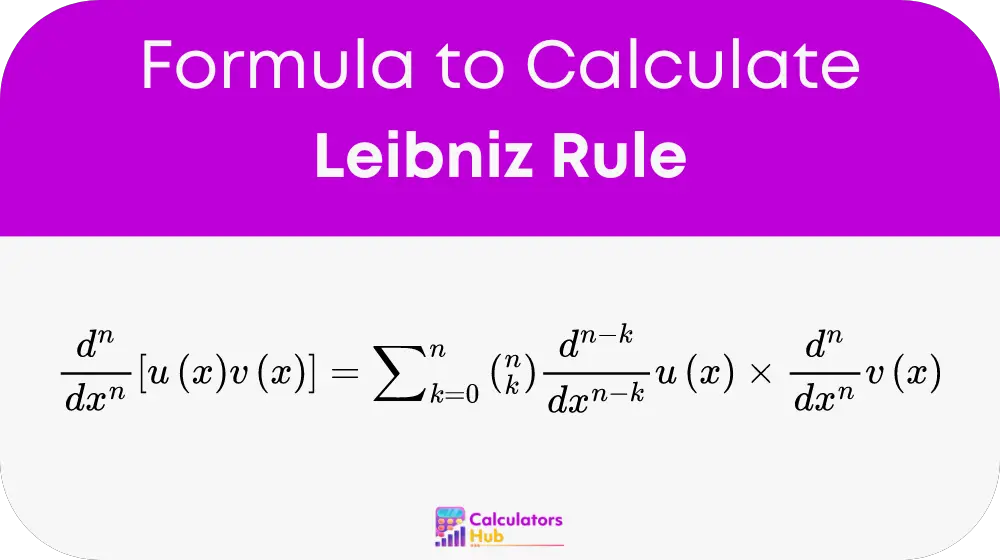
where:
- n is the order of the derivative
- C(n, k) is the binomial coefficient, given by C(n, k) = n! / (k! * (n - k)!)
- (d^k/dx^k) v(x) is the k-th derivative of v(x)
- (d^(n - k)/dx^(n - k)) u(x) is the (n - k)-th derivative of u(x)
This formula helps in finding higher-order derivatives of products of functions.
Common Terms and Conversions Table
Below is a table of commonly searched terms related to the Leibniz rule and their corresponding explanations:
| Term | Explanation |
|---|---|
| Derivative | The rate at which a function is changing at any given point |
| Higher-order Derivative | Derivatives of derivatives, indicating rates of change of rates of change |
| Binomial Coefficient (C(n,k)) | A coefficient giving the number of ways to choose k elements from n elements |
| Product of Functions | A function that is the result of multiplying two other functions |
| nth Derivative | The derivative taken n times |
Example of Leibniz Rule Calculator
Consider the functions u(x) = x^2 and v(x) = e^x. We want to find the 3rd derivative of their product using the Leibniz rule.
Using the formula:
d^3/dx^3 [x^2 e^x] = sum from k=0 to 3 of C(3, k) * (d^(3-k)/dx^(3-k)) x^2 * (d^k/dx^k) e^x
Calculating each term:
For k = 0: C(3, 0) * (d^3/dx^3) x^2 * e^x = 1 * 0 * e^x = 0
For-k = 1: C(3, 1) * (d^2/dx^2) x^2 * (d/dx) e^x = 3 * 2 * e^x = 6e^x
For k = 2: C(3, 2) * (d/dx) x^2 * (d^2/dx^2) e^x = 3 * 2x * e^x = 6xe^x
For-k = 3: C(3, 3) * x^2 * (d^3/dx^3) e^x = 1 * x^2 * e^x = x^2 e^x
Summing all terms: d^3/dx^3 [x^2 e^x] = 0 + 6e^x + 6xe^x + x^2 e^x
Most Common FAQs
The Leibniz Rule Calculator uses the Leibniz formula to compute the nth derivative of the product of two functions. By inputting the functions and the order of the derivative, the calculator automates the process and provides the result.
The Leibniz rule is crucial in calculus as it simplifies the process of finding higher-order derivatives of product functions. This is especially useful in fields requiring precise mathematical modeling and analysis.
Yes, the calculator is designed to handle a wide range of functions as long as they are properly defined and differentiable. This includes polynomial, exponential, trigonometric, and logarithmic functions, among others.