The Angle Multiplication Calculator is an invaluable tool for mathematicians, engineers, architects, and professionals involved in fields requiring precise rotational measurements. This calculator allows users to multiply an angle by a scalar, which is a fundamental operation in scaling geometric figures, adjusting navigational routes, and designing mechanical systems.
Formula of Angle Multiplication Calculator
The fundamental operation behind the Angle Multiplication Calculator is straightforward yet vital for numerous applications:
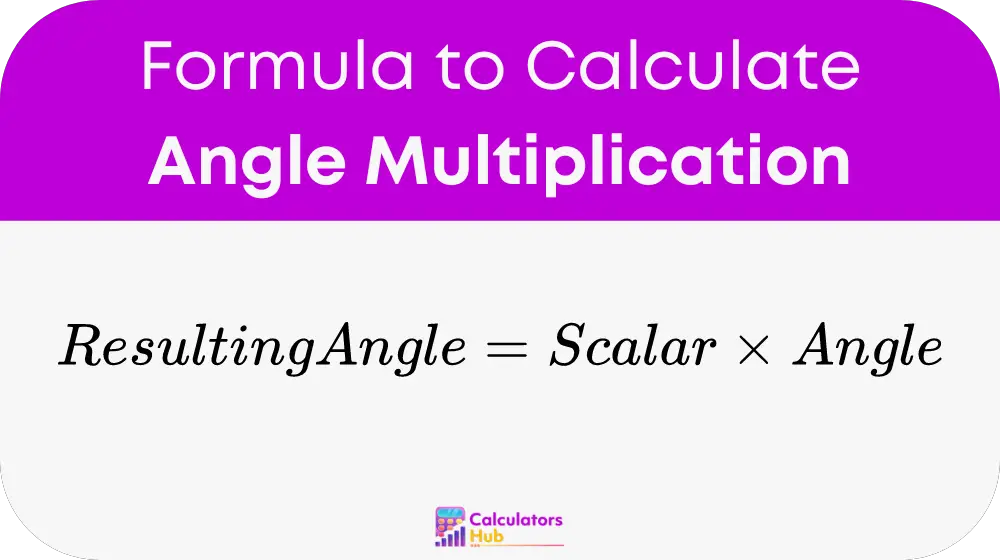
Where:
- Resulting Angle is the product of the multiplication.
- Scalar is the multiplier, a numerical value by which the angle is to be multiplied.
- Angle is the original angle, which can be inputted in degrees or radians.
Steps for Calculation:
- Identify the Scalar Value: Determine the number by which you want to multiply the angle.
- Identify the Angle: Input the original angle. Ensure it is in the correct unit (degrees or radians) for your calculation needs.
- Multiply the Scalar Value by the Angle to find the resulting angle.
Detailed Process:
- Convert the angle from degrees to radians if necessary:
- For degrees to radians: Angle in radians = Angle in degrees * (π / 180)
- For radians to degrees: Angle in degrees = Angle in radians * (180 / π)
- Multiply the scalar value by the angle:
- Resulting Angle = Scalar * Angle
Table of General Terms and Calculations
To enhance usability, a table of terms commonly associated with angle multiplication is provided:
| Term | Definition | Usage |
|---|---|---|
| Resulting Angle | The angle obtained after multiplication | Main output of the calculator |
| Scalar | The multiplier for the angle | Determines how much the angle is scaled |
| Angle | The initial angle before multiplication | Input that will be scaled |
| Conversion | The process of changing angle units | Necessary for accurate calculations |
This table acts as a quick reference guide, allowing users to understand and apply the calculator’s functions without extensive searches.
Example of Angle Multiplication Calculator
Suppose an architect needs to design a spiral staircase and must calculate the angle of rotation for each step if the staircase makes two complete 360-degree turns and has 24 steps:
- Scalar (number of turns): 2 turns * 360 degrees = 720 degrees
- Number of steps: 24
- Angle per step: Scalar / Number of steps = 720 / 24 = 30 degrees per step
This calculation shows that each step should rotate 30 degrees from the previous one to achieve the desired spiral design.
Most Common FAQs
Angle multiplication is crucial in fields like robotics (for joint movement calculations), architecture (for designing elements like staircases and windows), and navigation (for adjusting course directions).
Scalar multiplication scales the angle by a factor, increasing or decreasing its magnitude without altering its inherent properties. This is used to create proportional adjustments in design and motion.
Yes, depending on the context of the calculation. Some scientific and engineering fields prefer radians for theoretical work and calculations, while others, like construction, may use degrees for practical measurements.