The Beta Coefficient Calculator is a crucial tool for investors, financial analysts, and portfolio managers. It helps in measuring the volatility or systemic risk of an individual asset relative to the overall market. In the world of finance, understanding the risk associated with an investment is key to making informed decisions. The beta coefficient is a statistical measure that indicates how much an asset’s price is expected to move in relation to the market’s movement.
If a stock has a beta greater than 1, it means that the stock is more volatile than the market. Conversely, a beta of less than 1 indicates that the stock is less volatile. A beta of 1 means that the stock’s price will likely move with the market. This information is vital for those who are building a portfolio and need to balance risk versus return.
Beta Coefficient Calculation Formula
Beta(β) is calculated using the following formula:
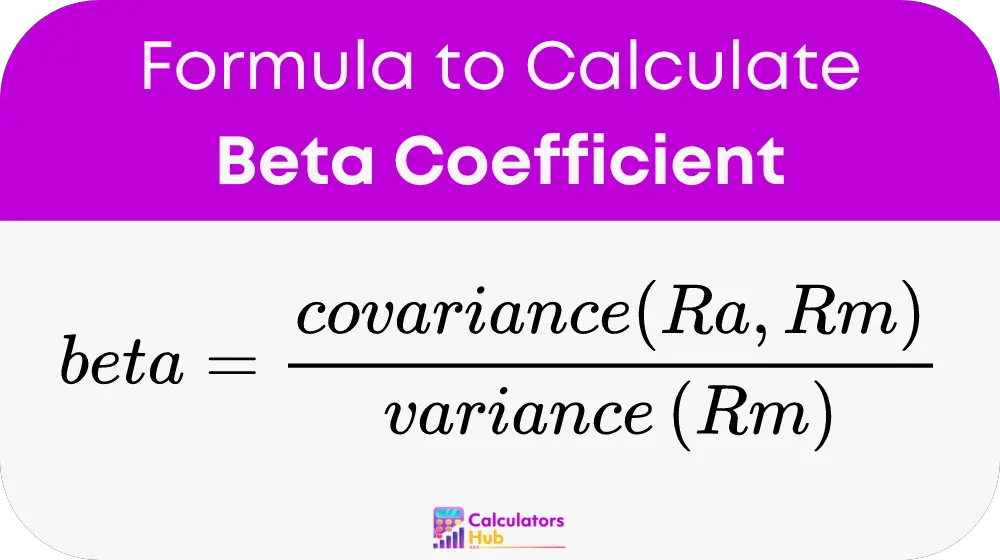
Where:
- Ra = Return of the asset
- Rm = Return of the market
- covariance(Ra, Rm) = Sum of the product of the differences of each return from their respective means, divided by the number of observations minus one
- variance(Rm) = Sum of the squared differences of each market return from the mean market return, divided by the number of observations minus one
This formula gives a quantitative measure of how an asset’s returns change in response to market changes. The result is used to understand and predict the asset’s risk level and its impact on the overall portfolio.
Common Terms and Conversion Table
To simplify the use of the Beta Coefficient Calculator, here is a table that defines key terms and provides some common conversions that are useful in financial calculations.
| Term | Definition |
|---|---|
| Beta (β) | A measure of an asset’s volatility relative to the market |
| Covariance | Measure of how two variables move together |
| Variance | Measure of the dispersion of returns for the market |
| Return of the Asset (Ra) | The gain or loss on an investment over a specified period |
| Return of the Market (Rm) | The gain or loss of the market index over a specified period |
| Standard Deviation | A measure of the amount of variation in a set of values |
| Correlation Coefficient | A statistical measure of the strength and direction of a relationship between two variables |
This table serves as a handy reference for anyone calculating beta coefficients, helping them to better understand the underlying financial concepts and ensuring accurate results.
Example of Beta Coefficient Calculator
Let’s work through a practical example of how to calculate the beta coefficient for a stock.
Assume you have the following data:
- The returns of the asset (Ra) over a period are: 5%, 7%, 10%, -2%, and 4%.
- The returns of the market (Rm) over the same period are: 3%, 6%, 9%, -1%, and 3%.
First, you would calculate the mean returns for both the asset and the market. Then, you calculate the covariance between the asset returns and the market returns, and the variance of the market returns. Finally, you would use the formula:
beta = covariance(Ra, Rm) / variance(Rm)
After performing the calculations, let’s assume the covariance is 0.0018 and the variance of the market is 0.0025. The beta would then be:
beta = 0.0018 / 0.0025 = 0.72
This beta value of 0.72 indicates that the asset is less volatile than the market, meaning it is likely to experience smaller price fluctuations compared to the overall market.
Most Common FAQs
Beta coefficient provides insight into the volatility of a stock compared to the market. A beta greater than 1 suggests that the stock is more volatile than the market, while a beta less than 1 suggests it is less volatile. A beta of 1 implies that the stock’s price movement is expected to mirror the market’s movements.
The beta coefficient is crucial in portfolio management because it helps in assessing the risk associated with a particular stock relative to the market. Investors use beta to determine how much risk a stock adds to a portfolio and to balance the portfolio according to their risk tolerance.
Beta affects investment decisions by providing a measure of the risk that an investor might take on by including a particular stock in their portfolio. Investors seeking higher returns might opt for stocks with higher betas, while risk-averse investors might prefer stocks with lower betas.