The Electrostatic Surface Potential Calculator helps determine the electric potential at a surface due to a charged interface within a surrounding ionic medium. This potential, often symbolized as ψ₀, plays a vital role in electrochemistry, surface science, and colloidal chemistry. It helps scientists and engineers understand surface interactions, double-layer formation, and stability in systems like biological membranes, nanoparticles, and charged surfaces in electrolyte solutions.
This calculator is especially valuable for researchers working with zeta potentials, Debye lengths, and charged colloidal systems. It translates complex mathematical modeling into an accessible and accurate estimation of electrostatic surface potential.
Formula of Electrostatic Surface Potential Calculator
The electrostatic surface potential is calculated using the following expression:
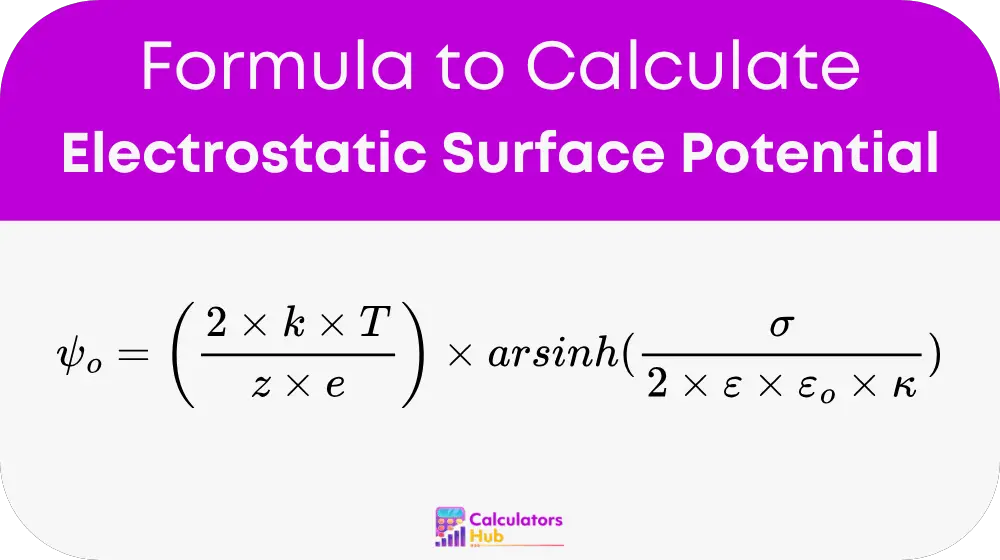
Where:
- ψ₀ is the electrostatic surface potential (in volts)
- k is the Boltzmann constant = 1.380649 × 10⁻²³ J/K
- T is the absolute temperature (in Kelvin)
- z is the valency of the ion (unitless)
- e is the elementary charge = 1.602 × 10⁻¹⁹ C
- σ is the surface charge density (in C/m²)
- ε is the relative permittivity (dielectric constant) of the medium
- ε₀ is the vacuum permittivity = 8.854 × 10⁻¹² F/m
- κ is the inverse Debye length (in m⁻¹), related to the ionic strength
- arsinh is the inverse hyperbolic sine function
This equation is derived from the Poisson-Boltzmann model for charged surfaces and provides a reliable way to estimate the surface potential from known surface and medium parameters.
General Terms Related to Surface Potential Calculations
Below is a table with commonly searched terms and helpful definitions for understanding and working with this calculator.
| Term | Definition |
|---|---|
| Electrostatic Surface Potential (ψ₀) | The electrical potential at a charged interface within a solution |
| Surface Charge Density (σ) | The charge per unit area on a surface, measured in C/m² |
| Dielectric Constant (ε) | A measure of a medium’s ability to reduce electric field strength |
| Debye Length (κ⁻¹) | The distance over which electric potential decays in an electrolyte |
| Boltzmann Constant (k) | Relates temperature to energy in physical systems |
| Valency (z) | The charge number of an ion (e.g., +1 for Na⁺, +2 for Ca²⁺) |
| Inverse Hyperbolic Sine (arsinh) | A mathematical function related to logarithmic expressions |
| Ionic Strength | A measure of the concentration of ions in a solution |
These terms are essential for interpreting results and understanding how input values influence surface potential outcomes.
Example of Electrostatic Surface Potential Calculator
Let’s calculate the electrostatic surface potential for a system under specific conditions.
Given:
- Temperature (T) = 298 K
- Surface charge density (σ) = 0.01 C/m²
- Relative permittivity (ε) = 80 (for water)
- Valency (z) = 1
- Inverse Debye length (κ) = 1 × 10⁹ m⁻¹
Step 1: Insert constants
- k = 1.380649 × 10⁻²³ J/K
- e = 1.602 × 10⁻¹⁹ C
- ε₀ = 8.854 × 10⁻¹² F/m
Step 2: Calculate the argument of arsinh = σ / (2 × ε × ε₀ × κ)
= 0.01 / (2 × 80 × 8.854 × 10⁻¹² × 1 × 10⁹)
≈ 0.01 / 1.41664 × 10⁻¹⁰ ≈ 70.58
Step 3: Calculate the full expression
ψ₀ = (2 × 1.380649 × 10⁻²³ × 298) / (1 × 1.602 × 10⁻¹⁹) × arsinh(70.58)
ψ₀ ≈ 0.0513 × ln(70.58 + 70.59) ≈ 0.254 V
So, the electrostatic surface potential in this example is approximately 0.254 volts.
Most Common FAQs
A high potential usually suggests a strongly charged surface or a highly concentrated ionic environment. It often leads to strong repulsion or attraction forces in colloidal systems.
The Debye length determines how quickly the potential decays away from the surface. Shorter Debye lengths (due to high ionic strength) lead to a smaller interaction distance and reduced surface potential.
Yes, especially for membrane surfaces, proteins, or nanoparticle surfaces in buffered solutions. Just ensure accurate input values based on your medium and temperature.