The Critical Distance Calculator determines the point at which the direct sound from a source is equal in level to the reverberant sound in a given space. This calculation is essential in acoustics, particularly for audio engineers, architects, and sound designers, to optimize speaker placement, room acoustics, and sound clarity.
Critical distance is a crucial factor in sound system design, ensuring that speech intelligibility and sound fidelity are maintained in various environments, such as auditoriums, concert halls, and recording studios.
Formula of Critical Distance Calculator
To calculate the critical distance, use the following formula:
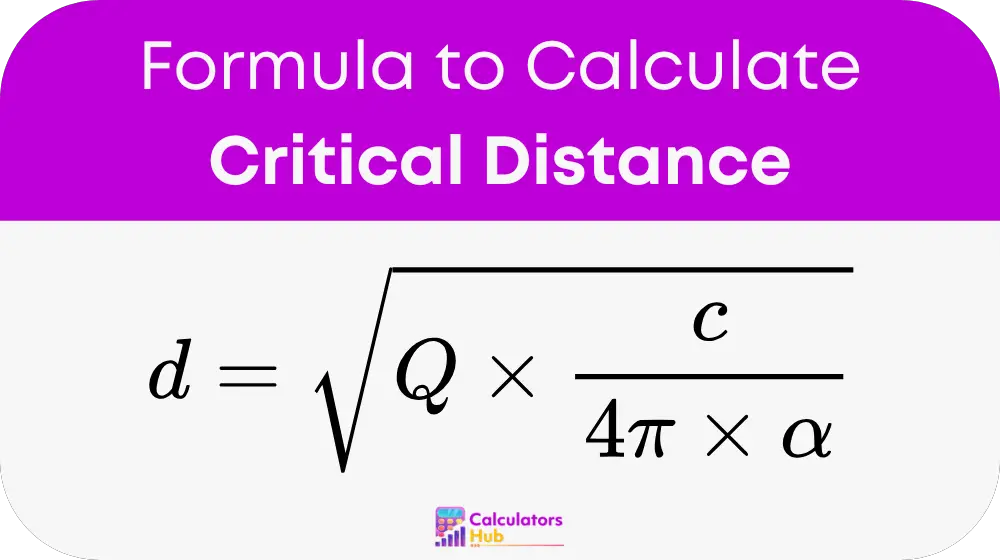
Where:
- d is the critical distance (in meters).
- Q is the directivity factor of the sound source (dimensionless).
- c is the speed of sound (approximately 343 m/s at 20°C in air).
- α is the absorption coefficient of the room (dimensionless).
This formula helps determine the optimal distance where the direct and reverberant sound levels are balanced.
General Terms Table
| Parameter | Description | Typical Values |
|---|---|---|
| Directivity Factor (Q) | Ratio of the directional sound power to an omnidirectional source | 1 (Omnidirectional), 2-6 (Directional) |
| Speed of Sound (c) | Speed at which sound travels in air | 343 m/s at 20°C |
| Absorption Coefficient (\alpha) | Represents how much sound is absorbed by surfaces | 0.1 (Concrete), 0.6 (Carpet), 0.9 (Acoustic Panels) |
This table provides a reference for commonly used values in critical distance calculations.
Example of Critical Distance Calculator
Let’s calculate the critical distance for a sound system with the following parameters:
- Directivity Factor (Q) = 4
- Speed of Sound (c) = 343 m/s
- Absorption Coefficient (α) = 0.3
Using the formula:
d = √(4 * 343 / (4 * π * 0.3))
d = √(364.22) ≈ 19.09 meters
This means the critical distance for the given parameters is approximately 19.09 meters.s.
Most Common FAQs
The critical distance helps in determining the best speaker placement for clear audio reproduction, minimizing reverberation issues and ensuring even sound distribution.
A higher absorption coefficient (\alpha) reduces reverberation, leading to a shorter critical distance, whereas a low absorption coefficient results in a larger critical distance.
Yes, critical distance varies with frequency since absorption coefficients and directivity factors change depending on the frequency of the sound.