In statistics and probability, understanding the frequency of one event occurring given that another has already occurred is essential. This is the purpose of the Conditional Relative Frequency Calculator. It provides an easy-to-use method for calculating the likelihood of an event B occurring, assuming event A has already happened. Such calculations play a crucial role in various fields, including finance, healthcare, and engineering, by offering insights for decision-making.
Formula of Conditional Relative Frequency Calculator
The formula for calculating conditional relative frequency, or P(B|A), quantifies the probability of event B happening given the occurrence of event A. It is given by:
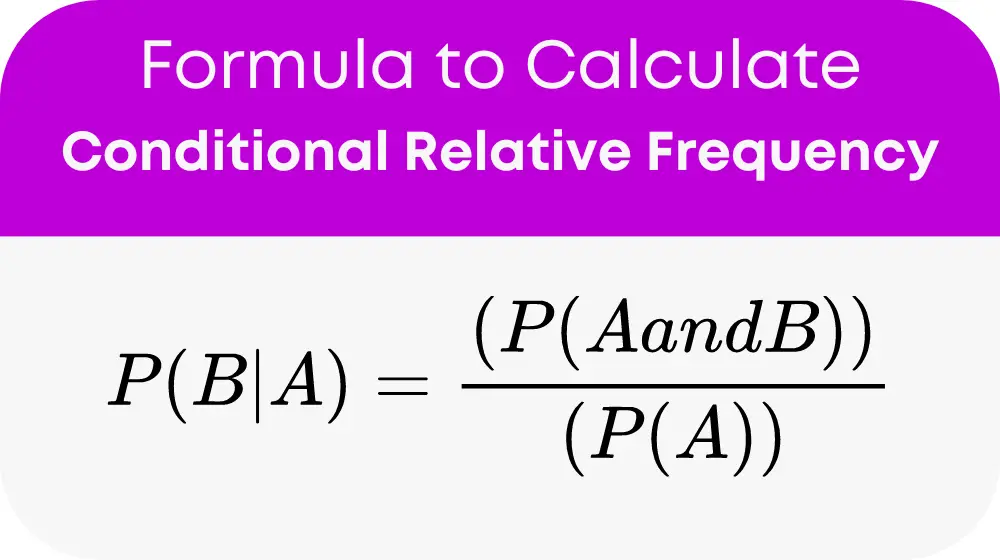
where:
- P(B|A) represents the conditional relative frequency of event B given event A.
- P(A and B) denotes the joint probability of events A and B occurring together.
- P(A) is the probability of event A happening.
This formula allows for the calculation of the conditional probability of event B, aiding in informed decision-making through statistical analysis.
General Terms Table
Below is a table of general terms often use with the Conditional Relative Frequency Calculator, aimed at aiding understanding and application:
| Term | Description |
|---|---|
| Conditional Relative Frequency | The likelihood of an event happening, given another event has already occurred. |
| Joint Probability | The probability of two events happening at the same time. |
| Probability | A measure of the likelihood of an event occurring. |
This table acts as a quick reference, equipping users with the knowledge needed to effectively use the calculator.
Example of Conditional Relative Frequency Calculator
In a healthcare scenario, let event A be the administration of a new medication, and event B be the recovery of patients. If the joint probability of both events (P(A and B)) is 0.15 and the probability of the medication being administered (P(A)) is 0.20, the conditional relative frequency is calculated as:
P(B|A) = 0.15 / 0.20 = 0.75
This result shows a 75% probability of recovery given the medication is administered, demonstrating the practical application of the calculator.
Most Common FAQs
It is a statistical measure that calculates the likelihood of an event occurring, given another event has already taken place, helping to understand the relationship between two events.
This calculator helps individuals and professionals make informed decisions by understanding the probability of certain outcomes based on specific conditions. It is particularly useful in sectors that rely on predictive modeling and risk assessment.
This concept finds application across various sectors such as finance for forecasting market trends, healthcare for predicting treatment outcomes, and engineering for estimating failure rates under certain conditions.