The Closed Pipe Resonance Calculator helps determine the resonant frequency of sound waves in a pipe that is closed at one end. This phenomenon is important in acoustics and physics, especially when designing musical instruments, pipes for ventilation systems, or other engineering applications where sound properties are critical. The calculator uses basic principles of wave propagation and harmonic frequencies to determine the pitch or tone produced by a closed pipe. Resonance occurs when the natural frequency of the pipe matches the frequency of sound waves, amplifying the sound.
Formula of Closed Pipe Resonance Calculator
The formula for calculating the resonant frequency (f) of a closed pipe is:
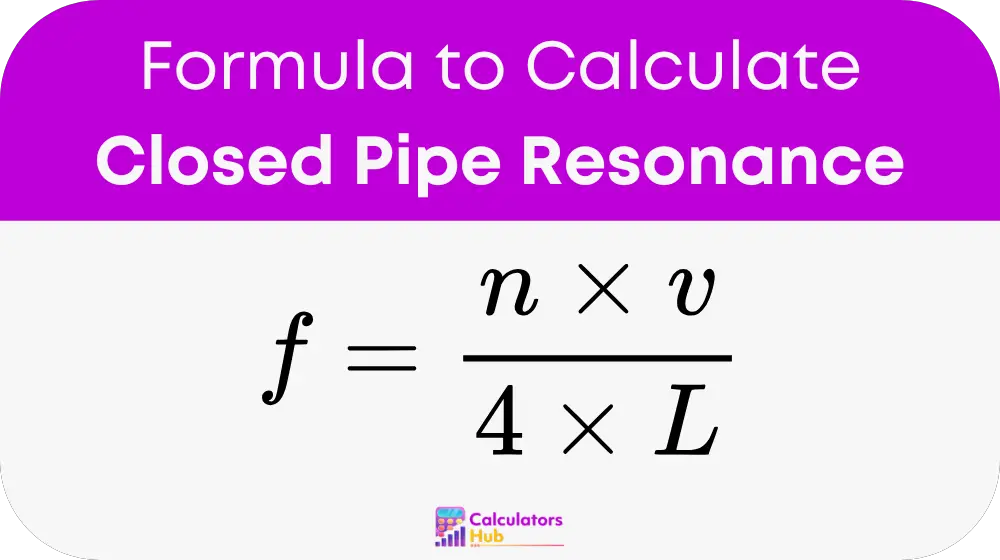
Where:
- f: Resonant frequency in Hertz (Hz)
- n: Harmonic number (1, 3, 5, 7, …)
- v: Speed of sound in the medium in meters per second (m/s)
- L: Length of the pipe in meters (m)
Understanding the Formula
- n (Harmonic Number): The harmonic number represents the multiples of the fundamental frequency. For a closed pipe, only odd harmonics (1, 3, 5, ...) are allowed.
- v (Speed of Sound): The speed of sound varies depending on the medium (air, water, etc.) and environmental conditions like temperature. For air at 20°C, the speed of sound is approximately 343 m/s.
- L (Length of the Pipe): The length of the pipe directly affects the resonant frequency. A longer pipe results in a lower frequency, while a shorter pipe yields a higher frequency.
Table for General Terms and Useful Conversions
Here is a table that provides some general values and terms you might need when using the Closed Pipe Resonance Calculator:
| Parameter | Value/Unit | Notes |
|---|---|---|
| Speed of Sound (v) | 343 m/s | For air at 20°C. Varies with temperature. |
| Harmonic Numbers (n) | 1, 3, 5, 7, ... | Odd harmonics only for a closed pipe. |
| Pipe Length (L) | 1 to 10 meters | Adjust based on the pipe's actual length. |
| Resonant Frequency (f) | Hz (Hertz) | The frequency produced by the closed pipe. |
These values are essential when calculating the resonance frequency, ensuring a quick understanding of how the pipe’s dimensions affect the sound produced.
Example of Closed Pipe Resonance Calculator
Scenario: You have a closed pipe with a length of 2 meters, and you want to find the first harmonic (fundamental frequency) of the pipe when the speed of sound is 343 m/s.
Using the formula:
f = (n * v) / (4 * L)
For the fundamental frequency (n = 1):
f = (1 * 343) / (4 * 2)
f = 343 / 8 = 42.875 Hz
Conclusion: The resonant frequency of a closed 2-meter pipe at the fundamental frequency is approximately 42.88 Hz. This is the primary frequency the pipe will emit when air is pushed through it.
Most Common FAQs
Resonance occurs when the natural frequency of vibration of the air column inside the closed pipe matches the frequency of the sound waves being produced. This amplifies the sound, making it much louder at specific frequencies, depending on the pipe's dimensions.
In a closed pipe, one end is closed while the other is open, which means the air at the closed end must remain stationary (a node), and the air at the open end vibrates freely (an antinode). This configuration only allows odd harmonics (1st, 3rd, 5th, etc.) to form, as the pipe cannot support even harmonics.
The length of the pipe plays a direct role in determining the resonant frequency. A longer pipe has a lower frequency, while a shorter pipe will have a higher frequency. This is because the sound waves need more space to travel and resonate within the pipe.