The Carry Distance Calculator is a useful tool primarily used in sports, physics, and engineering to determine how far an object, such as a golf ball, basketball, or projectile, will travel through the air before it lands. This calculator is essential for athletes and coaches who want to optimize their performance by understanding the dynamics of their shots or throws.
By inputting key variables like initial velocity, launch angle, and the acceleration due to gravity, users can accurately estimate the horizontal distance an object will carry. This information is valuable for making informed decisions during training and competitive scenarios. The calculator is not only applicable in sports but also in various fields, including aerodynamics, ballistics, and environmental science.
Formula of Carry Distance Calculator
The formula for calculating the carry distance is:
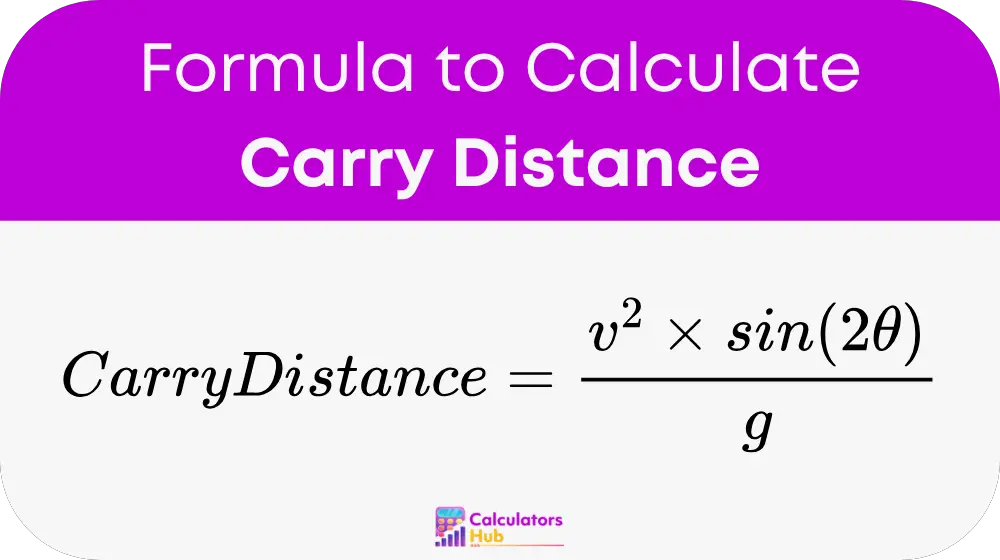
where:
- Carry Distance = Horizontal distance the object travels before landing (in meters or feet)
- v = Initial velocity (in meters per second or feet per second)
- θ = Launch angle with respect to the horizontal (in degrees)
- g = Acceleration due to gravity (9.81 m/s² or 32.17 ft/s²)
General Terms Table
The following table includes commonly searched terms related to carry distance, providing quick references to relevant terminology:
| Term | Definition |
|---|---|
| Initial Velocity | The speed at which an object is thrown or hit, measured in meters per second (m/s) or feet per second (ft/s). |
| Launch Angle | The angle at which an object is projected into the air, measured in degrees. |
| Acceleration Due to Gravity | The rate at which an object accelerates toward the Earth, approximately 9.81 m/s² or 32.17 ft/s². |
| Projectile Motion | The motion of an object that is thrown into the air, affected by gravity and air resistance. |
| Range | The total horizontal distance traveled by a projectile. |
| Trajectory | The path that a moving object follows through space as a function of time. |
| Ballistic Coefficient | A measure of a projectile's ability to overcome air resistance. |
Example of Carry Distance Calculator
To illustrate how to use the Carry Distance Calculator, consider the following scenario:
- Initial Velocity (v): 20 m/s
- Launch Angle (θ): 30 degrees
- First, calculate the sine of twice the launch angle:sin(2θ) = sin(60°) = √3 / 2 ≈ 0.866
- Now, apply the formula:Carry Distance = (20² × 0.866) / 9.81Carry Distance = (400 × 0.866) / 9.81Carry Distance ≈ 35.2 meters
In this example, the carry distance is approximately 35.2 meters, indicating how far the object will travel before landing when launched at an angle of 30 degrees with an initial velocity of 20 m/s.
Most Common FAQs
The launch angle significantly influences the carry distance. An optimal angle for most projectiles is around 30 to 45 degrees, as this range provides the best balance between vertical height and horizontal distance. Launching an object too steeply will result in a shorter carry distance, while a lower angle may increase air resistance and decrease distance.
Initial velocity is one of the most critical factors in calculating carry distance. A higher initial velocity means that the object will travel further before landing. This is why athletes focus on maximizing their speed when making throws or hits, as it directly correlates to how far the object will carry.
While the Carry Distance Calculator is commonly used in sports, its applications extend to various fields such as physics, engineering, and environmental science. It can be utilized in studying projectile motion, designing aerodynamics in vehicles, and even analyzing the environmental impact of airborne particles.