The Chord to Radius Calculator is a helpful tool used in geometry to find the radius of a circle when the length of the chord (C) and the height (H) of the arc are known. This is especially useful for architects, engineers, and designers who work with circular or curved structures. By entering these values, the calculator provides an accurate radius, eliminating the need for manual calculations. This tool falls under the category of geometry and measurement calculators. It ensures precision and reliability in solving problems related to circular geometry.
Formula of Chord To Radius Calculator
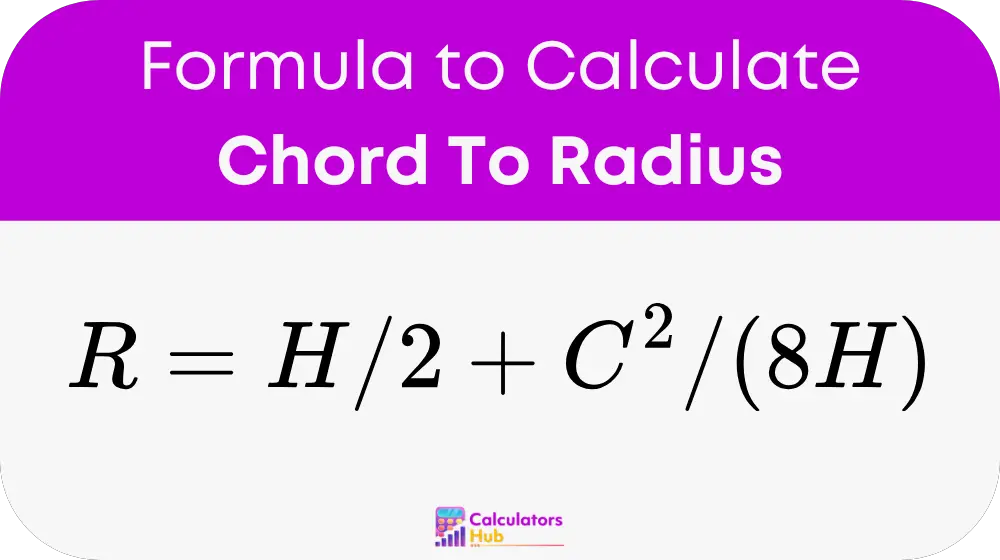
To calculate the radius (R) of a circle given the chord length (C) and the height (H) of the arc, use the formula:
Breakdown of the Formula:
- H/2: This term represents half the height of the arc.
- C²/(8H): This part comes from geometric properties of circles and chords, accounting for the relationship between the chord length and the arc height.
This formula is simple yet effective, allowing quick computation of the circle's radius without requiring advanced geometric knowledge.
Pre-Calculated Values Table
Here is a table of general values that can be used for reference without the need for recalculation. These values are often needed in practical situations when working with circular shapes:
| Chord Length (C) | Arc Height (H) | Radius (R) |
|---|---|---|
| 10 units | 3 units | 7.625 units |
| 20 units | 5 units | 13.125 units |
| 30 units | 8 units | 20.5 units |
This table can serve as a quick reference for common calculations involving circles, especially for designers and engineers who need to calculate radii quickly without performing each calculation individually.
Example of Chord To Radius Calculator
Let’s say you have a chord length of 12 units and an arc height of 4 units. To find the radius (R) of the circle:
R = 4/2 + 12²/(8×4)
R = 2 + 144/32 = 6.5 units
So, the radius of the circle is 6.5 units.
Most Common FAQs
The Chord to Radius Calculator helps you determine the radius of a circle when you know the length of the chord and the height of the arc. This is particularly useful in construction, engineering, and design fields when working with circular shapes.
The calculation is highly accurate as it is based on well-established geometric principles. The results you get from this calculator are precise, assuming correct values for chord length and arc height are input.
Yes, the Chord to Radius Calculator can be used for any circle size, as long as you know the values for the chord and the arc height. The formula is universal and applies to both small and large circles.