Curvature Calculator Vector Online

A curvature calculator is a crucial tool designed to calculate the curvature of a curve at any given point based on its vector representation. Understanding how sharply a curve bends, known as curvature, is essential for professionals in fields such as geometry and calculus, influencing designs and analyses.

Formula of Curvature Calculator Vector

The curvature of a curve defined by a vector function, r(t), is calculated using the following formula:

Curvature Formula:

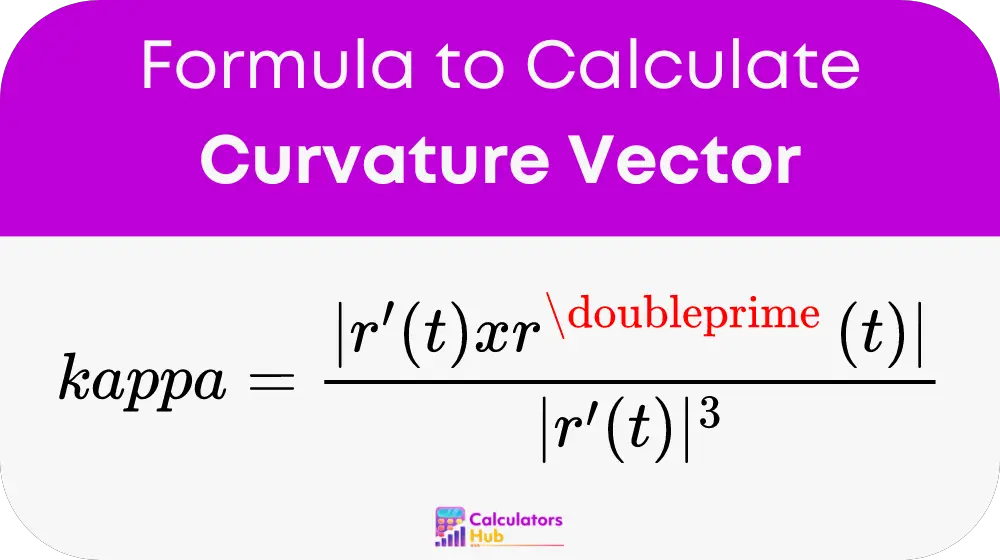
Definitions:

• r(t) represents the position vector of the curve as a function of parameter t,
• r'(t) is the first derivative of the position vector with respect to t,
• r”(t) is the second derivative of the position vector with respect to t,
• x denotes the cross product,
• |v| represents the magnitude of vector v.

Steps to Calculate Curvature:

1. Calculate the first derivative r'(t) by deriving each component of the position vector r(t).
2. Derive the second derivative r”(t) from each component of the first derivative r'(t).
3. Find the cross product of r'(t) and r”(t).
4. Calculate the magnitudes |r'(t)| and |r'(t) x r”(t)|.
5. Substitute the obtained values into the curvature formula to compute kappa.

This process allows the determination of the curvature of any smooth curve accurately.

Table of Common Curvature Values

Here is a table of typical curvature values for standard curves like circles of various radii and ellipses:

Curve Type	Equation	Curvature (kappa)
Circle (r=5)	x^2 + y^2 = 25	0.20
Ellipse	x^2/4 + y^2/9 = 1	Varies with t

Example of Curvature Calculator Vector

To illustrate, let’s calculate the curvature for the circle x^2 + y^2 = 25 using the provided formula:

1. Position vector r(t) = (5 cos(t), 5 sin(t)).
2. Deriving first and second derivatives: r'(t) = (-5 sin(t), 5 cos(t)), r”(t) = (-5 cos(t), -5 sin(t)).
3. Calculation of cross product and magnitudes.
4. Substitution into the curvature formula gives kappa = 0.20.

This example highlights the simplicity and effectiveness of using a curvature calculator for complex calculations.

Most Common FAQs