The Altitude Down Range Tangent Calculator is designed to compute the angle of descent or ascent, known as the tangent of the angle θ, for any given projectile or object in motion. This angle is crucial for determining the most efficient trajectory or for analyzing the path taken by a projectile. The calculator is particularly useful in applications involving ballistics, space flight trajectories, and sports physics.
Formula of Altitude Down Range Tangent Calculator
To determine the tangent of the descent or ascent angle θ, the following mathematical formula is used:
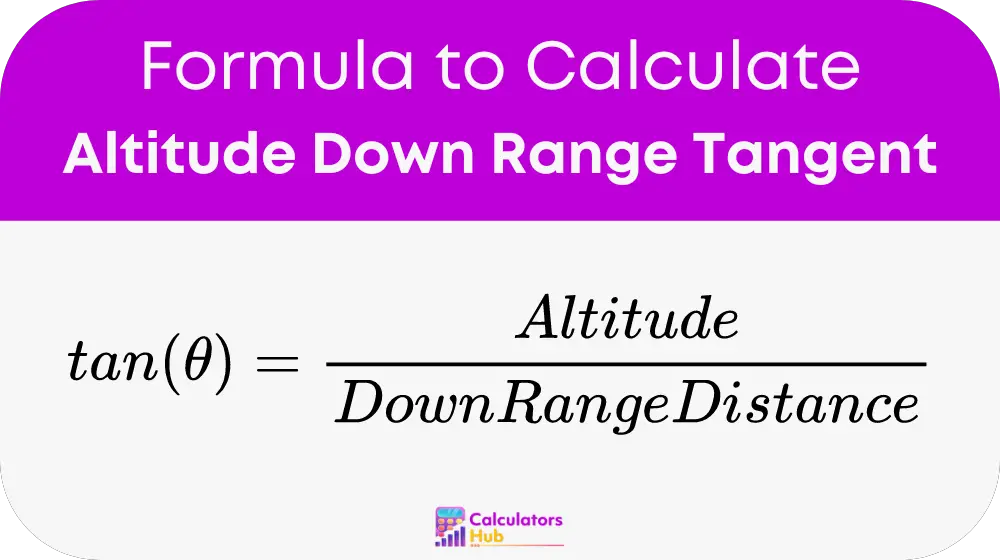
Where:
- θ is the angle of the projectile’s path relative to the horizontal.
- Altitude is the vertical distance from the launch point to the point of interest.
- Down Range Distance is the horizontal distance from the launch point to the point of interest.
To find the angle θ in degrees:
θ = arctan(Altitude / Down Range Distance)
Consistent units should be used for both Altitude and Down Range Distance to ensure accuracy.
Table for General Terms
| Term | Definition |
|---|---|
| θ (Theta) | The angle of the projectile’s path relative to the horizontal. |
| Altitude | Vertical distance from the launch point to the point of interest. |
| Down Range Distance | Horizontal distance from the launch point to the point of interest. |
| Tangent (tan) | The ratio of the opposite side to the adjacent side in a right-angled triangle. |
This table provides a quick reference for common terms associated with trajectory calculations, aiding users in understanding the key components without complex calculations.
Example of Altitude Down Range Tangent Calculator
For an example, suppose a projectile is launched from a height of 500 meters aiming at a target 1500 meters away horizontally. Using the calculator:
tan(θ) = 500 / 1500 = 0.3333
θ = arctan(0.3333) ≈ 18.43 degrees
This example demonstrates how to use the calculator to find the angle of ascent or descent, which can be crucial for adjusting firing angles or planning flight paths.
Most Common FAQs
A1: Calculating the altitude down range tangent is essential for understanding the trajectory of projectiles, which can influence the accuracy and efficiency of projectiles in military, sports, and aerospace applications.
A2: Yes, this calculator can be use for any projectile, provide that the altitude and down range distance are know, making it versatile across various fields.
A3: The accuracy of the calculator depends on the precision of the input values. It is highly accurate if precise measurements are use or the altitude and down range distances.