The Compressed Air Temperature Calculator is a tool designed to determine the final temperature of air after compression. This calculation is crucial for engineers and technicians working with compressed air systems, as it helps ensure system efficiency, safety, and proper equipment functioning. By using parameters such as initial temperature, pressure changes, and the adiabatic index, the calculator provides an accurate temperature value after compression.
Why Is It Important?
Understanding the temperature of compressed air is essential in designing and maintaining pneumatic systems, compressors, and pipelines. Excessively high temperatures can damage equipment, reduce efficiency, and pose safety risks. This calculator simplifies complex thermodynamic calculations, saving time and ensuring accuracy.
Formula of Compressed Air Temperature Calculator
The Compressed Air Temperature Calculator uses the following formula:
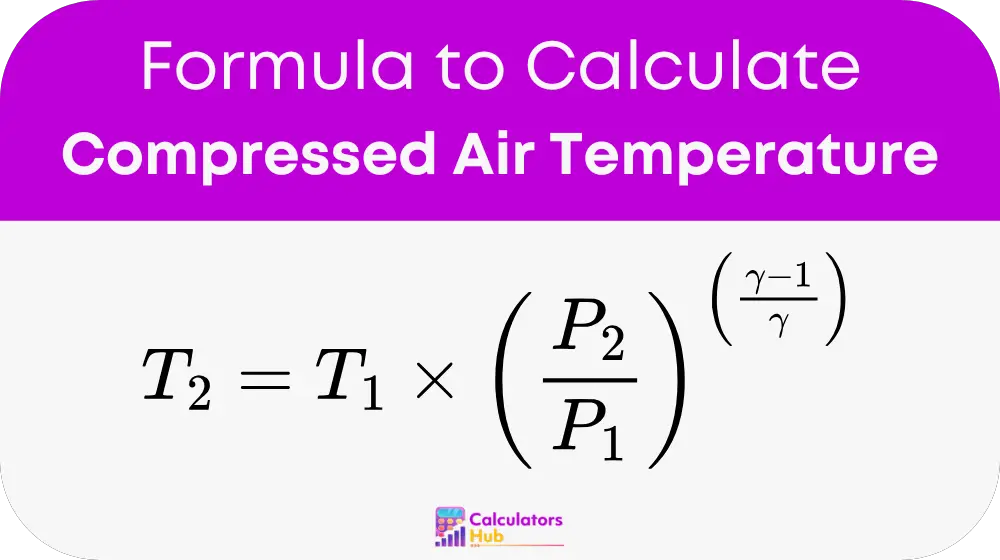
Variables
- T₂: Final temperature after compression (in Kelvin).
- T₁: Initial temperature before compression (in Kelvin).
- P₂: Final pressure after compression (absolute pressure).
- P₁: Initial pressure before compression (absolute pressure).
- γ: Adiabatic index (ratio of specific heats, Cₚ/Cᵥ).
Steps for Calculation
- Convert Temperatures to Kelvin:
If temperatures are provided in Celsius, convert them using:
T (Kelvin) = T (Celsius) + 273.15 - Determine Absolute Pressures:
If pressures are given as gauge pressures, convert them to absolute pressures using:
Absolute Pressure = Gauge Pressure + Atmospheric Pressure - Use the Adiabatic Index (γ):
For air, the adiabatic index is approximately 1.4. - Substitute Values into the Formula:
Use the formula T₂ = T₁ × (P₂ / P₁)^((γ - 1)/γ) to calculate the final temperature.
Pre-calculated Table for Common Scenarios
Below is a table showing the final temperature of compressed air for common scenarios:
| Initial Temp (°C) | Initial Pressure (P₁, bar) | Final Pressure (P₂, bar) | Final Temp (°C) |
|---|---|---|---|
| 25 | 1.0 | 5.0 | 194.6 |
| 30 | 1.0 | 8.0 | 320.3 |
| 40 | 1.0 | 10.0 | 425.5 |
| 50 | 1.0 | 6.0 | 272.1 |
This table helps users quickly estimate final temperatures for frequently encountered conditions.
Example of Compressed Air Temperature Calculator
Scenario
Calculate the final temperature of air compressed from 1 bar (absolute) to 5 bar (absolute) with an initial temperature of 25°C.
Step-by-Step Calculation
- Convert Initial Temperature to Kelvin:
T₁ = 25 + 273.15 = 298.15 K - Determine Absolute Pressures:
P₁ = 1 bar
P₂ = 5 bar - Use the Adiabatic Index:
γ = 1.4 - Apply the Formula:
T₂ = T₁ × (P₂ / P₁)^((γ - 1)/γ)
T₂ = 298.15 × (5 / 1)^((1.4 - 1)/1.4)
T₂ ≈ 298.15 × (5)^0.2857
T₂ ≈ 298.15 × 1.72 ≈ 512.8 K - Convert Final Temperature Back to Celsius:
T₂ (Celsius) = T₂ (Kelvin) − 273.15 ≈ 512.8 − 273.15 ≈ 239.65°C
Thus, the final temperature of the compressed air is approximately 239.65°C.
Most Common FAQs
The calculator helps determine the temperature of air after compression, ensuring system safety and efficiency in pneumatic and thermodynamic applications.
The adiabatic index (γ) reflects the heat properties of the gas and affects how temperature changes during compression. For air, γ is typically 1.4.
Gauge pressures must be converted to absolute pressures by adding atmospheric pressure (approximately 1 bar or 14.7 psi at sea level).