The RMS Speed Calculator computes the root mean square speed of gas molecules based on the given temperature and the mass of a single gas molecule. It simplifies the complex calculations involved in determining the average speed of gas molecules, providing accurate and quick results. This calculator is useful for students, researchers, and professionals who need to understand the dynamics of gases.
Formula of RMS Speed Calculator
The root mean square (RMS) speed of gas molecules can be calculated using the following formula:
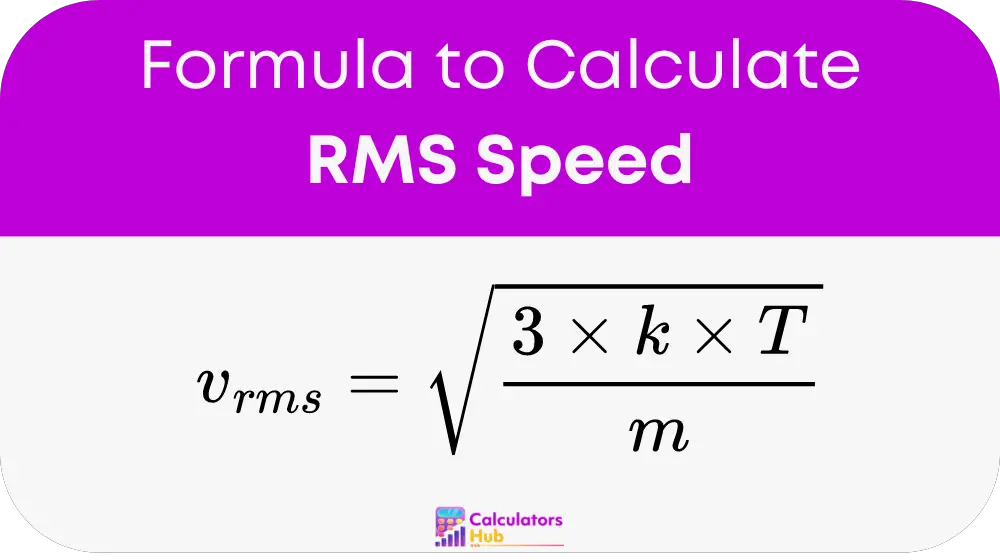
Where:
- v_rms is the root mean square speed
- k is the Boltzmann constant (1.38 × 10^-23 J/K)
- T is the temperature in Kelvin
- m is the mass of a single gas molecule
This formula is derived from the kinetic theory of gases, which states that the average kinetic energy of gas molecules is directly proportional to the temperature of the gas.
General Terms and Conversion Table
Below is a table of common terms and conversions that are useful when working with the RMS Speed Calculator:
| Term | Symbol | Value/Conversion |
|---|---|---|
| Boltzmann Constant | k | 1.38 × 10^-23 J/K |
| Temperature | T | Value in Kelvin (K) |
| Mass of Gas Molecule | m | Value in kilograms (kg) |
| RMS Speed | v_rms | √(3 * k * T / m) |
| Celsius to Kelvin | - | K = °C + 273.15 |
| Grams to Kilograms | - | 1 g = 1 × 10^-3 kg |
Example of RMS Speed Calculator
Let's calculate the RMS speed of nitrogen gas (N2) molecules at room temperature (25°C).
- Convert temperature to Kelvin: T = 25 + 273.15 = 298.15 K
- Mass of one N2 molecule: m = 4.65 × 10^-26 kg
- Using the RMS speed formula: v_rms = √(3 * 1.38 × 10^-23 * 298.15 / 4.65 × 10^-26)
- Calculate the value: v_rms ≈ 517 m/s
Therefore, the RMS speed of nitrogen gas molecules at room temperature is approximately 517 meters per second.
Most Common FAQs
The RMS speed gives an average speed of gas molecules, which helps in understanding the kinetic energy and behavior of gases under different temperatures.
As temperature increases, the kinetic energy of gas molecules increases, leading to a higher RMS speed. Conversely, a decrease in temperature results in a lower RMS speed.
Yes, the RMS Speed Calculator can be use for any gas. Provided the temperature and mass of the gas molecules are known.