The Atm to Moles Calculator utilizes the Ideal Gas Law to convert atmospheric pressure into the number of moles of a gas. This tool simplifies calculations for experiments and problems involving gas laws, ensuring precision in scenarios where understanding gas behavior under different conditions is crucial.
Formula of Atm To Moles Calculator
The formula used by the Atm to Moles Calculator is derived from the Ideal Gas Law:
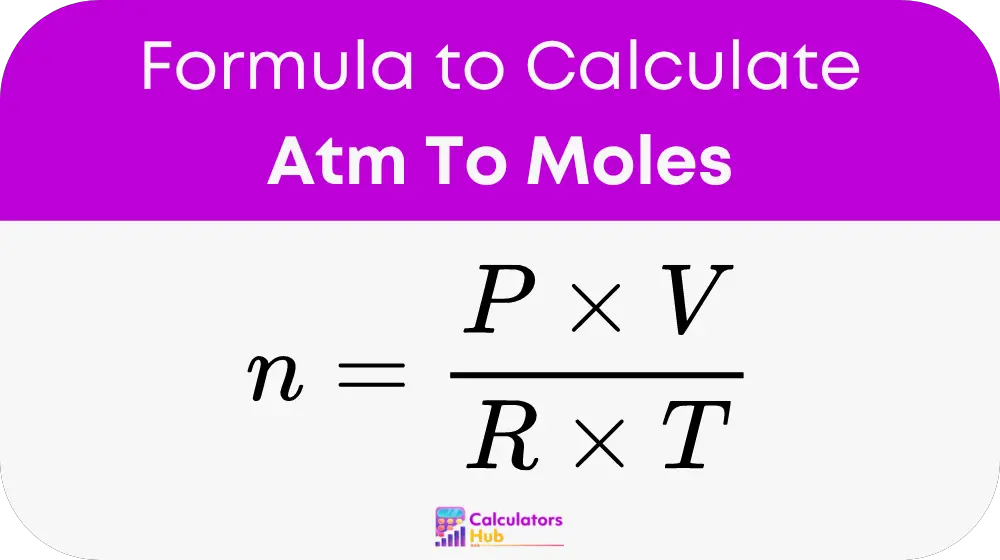
Where:
- n represents the number of moles of the gas.
- P is the pressure in atmospheres (ATM).
- V is the volume in liters (L).
- R is the ideal gas constant, which is approximately 0.0821 L·ATM/(mol·K).
- T is the temperature in Kelvin (K).
This rearrangement of the Ideal Gas Law (PV = nRT) allows for solving directly for the number of moles, facilitating quick computations in lab settings or theoretical exercises.
Table of General Terms
To aid in understanding, here’s a table defining key terms related to the Atm to Moles calculations:
| Term | Definition | Example Values |
|---|---|---|
| n | Number of moles of the gas | 2 moles, 5 moles |
| P | Pressure in atmospheres | 1 ATM, 2 ATM |
| V | Volume in liters | 22.4 L, 50 L |
| R | Ideal gas constant | 0.0821 L·ATM/(mol·K) |
| T | Temperature in Kelvin | 273 K, 298 K |
Example of Atm To Moles Calculator
Consider a scenario where you need to calculate the number of moles of a gas at 1 atmosphere of pressure, a volume of 22.4 liters, and a temperature of 273 Kelvin. Using the formula:
n = (1 ATM * 22.4 L) / (0.0821 L·ATM/(mol·K) * 273 K)
n ≈ 1 mole
This example demonstrates that under standard conditions (STP), 22.4 liters of an ideal gas at 1 atmosphere and 273 K contains approximately 1 mole of gas.
Most Common FAQs
A1: The Ideal Gas Law provides a foundational understanding of how gases behave under various conditions of pressure, volume, and temperature, crucial for fields such as chemistry, physics, and engineering.
A2: Yes, the Atm to Moles Calculator can adjust calculations based on different pressures, volumes, and temperatures. Making it a flexible tool for various scientific applications.
A3: The Ideal Gas Law assumes gases behave ideally, which is more accurate under high temperature and low pressure. It may not be as accurate for real gases under conditions of very high pressure and low temperature. Where intermolecular forces become significant.