The Air Density Calculator is an indispensable tool used by meteorologists, engineers, and scientists to determine the density of air under various atmospheric conditions. Air density, which indicates how closely packed the air molecules are, is crucial for applications ranging from aviation and weather forecasting to HVAC system design and environmental research. This calculator simplifies the process of calculating air density by considering atmospheric pressure, temperature, and the molecular composition of air.
Formula of Air Density Calculator
The formula to calculate air density is a fundamental expression derived from the ideal gas law, and it involves several key variables:
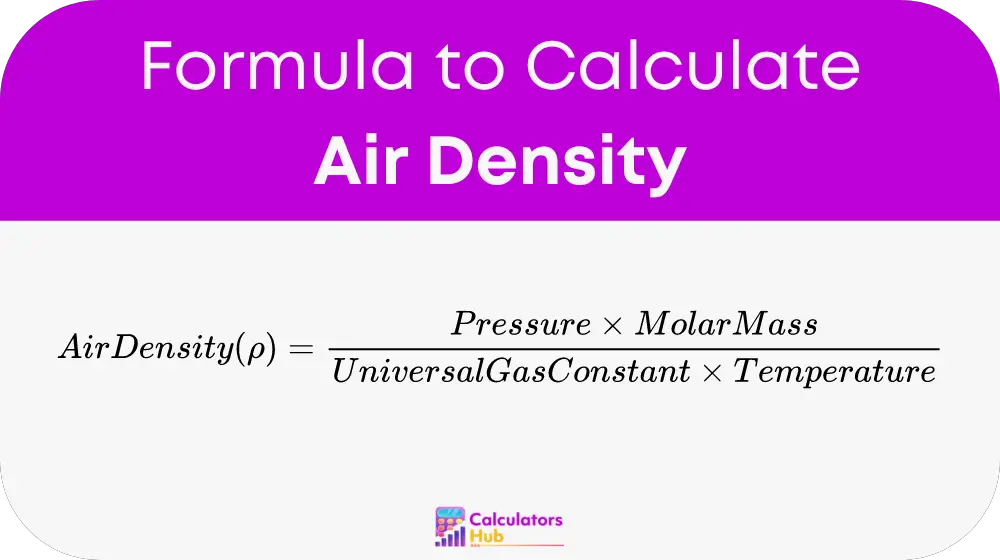
Where:
- Pressure (P): Measure the atmospheric pressure in Pascals (Pa).
- Molar Mass (M): Use the molar mass of dry air, which is approximately 0.029 kg/mol.
- Universal Gas Constant (R): Use the value 8.314 J/(mol·K).
- Temperature (T): Measure the temperature in Kelvin (K). To convert Celsius to Kelvin, add 273.15 to the Celsius temperature.
This formula allows for the calculation of air density by incorporating the physical constants of air and the prevailing atmospheric conditions.
General Terms Table
| Term | Definition |
|---|---|
| Air Density (ρ) | The mass per unit volume of air, typically in kilograms per cubic meter (kg/m³). |
| Pressure (P) | The force exerted by the atmosphere at a given point, measured in Pascals (Pa). |
| Molar Mass (M) | The mass of one mole of dry air, approximately 0.029 kg/mol. |
| Universal Gas Constant (R) | The constant in the ideal gas law, 8.314 J/(mol·K). |
| Temperature (T) | The absolute temperature measured in Kelvin (K). |
Example of Air Density Calculator
For instance, consider a scenario where the atmospheric pressure is 101,325 Pa (standard atmospheric pressure at sea level), the temperature is 15°C (converted to 288.15 K), and the molar mass of dry air is used:
- Air Density (ρ) = (101325 * 0.029) / (8.314 * 288.15) ≈ 1.225 kg/m³
This calculation results in the standard air density at sea level and 15°C, which is a common reference point in many scientific and engineering calculations.
Most Common FAQs
Air density affects wind patterns, cloud formation, and precipitation dynamics, making it crucial for accurate weather predictions.
Air density influences aircraft performance, including lift, engine output, and fuel efficiency. Pilots must consider air density to ensure safe flight operations.
Air density varies primarily with altitude, temperature, and humidity. Higher altitudes and temperatures generally decrease air density.