Strand Size: — m²
A Cable Strand Size Calculator is an essential tool for electrical engineers, contractors, and project managers involved in cable design and installation. This calculator determines the optimal size of individual strands within a cable, ensuring that the cable meets the necessary electrical and mechanical specifications. Properly calculating strand size is crucial for maintaining cable flexibility, strength, and electrical conductivity, which are vital for the safety and efficiency of electrical systems.
By using the Cable Strand Size Calculator, professionals can accurately select the number of strands and their diameters, preventing issues such as excessive heat generation, signal loss, and mechanical failure. This leads to reliable installations, reduced maintenance costs, and enhanced performance of electrical and communication systems.
Formula of Cable Strand Size Calculator
The formula used to calculate the cable strand size is:
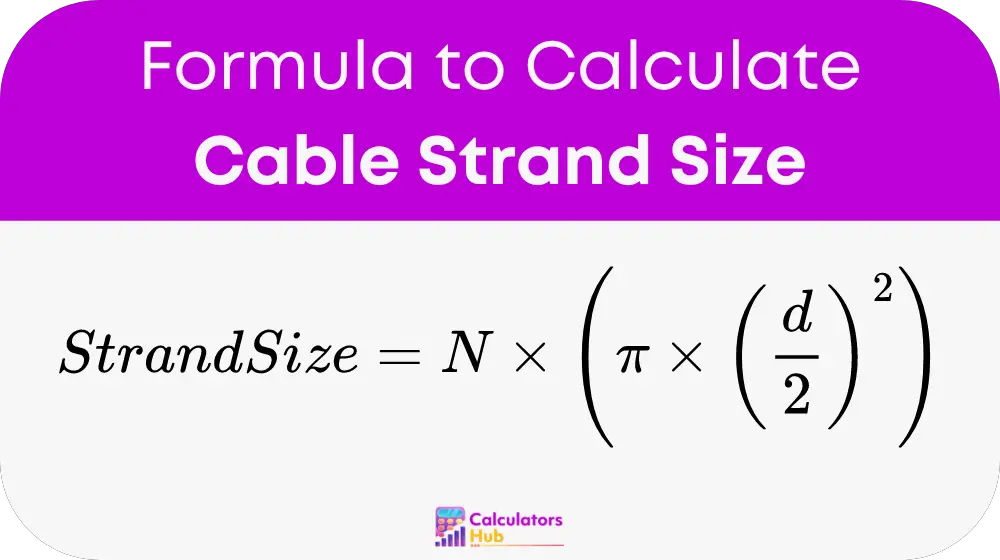
Where:
- N is the number of individual strands in the cable.
- d is the diameter of one strand in meters (m).
- π is the mathematical constant Pi (~3.14159).
Explanation of Terms
- Number of Strands (N): Represents how many individual wires make up the cable. More strands can provide greater flexibility and durability.
- Diameter of One Strand (d): The thickness of a single strand within the cable. Larger diameters can carry more current but may reduce flexibility.
- Pi (π): A constant approximately equal to 3.14159, used in calculating the area of a circle.
This formula calculates the total cross-sectional area of all strands combined, which is essential for ensuring that the cable can handle the required electrical load without overheating or causing voltage drops.
Helpful Table for Common Terms
The table below provides a quick reference for common strand sizes and their corresponding cable capacities, helping users estimate cable requirements without performing detailed calculations each time.
| Number of Strands (N) | Strand Diameter (mm) | Total Strand Area (mm²) | Suitable Applications |
|---|---|---|---|
| 7 | 1.0 | 5.50 | Light residential wiring |
| 19 | 0.8 | 4.78 | Communication and data cables |
| 37 | 0.6 | 3.95 | Flexible power cables |
| 61 | 0.5 | 3.14 | High-flex applications |
| 91 | 0.4 | 2.29 | Tight spaces and portable devices |
This table serves as a general guide for estimating the cable strand size based on the number of strands and their diameters. Users can adjust the values according to their specific requirements to obtain accurate results.
Example of Cable Strand Size Calculator
Let’s walk through an example to understand how the Cable Strand Size Calculator works.
Problem: You need to design a cable that requires a total strand area of 10 mm². You decide to use 19 strands in the cable. Calculate the diameter of each strand.
Solution:
Using the formula:
Strand Size = N × (π × (d / 2)²
First, rearrange the formula to solve for the diameter of one strand (d):
d = 2 × √(Strand Size / (N × π))
Plugging in the values:
- Strand Size = 10 mm²
- N = 19
- π ≈ 3.14159
Calculate the diameter:
d = 2 × √(10 / (19 × 3.14159))
d = 2 × √(0.1673) ≈ 0.818 meters
Since the diameter is typically measured in millimeters for practical applications:
d ≈ 0.818 mm
Thus, each strand should have a diameter of approximately 0.818 mm to achieve a total strand area of 10 mm² with 19 strands.
Most Common FAQs
The number of strands in a cable significantly influences its flexibility. More strands generally result in greater flexibility, making the cable easier to bend and handle. This is particularly important in applications where the cable needs to navigate through tight spaces or undergo frequent movement.
Yes, the Cable Strand Size Calculator is versatile and can be used for various types of cables, including electrical power cables, communication cables, and fiber optic cables. The key factor is the cable's application and the required electrical and mechanical properties.
Using the Cable Strand Size Calculator ensures that cables are designed with the appropriate number of strands and strand sizes. This enhances performance and longevity while preventing issues such as overheating and signal loss. It also aids in optimizing material usage and reducing costs.