The T-score Percentile Calculator is designed to convert T-scores into percentiles. A T-score indicates how far a score deviates from the mean in terms of standard deviations. The percentile tells you the percentage of scores in the reference group that fall below the given T-score. This calculator is essential for interpreting T-scores in a more understandable format, helping individuals and professionals make informed decisions based on standardized data.
Formula of T-score Percentile Calculator
The T-score Percentile Calculator uses the following formula to estimate the percentile from a T-score:
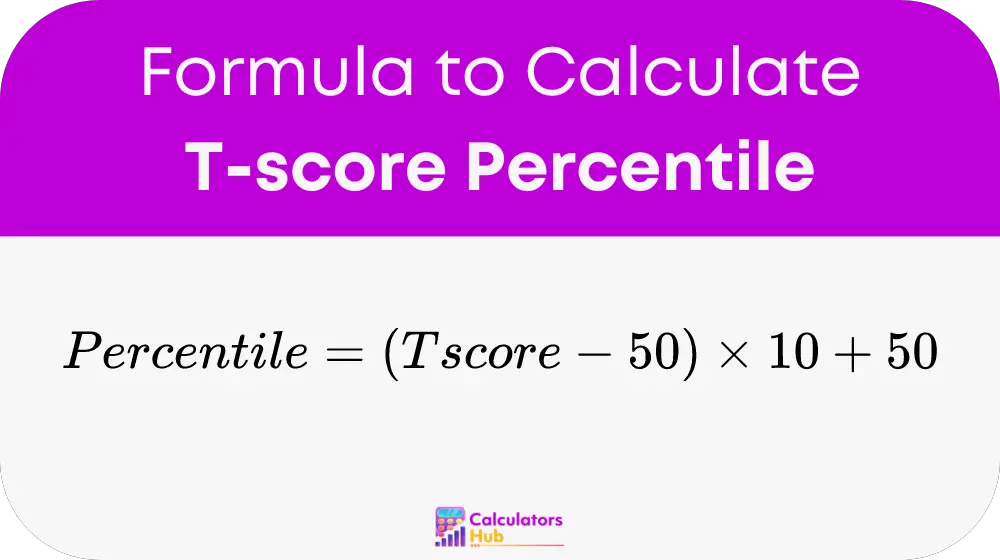
This formula assumes a normal distribution with a mean of 50 and a standard deviation of 10. While this formula provides an estimate, it’s most accurate for T-scores near the mean and less accurate for extreme values.
T-score to Percentile Conversion Table
Below is a table that provides a quick reference for converting T-scores to percentiles. This can be especially helpful for common T-scores without needing to calculate each time.
| T-score | Percentile |
|---|---|
| 20 | 5 |
| 30 | 15 |
| 40 | 25 |
| 50 | 50 |
| 60 | 75 |
| 70 | 85 |
| 80 | 95 |
This table simplifies the process of finding percentiles for common T-scores, allowing for quick and easy interpretation of results.
Example of T-score Percentile Calculator
Let’s go through an example to see how the Calculator works.
Step 1: Identify the T-score.
- Suppose we have a T-score of 60.
Step 2: Apply the formula.
- Percentile = (60 – 50) * 10 + 50
- Percentile = 10 * 10 + 50
- Percentile = 100 + 50
- Percentile = 150
Since percentiles cannot exceed 100, a T-score of 60 corresponds to the 99th percentile.
Step 3: Interpret the result.
- A T-score of 60 places an individual in the 99th percentile, meaning they scored higher than 99% of the reference group.
Most Common FAQs
A T-score is a standardized score that indicates how many standard deviations a data point is from the mean of a set of data.
It provides a clear understanding of how an individual score compares to a reference group, making it easier to interpret T-scores.
The calculator is most accurate for T-scores near the mean but provides a good estimate for all T-scores.