The Cumulative Distribution Calculator is a powerful tool used in statistics to determine the cumulative probability distribution of a random variable. It calculates the probability that the variable will be less than or equal to a certain value. This tool is especially handy in various fields such as finance, engineering, and data analysis, where understanding the distribution of data is crucial for decision-making.
Formula of Cumulative Distribution Calculator
Continuous Random Variables:
For continuous random variables, the Cumulative Distribution Function (CDF), denoted by F(x), is calculated by integrating the Probability Density Function (PDF) of the variable, f(x), from negative infinity to x.
Here's the formula:
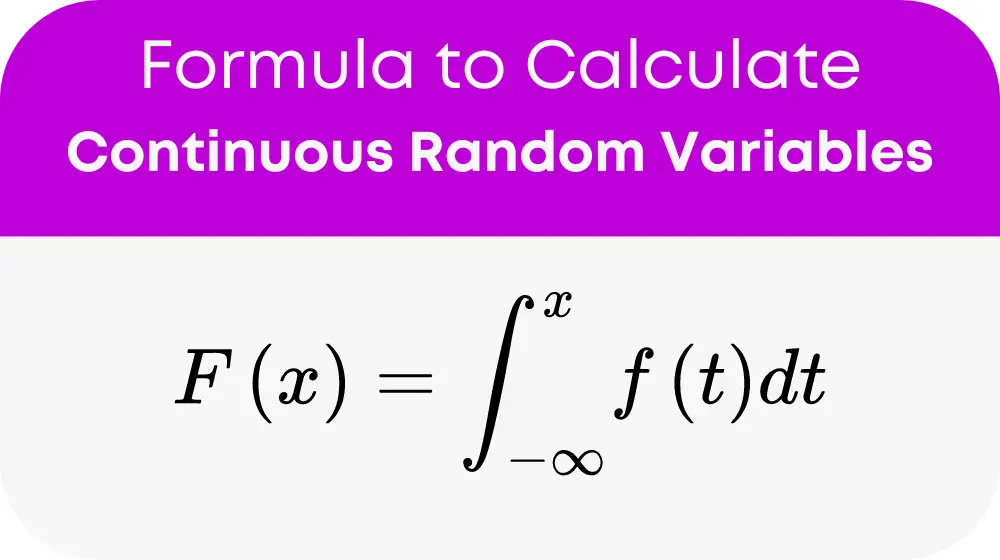
This essentially sums the probabilities of the variable taking on values less than or equal to x.
Discrete Random Variables:
For discrete random variables, the CDF is calculated by summing the probabilities of the variable taking on values less than or equal to x.
For each possible value (x_i) of the variable, you sum the probabilities (P(X = x_i)) associated with those values that are less than or equal to x.

Important Points:
- The CDF, F(x), represents the probability that the random variable X is less than or equal to x (F(x) = P(X ≤ x)).
- The CDF always starts at 0 (lim(x->-∞) F(x) = 0) and ends at 1 (lim(x->∞) F(x) = 1).
General Terms
| Random Variable Type | Example Distribution | Formula for CDF (F(x)) | Calculator Input |
|---|---|---|---|
| Continuous | Normal Distribution (describes heights, weights, etc.) | F(x) = Φ((x - μ) / σ) [Φ represents the standard normal CDF] | Input μ (mean), σ (standard deviation), and desired value (x). |
| Discrete | Binomial Distribution (models success/failure events) | F(x) = Σ (P(X = k) | k ≤ x) [X = number of successes, k = specific number of successes] |
Example of Cumulative Distribution Calculator
We are analyzing exam scores with a normal distribution (mean = 75, standard deviation = 10). We want to find the probability of scoring ≤ 80.
Solution: 1. Identify Parameters: - Mean (μ) = 75 - Standard Deviation (σ) = 10 - Target Score (x) = 80 2. Calculate Z-Score: Z = (80 - 75) / 10 = 0.5 3. Use the Cumulative Distribution Calculator: - Input Z = 0.5 and parameters into the calculator. - Obtain the cumulative probability (CDF) for Z = 0.5. 4. Interpret Results: - The CDF represents the probability of scoring ≤ 80. - This probability informs us about the likelihood of achieving a score below 80. Conclusion: The Cumulative Distribution Calculator facilitates precise probability assessments, aiding in informed decision-making based on statistical distributions.Most Common FAQs
The Cumulative Distribution Calculator is used to determine the probability that a random variable is less than or equal to a specified value. It helps in understanding the distribution of data and making statistical inferences.
The calculator operates by integrating or summing the probabilities of a random variable taking on values less than or equal to a given value, based on whether the variable is continuous or discrete.
Yes, the calculator can handle both discrete and continuous random variables, providing accurate cumulative distribution values for various statistical analyses.