–
A Crossover Sample Size Calculator helps researchers determine the number of participants needed for a crossover study. Crossover studies are commonly used in clinical trials, medical research, and experimental designs where the same participants receive multiple treatments over different periods.
Unlike parallel-group studies, crossover studies allow each participant to serve as their own control, reducing variability and increasing statistical power. Proper calculation of the sample size ensures that the study achieves reliable and statistically significant results.
This calculator is essential for researchers planning pharmaceutical trials, behavioral studies, and clinical interventions to determine the minimum number of subjects required for valid conclusions.
Formula of Crossover Sample Size Calculator
The required sample size for a crossover study is determined using the following formula:
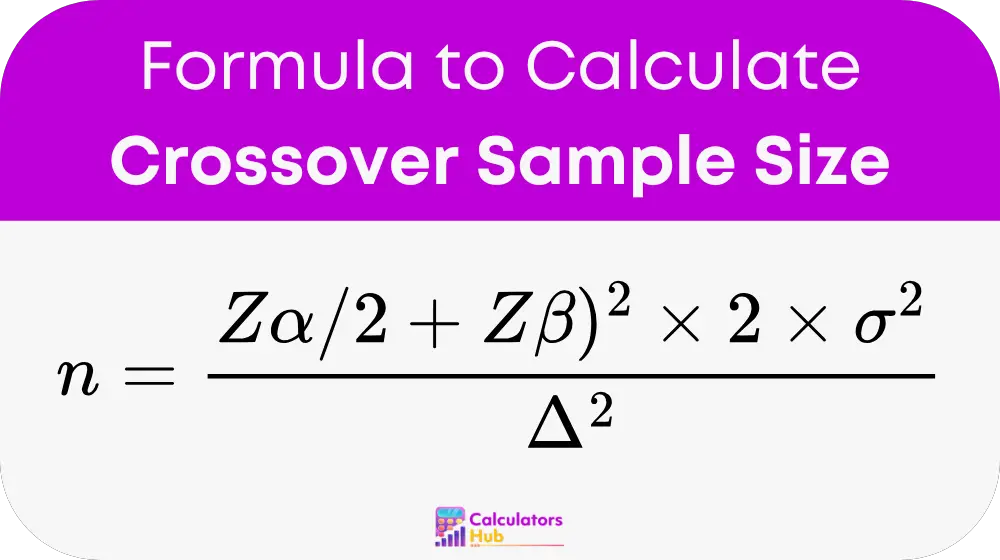
Where:
- n = required sample size per group
- Zα/2 = Z-value corresponding to the significance level (α), typically 1.96 for α = 0.05 (two-tailed test)
- Zβ = Z-value corresponding to the desired power, typically 0.84 for 80% power
- σ² = estimated variance of the outcome measure
- Δ = expected difference between treatments (effect size)
This formula ensures that the study includes enough participants to detect meaningful differences between treatments while maintaining statistical reliability.
Pre-Calculated Sample Size Estimates
The following table provides pre-calculated sample sizes for common study conditions, assuming a two-tailed test, 80% power, and different effect sizes:
| Effect Size (Δ) | Variance (σ²) | Required Sample Size (n) |
|---|---|---|
| 0.2 | 0.25 | 98 |
| 0.3 | 0.25 | 44 |
| 0.5 | 0.25 | 16 |
| 0.2 | 0.50 | 196 |
| 0.3 | 0.50 | 88 |
This table provides researchers with an estimate of sample size requirements based on typical effect sizes and variances.
Example of Crossover Sample Size Calculator
Let’s calculate the required sample size for a crossover clinical trial with the following parameters:
- Significance level (α) = 0.05 (two-tailed)
- Power = 80%
- Variance (σ²) = 0.4
- Expected effect size (Δ) = 0.3
- Find the Z-values:
- Zα/2 = 1.96
- Zβ = 0.84
- Apply the formula:n = ((1.96 + 0.84)² × 2 × 0.4) / (0.3²)
n = (7.84 × 0.8) / 0.09
n = 69.7 ≈ 70 participants per group
Thus, the study requires at least 70 participants per group to achieve statistical significance.
Most Common FAQs
Sample size calculation ensures that the study includes enough participants to detect significant differences between treatments, preventing underpowered or inconclusive results.
A larger effect size (Δ) requires fewer participants, while a smaller effect size increases the number of participants needed to detect statistical significance.
This calculator is specific to crossover study designs. For parallel-group trials, different sample size formulas are used.