The Critical Value Zc Calculator is a statistical tool used to determine the critical z-score for a given confidence level in hypothesis testing and confidence intervals. The critical value Zc helps identify the cut-off points in a normal distribution, beyond which values are considered statistically significant. It is widely used in statistics, research, quality control, and decision-making processes.
Formula of Critical Value Zc Calculator
The critical value Zc is calculate using the formula:
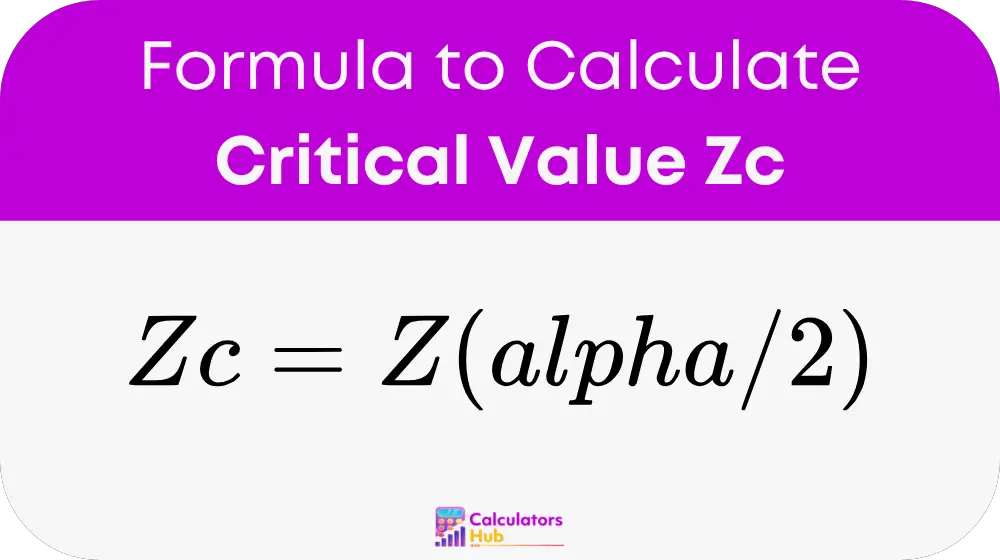
Where:
- Zc is the critical z-score.
- Alpha represents the significance level, calculated as 1 minus the confidence level.
- Z (alpha / 2) is the z-score corresponding to the given confidence level.
Common Critical Zc Values
- For a 90 percent confidence level: Zc is approximately 1.645
- For a 95 percent confidence level: Zc is approximately 1.96
- For a 99 percent confidence level: Zc is approximately 2.576
How to Calculate Critical Value Zc
- Determine the Confidence Level: Identify the confidence level needed (such as 90 percent, 95 percent, or 99 percent).
- Find the Significance Level: Calculate alpha as 1 minus the confidence level (for example, for 95 percent confidence, alpha = 1 – 0.95 = 0.05).
- Divide Alpha by 2: For a two-tailed test, divide alpha by 2 (for example, 0.05 / 2 = 0.025).
- Find the Corresponding Z-score: Use a statistical z-table or a calculator to find the z-score corresponding to 1 minus alpha divided by 2.
Z-Score Table for Common Confidence Levels
| Confidence Level | Alpha | Alpha / 2 | Critical Value Zc |
|---|---|---|---|
| 90 percent | 0.10 | 0.05 | 1.645 |
| 95 percent | 0.05 | 0.025 | 1.96 |
| 99 percent | 0.01 | 0.005 | 2.576 |
Example of Critical Value Zc Calculator
Suppose you are conducting a study and need to find the critical value Zc for a 95 percent confidence level.
- The confidence level is 95 percent.
- The significance level alpha is 1 – 0.95 = 0.05.
- Dividing alpha by 2 gives 0.025.
- Using a z-table, the z-score corresponding to 1 – 0.025 = 0.975 is 1.96.
Thus, the critical value Zc for a 95 percent confidence level is 1.96.
Most Common FAQs
A critical value Zc is the z-score that defines the threshold for statistical significance in hypothesis testing and confidence intervals.
Zc is use to determine the probability of a test statistic falling in the rejection region in hypothesis testing and to construct confidence intervals.
You can refer to a z-score table or use a statistical calculator to determine Zc based on the desired confidence level.