The Contingency Coefficient Calculator is a statistical tool used to measure the strength of association between two categorical variables. It is derived from a contingency table, which displays the frequency distribution of variables. The contingency coefficient helps researchers understand how strongly the variables are related, making it useful in fields such as social sciences, marketing, and healthcare research.
Formula of Contingency Coefficient Calculator
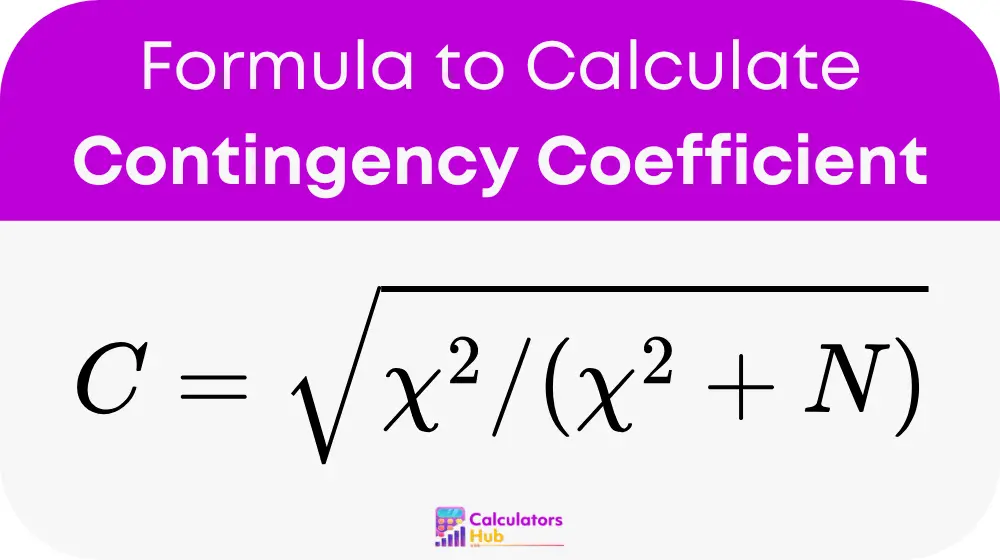
The contingency coefficient is calculated using the formula:
Detailed Formula Components
- χ² (Chi-Square Value):
- The chi-square statistic is calculated from the observed and expected frequencies in the contingency table:
χ² = Σ((Observed Frequency - Expected Frequency)² / Expected Frequency) - This value quantifies the difference between the observed and expected frequencies.
- The chi-square statistic is calculated from the observed and expected frequencies in the contingency table:
- N (Total Number of Observations):
- The total number of data points in the contingency table, representing the sum of all observed frequencies.
Characteristics of the Contingency Coefficient
- The value of C ranges between 0 and approximately 1.
- 0 indicates no association between the variables.
- Higher values indicate a stronger association.
- The maximum value of C depends on the size of the contingency table, meaning it is not standardized.
General Terms Table
Below is a table to provide quick reference values for common scenarios:
| χ² Value | Total Observations (N) | Contingency Coefficient (C) |
|---|---|---|
| 10 | 50 | √(10 / (10 + 50)) ≈ 0.408 |
| 25 | 100 | √(25 / (25 + 100)) ≈ 0.447 |
| 50 | 200 | √(50 / (50 + 200)) ≈ 0.447 |
| 75 | 250 | √(75 / (75 + 250)) ≈ 0.477 |
| 100 | 300 | √(100 / (100 + 300)) ≈ 0.5 |
This table demonstrates how the contingency coefficient changes with different chi-square values and total observations.
Example of Contingency Coefficient Calculator
Let’s calculate the contingency coefficient for a study where:
- χ² Value: 36
- Total Observations (N): 120
Calculation:
- Formula:
Contingency Coefficient (C) = √(χ² / (χ² + N)) - Substitute Values:
C = √(36 / (36 + 120))
C = √(36 / 156)
C = √0.2308
C ≈ 0.480.
Result:
The contingency coefficient is 0.480, indicating a moderate association between the two variables.
Most Common FAQs
The contingency coefficient measures the strength of association between two categorical variables. A higher value indicates a stronger relationship.
No, the contingency coefficient is not standardize, and its maximum value depends on the size of the contingency table. For comparison across studies, alternative measures like Cramér's V may be more appropriate.
The chi-square test evaluates whether there is an association between variables, while the contingency coefficient quantifies the strength of that association.