The Builder Notation Calculator is designed to interpret and compute sets defined by builder notation: { x | condition on x }. This tool simplifies the creation of sets by automating the computation process, allowing users to focus on analysis rather than manual calculation. It is particularly beneficial for students, educators, and professionals who regularly work with complex sets.
Formula of Builder Notation Calculator
Builder notation consists of several key components:
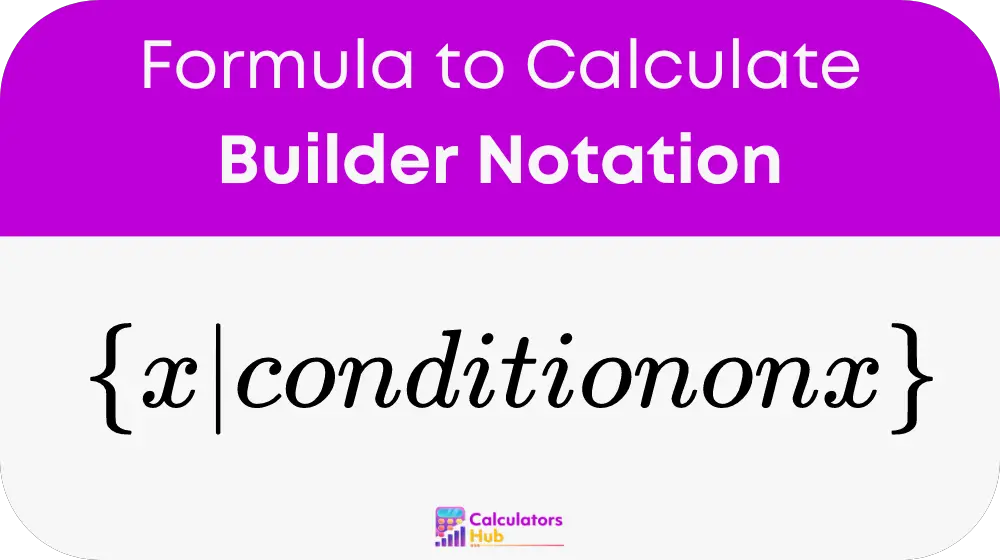
- Curly braces ({}) encapsulate the elements of the set.
- x represents each element within the set.
- Vertical bar (|), read as “such that”, separates the element from its defining condition.
- Condition specifies the criteria that elements must satisfy.
For instance, the set of all even numbers less than 10 is expressed as { x | x is even and x < 10 }, which results in the set { 2, 4, 6, 8 }.
Useful Tables and Conversion Tools
The following table includes common set notations and their meanings to assist users:
| Notation | Meaning |
|---|---|
| { x | x > 5 } |
| { x | x < 10 and x is prime } |
These tables and the calculator’s conversion tools help users apply builder notation without needing to perform each calculation manually.
Example of Builder Notation Calculator
To demonstrate the calculator’s capabilities, let’s solve for the set of prime numbers less than 10. By entering the condition “x < 10 and x is prime” into the calculator, it efficiently yields the set { 2, 3, 5, 7 }.
Most Common FAQs
Builder notation is a method to define sets with specific conditions.
It automates the calculation of sets defined in builder notation, ensuring accuracy and efficiency.
Yes, it is design to manage a wide range of mathematical sets with various complexities.
