The Boltzmann Ratio Calculator is a tool used to calculate the population ratio between two energy levels in a system, typically at thermal equilibrium. This calculation helps physicists and chemists understand how particles distribute themselves among different energy states in a system based on temperature. The Boltzmann ratio describes the likelihood that a particle occupies a higher energy state compared to a lower one, with the result depending on the temperature of the system and the energy difference between the states.
This is particularly useful in fields like quantum mechanics, statistical mechanics, and thermodynamics, where understanding the distribution of particles across energy levels is crucial for predicting the behavior of gases, radiation, and other systems.
Formula of Boltzmann Ratio Calculator
The Boltzmann ratio between two energy levels is calculated using the following formula:
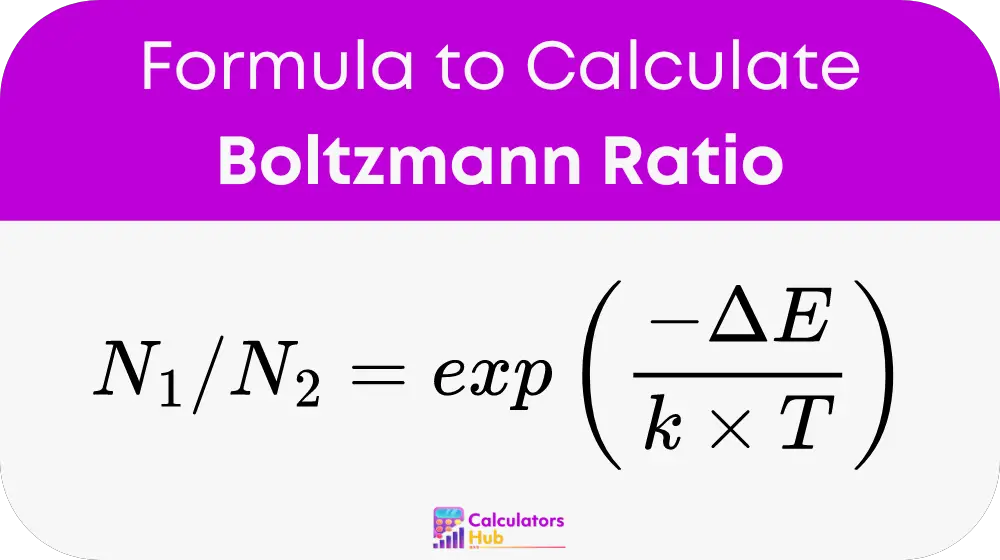
Where:
- N₂ is the population of particles in the higher energy state.
- N₁ is the population of particles in the lower energy state.
- ΔE is the energy difference between the two states (measured in Joules or electron volts).
- k is the Boltzmann constant (approximately 1.38 × 10⁻²³ J/K or 8.617 × 10⁻⁵ eV/K).
- T is the absolute temperature of the system (measured in Kelvins, K).
- exp(-x) represents the exponential function, which is e (Euler's number) raised to the power of -x.
Explanation of Key Terms:
- Energy Difference (ΔE): The amount of energy that separates the two states. Larger energy differences mean fewer particles will occupy the higher energy state at lower temperatures.
- Boltzmann Constant (k): A physical constant that relates temperature to energy in thermodynamic systems.
- Temperature (T): The absolute temperature of the system. Higher temperatures lead to a more even distribution of particles across different energy levels.
General Reference Table for Common Energy Levels and Temperatures
Here is a reference table showing the population ratios at various energy differences and temperatures. This table can help provide a quick estimate of how many particles are in the higher energy state relative to the lower energy state.
| Energy Difference (ΔE, eV) | Temperature (T, K) | Population Ratio (N₂/N₁) |
|---|---|---|
| 0.1 eV | 300 K | 0.0087 |
| 0.05 eV | 500 K | 0.224 |
| 0.01 eV | 1000 K | 0.728 |
| 0.005 eV | 2000 K | 0.871 |
| 0.001 eV | 5000 K | 0.981 |
This table provides a rough idea of how temperature and energy difference affect the population ratio between two states.
Example of Boltzmann Ratio Calculator
Let’s walk through an example of how the Boltzmann Ratio Calculator works.
Scenario:
You have a system with two energy states where the energy difference (ΔE) between the states is 0.1 eV, and the system is at a temperature of 300 K. You want to calculate the population ratio of particles in the higher energy state compared to the lower energy state.
- Step 1: Use the Boltzmann ratio formula: Population Ratio (N₂/N₁) = exp(-ΔE / (k * T))
- Step 2: Plug in the values:
- ΔE = 0.1 eVk = 8.617 × 10⁻⁵ eV/KT = 300 K
Population Ratio (N₂/N₁) ≈ exp(-3.87)
Ratio (N₂/N₁) ≈ 0.0209
So, the population ratio is approximately 0.0209, meaning that for every 100 particles in the lower energy state, about 2 are in the higher energy state.
Most Common FAQs
The Boltzmann ratio is essential for understanding how particles distribute themselves across energy levels in a system at thermal equilibrium. This information is crucial in fields like quantum mechanics, thermodynamics, and statistical mechanics, where energy distribution plays a significant role in system behavior.
Temperature has a significant effect on the population ratio. As temperature increases, more particles can occupy higher energy states, leading to a more even distribution between energy levels. At low temperatures, most particles will remain in the lower energy state.
The Boltzmann ratio is used in various applications, including:
Spectroscopy: To determine the relative populations of atomic or molecular energy levels.
Semiconductor physics: To study electron distributions in different energy bands.
Thermodynamic systems: To predict gas behavior or radiation distribution.