The Acceptance Sample Size Calculator is designed to determine the number of units that need to be sampled from a lot to make an informed decision about its acceptance or rejection. This tool is crucial in quality control processes, helping to ensure that the products meet the specified quality standards. By calculating the optimal sample size, companies can minimize the risk of accepting defective products or rejecting good ones.
Formula of Acceptance Sample Size Calculator
The formula to calculate the sample size n is:
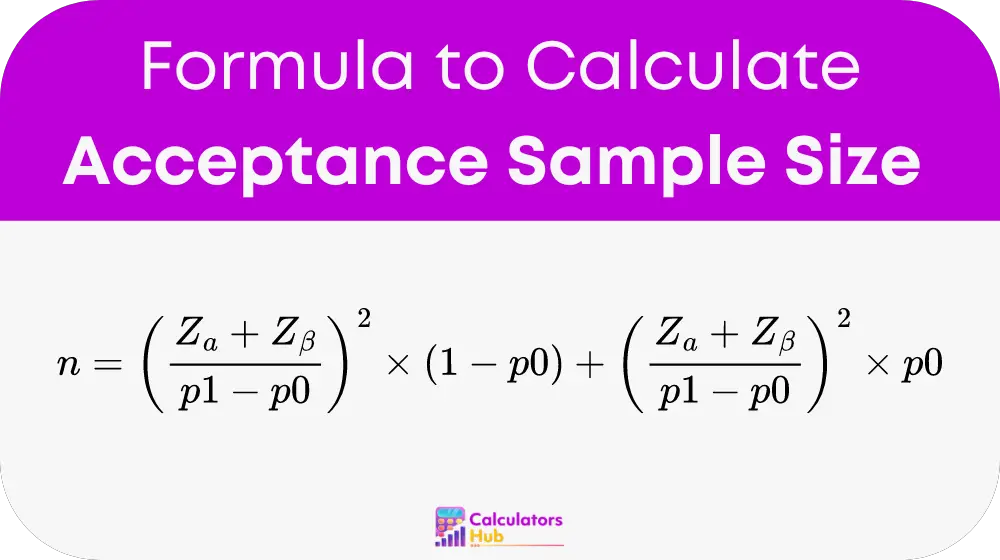
Where:
- n is the sample size
- Z_alpha is the Z-value corresponding to the desired confidence level (e.g., 1.96 for 95 percent confidence)
- Z_beta is the Z-value corresponding to the desired power (e.g., 0.84 for 80 percent power)
- p0 is the acceptable proportion of defects in the lot
- p1 is the proportion of defects considered unacceptable
General Terms and Reference Table
Common Terms
| Term | Definition |
|---|---|
| Sample Size | The number of units to be tested from the lot |
| Confidence Level | The probability that the sample accurately reflects the population |
| Power | The probability of correctly rejecting a defective lot |
| Acceptable Proportion (p0) | The maximum defect rate that is acceptable |
| Unacceptable Proportion (p1) | The defect rate that is considered unacceptable |
Pre-calculated Values
| Confidence Level | Power | Acceptable Proportion (p0) | Unacceptable Proportion (p1) | Sample Size |
|---|---|---|---|---|
| 95% | 80% | 0.01 | 0.05 | 258 |
| 95% | 90% | 0.01 | 0.05 | 323 |
| 99% | 80% | 0.01 | 0.05 | 333 |
| 99% | 90% | 0.01 | 0.05 | 419 |
Example of Acceptance Sample Size Calculator
Let's consider an example to illustrate the use of the Acceptance Sample Size Calculator.
Suppose a company wants to test a batch of products. The acceptable proportion of defects is 1 percent (p0), and the unacceptable proportion is 5 percent (p1). They desire a 95 percent confidence level (Z_alpha = 1.96) and an 80 percent power (Z_beta = 0.84).
Using the formula:
n = [(1.96 + 0.84) / (0.05 - 0.01)]^2 * (1 - 0.01) + [(1.96 + 0.84) / (0.05 - 0.01)]^2 * 0.01
n = (70)^2 * 0.99 + (70)^2 * 0.01 = 4851 + 49 = 4900
So, the sample size needed is 4900 units.
Most Common FAQs
The calculator helps determine the appropriate number of samples to be tested to make an informed decision about accepting or rejecting a lot.
The sample size is determine using a formula that considers the desired confidence level, power, acceptable proportion of defects, and unacceptable proportion of defects.
Using this calculator ensures that decisions about product quality are based on statistical evidence, minimizing the risk of accepting defective products or rejecting good ones.