The Absolute Ratio Test Calculator helps you find out if an infinite series converges or diverges. It does this by calculating the limit of the absolute value of the ratio of consecutive terms in the series. This saves you time and effort, especially when dealing with complex series.
Formula of Absolute Ratio Test Calculator
The Absolute Ratio Test is used to determine if an infinite series converges or diverges. For a series of the form:
sum from n=1 to infinity of a_n
The formula for the Absolute Ratio Test is:
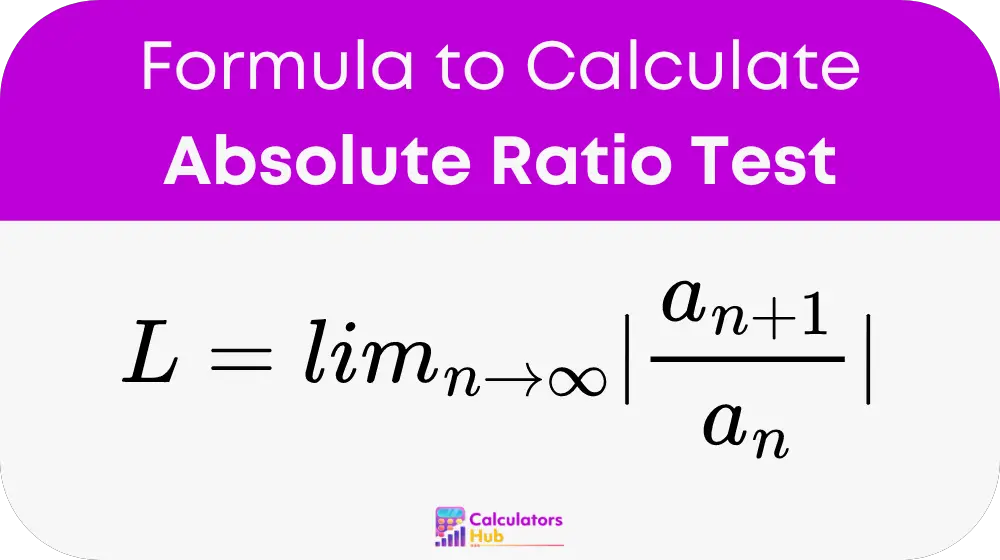
Where:
- a_n is the nth term of the series.
- |a_(n+1) / a_n| is the absolute value of the ratio of consecutive terms.
Interpretation of the Result:
- If L < 1, the series converges absolutely.
- If L > 1, the series diverges.
- If L = 1, the test is inconclusive (the series may converge or diverge).
This test helps to understand the behavior of the series by comparing the ratio of successive terms as n approaches infinity.
Commonly Used Terms Table
| Term (a_n) | Common Value |
|---|---|
| 1/n | 0.5 |
| 1/n^2 | 0.25 |
| 1/2^n | 0.125 |
This table provides common values for different terms, helping you quickly understand the behavior of the series without manual calculations.
Example of Absolute Ratio Test Calculator
Let’s consider the series sum from n=1 to infinity of 1/n^2.
- Identify the terms: a_n = 1/n^2.
- Calculate the ratio: |a_(n+1) / a_n| = |(1/(n+1)^2) / (1/n^2)| = |n^2 / (n+1)^2|.
- Find the limit: L = limit as n approaches infinity of |n^2 / (n+1)^2| = 1.
Since L = 1, the test is inconclusive for this series. Further tests would be needed to determine convergence or divergence.
Most Common FAQs
The Absolute Ratio Test is use to determine if an infinite series converges or diverges by examining the ratio of consecutive terms.
No, the Absolute Ratio Test is not conclusive for all series. If the limit is equal to 1, the test is inconclusive, and other tests may be needed.
The calculator automates the process of finding the limit of the ratio of consecutive terms, saving time and reducing the possibility of errors.