The Second Moment of Inertia quantifies the distribution of cross-sectional area of a beam around its bending axis, which directly influences the beam’s ability to resist bending or buckling under pressure. The I Beam Calculator simplifies these calculations, facilitating quick and accurate design decisions critical in engineering.
Formula of Second Moment of Inertia I Beam Calculator
Understanding the Formula: The formula to calculate the Second Moment of Inertia for an I Beam is given by:
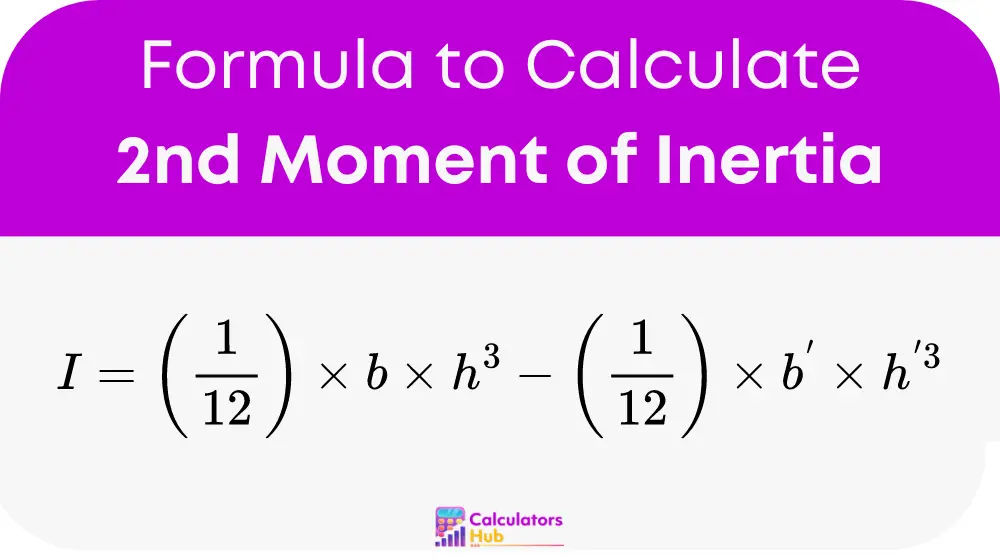
Where:
- I is the Second Moment of Inertia,
- b is the width of the flange,
- h is the height of the entire beam,
- b’ is the width of the web,
- h’ is the height of the web.
This formula helps predict how resistant a beam is to bending and is crucial for ensuring that structures are safe and efficient.
Pre-calculated Table for Common Beam Sizes
For convenience, below is a table with pre-calculated values of the Second Moment of Inertia for standard I Beam sizes:
| b (in) | h (in) | b’ (in) | h’ (in) | I (in^4) |
|---|---|---|---|---|
| 6 | 12 | 4 | 11 | 250 |
| 8 | 15 | 6 | 14 | 520 |
| 10 | 20 | 6 | 19 | 1250 |
This table serves as a quick reference to facilitate initial design and analysis phases, providing immediate access to necessary values.
Example of Second Moment of Inertia I Beam Calculator
Calculation Example: Let’s calculate the Second Moment of Inertia for an I Beam with the following dimensions:
- b = 8 in, h = 15 in, b’ = 6 in, h’ = 14 in.
Using the formula:
I = (1/12) * 8 * 15^3 – (1/12) * 6 * 14^3 I = 520 in^4
This example highlights the application of the formula and helps visualize how the dimensions of the beam impact its structural integrity.
Most Common FAQs
The Second Moment of Inertia measures the beam’s ability to resist bending. It is crucial for structural engineers to ensure beams are designed to handle specified loads without failing.
To calculate, use the specified formula and input the dimensions of the beam’s flange and web. The formula’s parameters must accurately represent the beam’s profile for correct results.
Yes, the calculator is versatile but check the dimension ranges for which it is calibrated to avoid errors in calculations for atypical beam sizes.