The Dual Spring Rate Calculator finds the combined spring rate when two springs work together in series. In series means one spring stacks after the other, like in some suspension systems. This calculator tells you how stiff the setup feels as a whole. It’s great for real-life tasks, like improving ride comfort, adjusting vehicle handling, or ensuring safety in mechanical designs.
You just need the spring rates of both springs, and the calculator gives you one number to work with. This helps you make smart decisions, like choosing the right springs for your project. Ready to see how it works? Let’s look at the formula next.
Formula for Dual Spring Rate
Calculating the combined spring rate for two springs in series is easy with this formula:
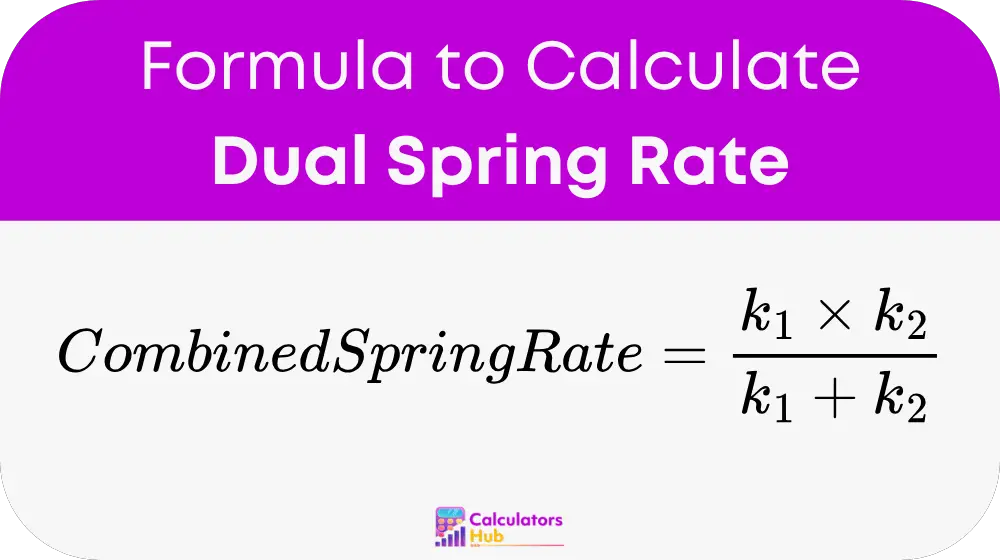
Where:
- Combined Spring Rate is the effective spring rate (in lbs/in or N/mm)
- k₁ is the spring rate of the first spring (lbs/in or N/mm)
- k₂ is the spring rate of the second spring (lbs/in or N/mm)
This formula comes from physics and shows how two springs share a load. The result is always softer than the weakest spring alone. Use pounds per inch (lbs/in) for U.S. units or newtons per millimeter (N/mm) for metric. Now, let’s simplify it with a table.
Quick Reference Table for Dual Spring Rates
Why calculate every time? This table shows common spring rate combos and their combined rates. It’s a fast way to plan without doing math each time.
| k₁ (lbs/in) | k₂ (lbs/in) | Combined Spring Rate (lbs/in) |
|---|---|---|
| 100 | 100 | 50 |
| 200 | 300 | 120 |
| 150 | 250 | 93.75 |
| 400 | 600 | 240 |
How to Use the Table
- Find the rates of your two springs.
- Check the combined rate in the table.
- Use it to plan your setup.
This table helps with searches like “dual spring rate for 200 and 300 lbs/in.” For other values, use the formula. Next, let’s try an example.
Example of Dual Spring Rate Calculator
Imagine you have two springs for a car suspension. The first spring has a rate of 150 lbs/in (k₁), and the second has a rate of 300 lbs/in (k₂). You want to know the combined spring rate. Here’s how to do it:
- Plug into the formula:
Combined Spring Rate = (k₁ × k₂) ÷ (k₁ + k₂) - Multiply the rates:
150 × 300 = 45,000 - Add the rates:
150 + 300 = 450 - Divide:
45,000 ÷ 450 = 100
So, the combined spring rate is 100 lbs/in. This matches engineering rules and shows the setup is softer than either spring alone.
Most Common FAQs
In series means one spring connects end-to-end with another, sharing the load and stretching together.
No, this formula is for series only. Parallel springs (side by side) add rates directly: k₁ + k₂.
In series, the springs share the work, making the setup feel softer than either one by itself.