A Direct Normal Radiation (DNI) Calculator helps measure the amount of solar radiation received per unit area on a surface that is always perpendicular to the sun’s rays. This is a critical metric for solar energy applications, including the design and efficiency analysis of concentrated solar power (CSP) systems and photovoltaic tracking systems.
Direct Normal Irradiance (DNI) is essential for understanding the solar potential of a location, allowing businesses and researchers to optimize solar panel installations. Since DNI is affected by atmospheric conditions, its calculation involves factors like air mass, atmospheric transmittance, and solar altitude angle.
Formula of Direct Normal Radiation Calculator
The formula to calculate Direct Normal Irradiance (DNI) is:
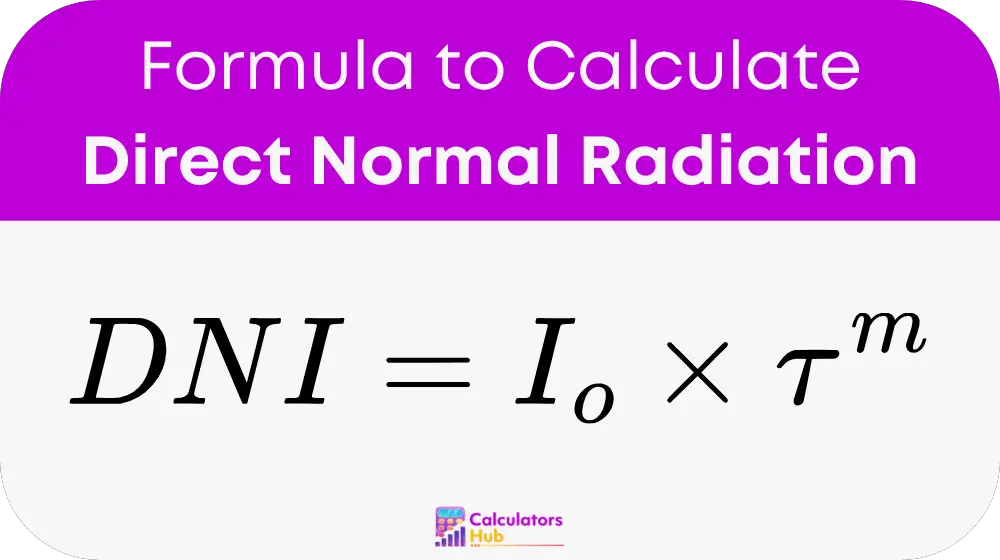
Where:
- DNI = Direct Normal Irradiance (W/m²)
- I₀ = extraterrestrial solar radiation constant (approximately 1367 W/m²)
- τ = atmospheric transmittance factor (dimensionless, typically between 0.6-0.8)
- m = air mass ratio (optical path length through the atmosphere relative to the path length at zenith)
To calculate the air mass ratio:
m = 1 / sin(α)
Where:
- α = solar altitude angle in degrees (angle between the horizon and the sun)
This equation allows accurate calculation of direct normal radiation based on the atmospheric and solar position parameters.
Reference Table for Direct Normal Radiation Values
This table provides approximate DNI values under different atmospheric transmittance conditions at varying solar altitude angles.
| Solar Altitude Angle (α) | Air Mass Ratio (m) | Atmospheric Transmittance (τ) | DNI (W/m²) |
|---|---|---|---|
| 90° (sun at zenith) | 1.00 | 0.8 | 1093.6 |
| 60° | 1.15 | 0.75 | 1035.6 |
| 45° | 1.41 | 0.7 | 960.0 |
| 30° | 2.00 | 0.65 | 890.5 |
| 15° | 3.86 | 0.6 | 820.2 |
This table helps users quickly estimate DNI values without performing calculations manually.
Example of Direct Normal Radiation Calculator
A solar engineer wants to calculate the Direct Normal Irradiance for a location where:
- The solar altitude angle (α) is 45°
- The atmospheric transmittance factor (τ) is 0.7
Step 1: Calculate the air mass ratio
m = 1 / sin(45°)
m = 1 / 0.707 = 1.41
Step 2: Apply the DNI formula
DNI = 1367 × 0.7¹·⁴¹
DNI ≈ 960 W/m²
This means that the location receives approximately 960 W/m² of direct solar radiation.
Most Common FAQs
DNI is crucial for designing solar energy systems, especially for concentrated solar power (CSP) and solar tracking systems that require direct sunlight for maximum efficiency.
Higher atmospheric transmittance (τ) results in greater DNI, while lower transmittance due to clouds, dust, or pollution reduces the amount of direct radiation reaching the surface.
DNI is mainly used for solar tracking systems. Fixed solar panels rely more on Global Horizontal Irradiance (GHI), which includes both direct and diffuse radiation.