The Coherence Length Calculator is a specialized tool used to determine the coherence length of a light source. Coherence length is a measure of how far a wave can propagate while maintaining a predictable phase relationship. It plays a vital role in fields like optics, laser technology, fiber communication, and spectroscopy. By accurately calculating the coherence length, researchers and engineers can design and analyze optical systems for maximum efficiency and reliability. This calculator belongs to the category of optical measurement tools, aiding in understanding and optimizing wave behavior in various mediums.
Formula of Coherence Length Calculator
The coherence length is calculated using the formula:
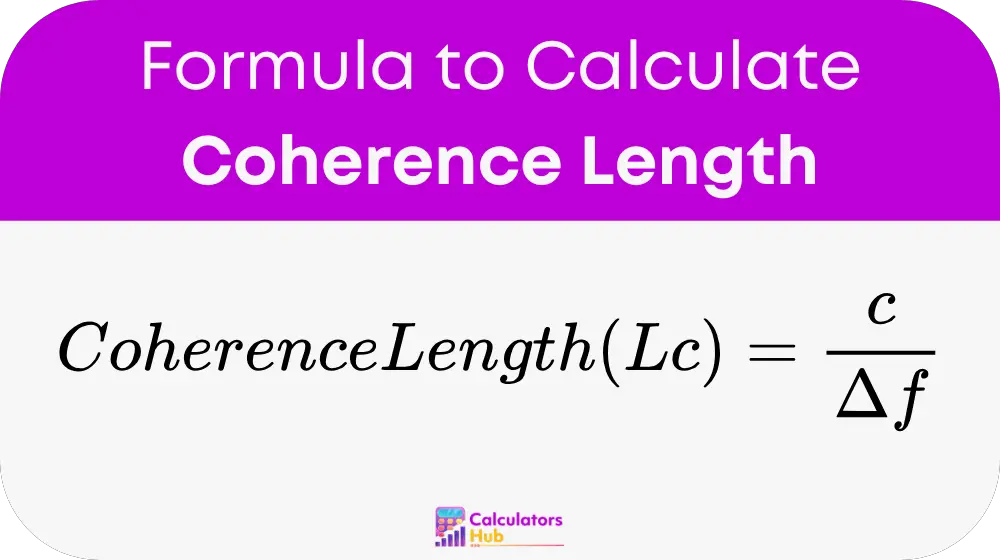
Where:
- Lc is the coherence length.
- c is the speed of light in a vacuum (approximately 3 × 10⁸ m/s).
- Δf is the bandwidth of the light source (in Hz).
Detailed Formulas for Variables:
Bandwidth (Δf):
If not directly given, bandwidth can be calculated from the frequency spread of the light source:
Δf = f_high – f_low
Where:
- f_high is the upper frequency limit.
- f_low is the lower frequency limit.
Speed of Light in a Medium:
If the wave travels in a medium other than a vacuum, adjust the speed of light as:
c_medium = c / n
Where:
- c is the speed of light in a vacuum.
- n is the refractive index of the medium.
These formulas ensure accurate determination of coherence length, accommodating variations in the wave’s frequency range and propagation medium.
Pre-Calculated Table for Coherence Length Scenarios
Below is a table summarizing coherence lengths for common scenarios, helping users avoid manual calculations:
| Bandwidth (Δf) | Medium | Refractive Index (n) | Coherence Length (Lc) |
|---|---|---|---|
| 1 GHz | Vacuum | 1.0 | 300 meters |
| 10 GHz | Vacuum | 1.0 | 30 meters |
| 1 GHz | Glass (n = 1.5) | 1.5 | 200 meters |
| 100 GHz | Water (n = 1.33) | 1.33 | 2.26 meters |
| 1 THz | Vacuum | 1.0 | 0.3 meters |
This table is particularly useful for quick reference in practical optical system design.
Example of Coherence Length Calculator
Let’s calculate the coherence length for a laser source:
- The light source has a frequency spread of 10 GHz (Δf = 10 × 10⁹ Hz).
- The light propagates through glass with a refractive index of 1.5.
Step 1: Adjust Speed of Light in Glass
c_medium = c / n
c_medium = 3 × 10⁸ / 1.5 = 2 × 10⁸ m/s
Step 2: Calculate Coherence Length
Lc = c_medium / Δf
Lc = 2 × 10⁸ / 10 × 10⁹ = 0.02 meters or 2 centimeters
Thus, the coherence length is 2 centimeters, a critical value for designing optical systems involving this laser.
Most Common FAQs
A good coherence length depends on the application. For instance, longer coherence lengths are desirable in interferometry, while shorter lengths might suffice for applications like broadband spectroscopy.
Bandwidth and coherence length are inversely proportional. Higher bandwidth results in shorter coherence lengths, while lower bandwidth yields longer coherence lengths.
Yes, the Coherence Length Calculator applies to any light source, including LEDs and broadband light sources, as long as the bandwidth and propagation medium are known.