Downstream Pressure (P₂): —
The Choked Flow Calculator is a vital tool for calculating mass flow rates in systems that operate under choked flow conditions. Choked flow occurs when a gas or fluid passes through a restriction (such as a valve, nozzle, or orifice) and the flow reaches its maximum limit due to a pressure drop. Beyond this point, even if the upstream pressure increases, the mass flow rate cannot increase further.
This phenomenon is crucial in industries like aerospace, chemical processing, and oil and gas, where accurate control of flow rates is essential for the design and safety of pressurized systems. The choked flow calculator helps engineers and technicians quickly determine the maximum mass flow rate under such conditions, optimizing system performance and preventing unsafe operation.
Formula for Choked Flow Pressure Calculator
The formula to calculate the mass flow rate in a choked flow system is as follows:
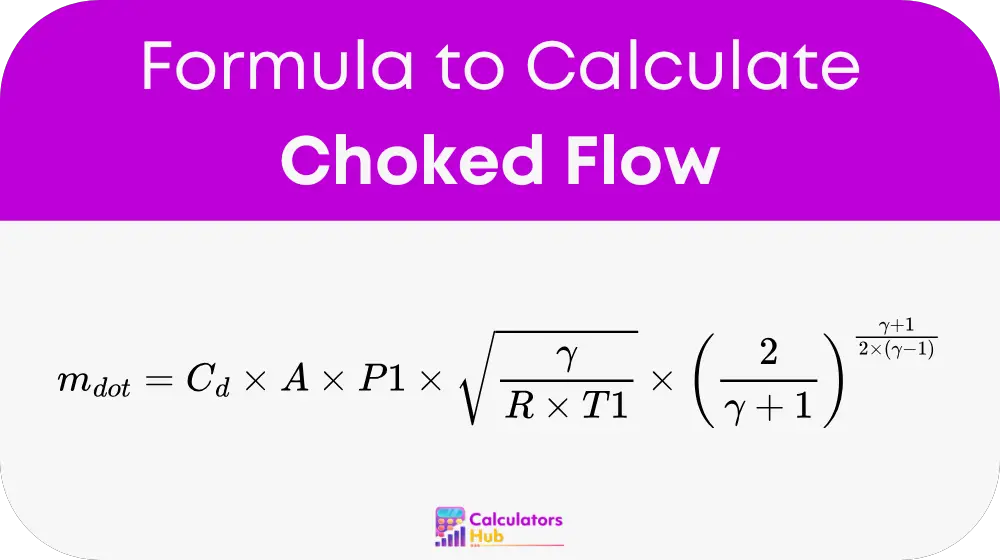
Where:
- m_dot: Mass flow rate (kg/s) – the amount of fluid or gas passing through the restriction per second.
- C_d: Discharge coefficient (dimensionless) – a factor accounting for flow efficiency, typically ranging from 0.6 to 0.8.
- A: Cross-sectional area of the restriction (m²) – the size of the opening through which the gas flows.
- P1: Upstream absolute pressure (Pa) – the pressure of the gas before it passes through the restriction.
- γ (Gamma): Specific heat ratio (dimensionless), also known as the adiabatic index, which is the ratio of specific heat at constant pressure to specific heat at constant volume.
- R: Specific gas constant (J/kg·K) – a constant that relates the energy of a gas to its temperature and pressure.
- T1: Upstream absolute temperature (K) – the temperature of the gas before it enters the restriction.
Breaking Down the Formula:
- The C_d accounts for the efficiency of the flow process, considering factors like turbulence and friction.
- A is the area of the restriction and determines how much space the gas has to pass through.
- P1, γ, and T1 together represent the state of the gas entering the restriction. These factors influence the gas’s behavior under pressure and temperature changes.
- The term (2 / (γ + 1))^((γ + 1) / (2 × (γ – 1))) accounts for the effects of the gas’s compressibility, which is especially important in high-speed gas flows where changes in density and pressure are significant.
Assumptions for Choked Flow Pressure Calculator
When using the choked flow calculator, several assumptions are made to simplify the calculations and ensure they are accurate under ideal conditions:
- Isentropic Flow: The flow is considered adiabatic (no heat transfer) and reversible, meaning no energy is lost to friction or other dissipative forces.
- Ideal Gas Behavior: The gas is assumed to behave ideally, meaning it follows the ideal gas law perfectly under the given conditions.
- One-Dimensional Flow: The flow is assumed to be along a single direction through the restriction, ignoring the effects of flow in other directions.
- Steady-State Flow: The flow conditions are assumed to be steady, meaning that the properties of the fluid or gas do not change over time.
Common Terminologies and Conversions
Below is a table of key terms and their conversions that are commonly encountered in choked flow calculations:
| Term | Description | Conversion Formula |
|---|---|---|
| Mass Flow Rate (m_dot) | The amount of fluid or gas passing through the restriction. | m_dot = C_d × A × P1 × sqrt(γ / (R × T1)) × (2 / (γ + 1))^((γ + 1) / (2 × (γ – 1))) |
| Discharge Coefficient (C_d) | A dimensionless factor accounting for the efficiency of the flow through the restriction. | Typically between 0.6 and 0.8 for most systems. |
| Specific Heat Ratio (γ) | The ratio of specific heat at constant pressure to constant volume. | Typically 1.4 for air. |
| Upstream Pressure (P1) | Pressure of the gas or fluid before it enters the restriction. | Measured in Pascals (Pa) or psi. |
| Specific Gas Constant (R) | A constant used to describe the behavior of gases. | 287 J/kg·K for air. |
| Upstream Temperature (T1) | The absolute temperature of the gas before it enters the restriction. | Measured in Kelvin (K). |
Example of Choked Flow Pressure Calculator
Let’s go through an example of calculating the mass flow rate in a choked flow scenario:
Given:
- Discharge Coefficient (C_d) = 0.7
- Cross-sectional Area (A) = 0.01 m²
- Upstream Pressure (P1) = 2 × 10⁵ Pa
- Specific Heat Ratio (γ) = 1.4
- Specific Gas Constant (R) = 287 J/kg·K
- Upstream Temperature (T1) = 300 K
Using the formula:
m_dot = 0.7 × 0.01 × 2 × 10⁵ × sqrt(1.4 / (287 × 300)) × (2 / (1.4 + 1))^((1.4 + 1) / (2 × (1.4 – 1)))
After performing the calculations:
m_dot ≈ 4.28 kg/s
Most Common FAQs
Choked flow occurs when the mass flow rate through a restriction (such as a valve or nozzle) reaches its maximum possible limit due to a pressure difference. It is crucial in systems where flow rates need to be precisely control, such as in pipelines, combustion chambers, and aircraft engines.
The discharge coefficient reflects how efficiently the gas flows through the restriction. A higher discharge coefficient results in a higher mass flow rate, meaning the system is more efficient at passing gas. Lower values of C_d indicate more resistance to flow.
The choked flow formula is primarily design for gases. However, a similar approach can be use for liquids, with adjustments for the specific properties of the liquid, such as its density and compressibility.