Cauchy’s constant experiment calculators are valuable tools used in the field of optics and material science to compute the refractive index of materials at various wavelengths of light. These calculators simplify the complex process of determining the refractive index (n(λ)) by using Cauchy's equation, which relates the refractive index to the wavelength of light.
In many optical experiments, understanding how light bends or refracts as it passes through different materials is crucial. By using the Cauchy’s constant experiment calculator, researchers and engineers can quickly determine the refractive index without performing lengthy calculations manually. This is particularly important in experiments related to lenses, fibers, and other optical components, where precise control over light propagation is necessary.
Formula of Cauchy’s Constant Experiment Calculators
Cauchy’s equation is the foundation of the calculations involved in determining the refractive index at a given wavelength. The general form of Cauchy’s equation is:
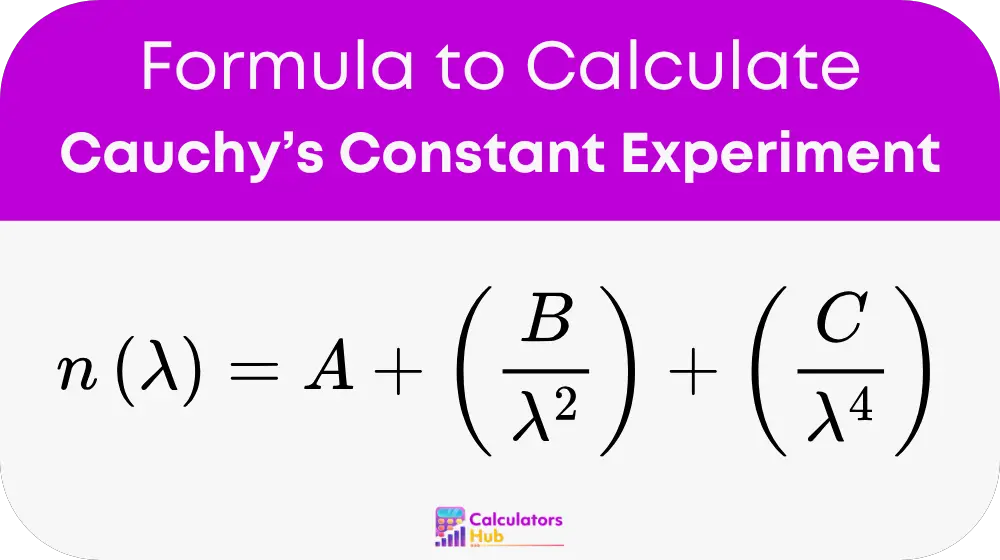
Where:
- n(λ) = Refractive index of the material at wavelength λ
- λ = Wavelength of light, often measured in micrometers (µm) or nanometers (nm)
- A = Cauchy’s constant, which represents the baseline refractive index at an infinite wavelength
- B = Cauchy’s coefficient for the term related to λ^2, representing the dispersion effect of the material
- C = Additional dispersion constant related to λ^4 (optional in simpler models)
In many cases, only the first two terms are used, resulting in the simplified version of the equation:
n(λ) = A + (B / λ^2)
Where:
- A = Primary Cauchy constant, which can be derived from empirical measurements at different wavelengths.
- B = Secondary Cauchy constant, representing the primary dispersion effect at different wavelengths.
This simplified version of Cauchy’s equation is commonly used in applications where higher-order dispersion effects (represented by C) are negligible or not considered.
General Terms for Cauchy’s Constant Calculations
To help users understand and use the Cauchy’s Constant Experiment Calculator effectively, here are some key terms and concepts that are commonly searched for in relation to the refractive index and Cauchy’s equation:
| Term | Definition |
|---|---|
| Refractive Index (n) | A measure of how much light is bent, or refracted, when it passes through a material. |
| Wavelength (λ) | The distance between two consecutive peaks (or troughs) of a wave, often measured in micrometers or nanometers. |
| Cauchy’s Constant (A) | A constant in Cauchy’s equation representing the refractive index at an infinite wavelength. |
| Dispersion | The variation in the refractive index of a material with respect to wavelength. |
| Cauchy’s Coefficient (B) | The coefficient in the equation that quantifies the dispersion at different wavelengths. |
| Micrometer (µm) | A unit of length used to measure wavelengths of light, equivalent to one-millionth of a meter. |
| Nanometer (nm) | A unit of length used to measure very short wavelengths, equivalent to one-billionth of a meter. |
This table provides a quick reference for understanding the terminology involved in Cauchy’s constant calculations, making it easier for users to engage with the calculator effectively.
Example of Cauchy’s Constant Experiment Calculators
Let’s walk through an example to see how the Cauchy’s equation works in practice. Suppose you are working with a material that has the following properties:
- Cauchy’s constant, A = 1.45
- Cauchy’s coefficient, B = 0.004
- Wavelength of light (λ) = 0.55 µm (green light)
To calculate the refractive index at this wavelength, you can use the simplified version of Cauchy’s equation:
n(λ) = A + (B / λ^2)
Substitute the values:
n(0.55) = 1.45 + (0.004 / (0.55)^2)
First, calculate the value of λ^2:
n(0.55) = 1.45 + (0.004 / 0.3025)
Now, divide 0.004 by 0.3025:
n(0.55) = 1.45 + 0.0132
Finally, add the results:
n(0.55) = 1.4632
Therefore, the refractive index of the material at a wavelength of 0.55 µm is approximately 1.4632. This result can be use for optical design, including lens or fiber development, where the refractive index plays a critical role in understanding light behavior.
Most Common FAQs
Cauchy’s constant is crucial because it allows researchers to determine how light interacts with different materials at varying wavelengths. This is essential in the design and optimization of optical devices like lenses, mirrors, and fiber optics. By knowing the refractive index, one can predict how light will propagate through a material.
Cauchy’s equation is widely use because it provides a simple yet effective way to model the refractive index as a function of wavelength. It is especially useful for transparent materials where higher-order dispersion effects are not significant. The equation gives an accurate estimation for many materials in the visible light range.
While Cauchy’s equation is highly effective for many transparent materials, it may not be accurate for all materials, especially those with high dispersion or non-linear optical properties. In such cases, more complex models may be necessary to accurately predict the refractive index.