The Binding Energy Per Nucleon Calculator is a tool used in nuclear physics to determine the binding energy per nucleon in a nucleus. This value is essential for understanding the stability of a nucleus. The binding energy per nucleon gives insight into how tightly bound the nucleons (protons and neutrons) are within the nucleus. A higher binding energy per nucleon generally indicates a more stable nucleus, which is less likely to undergo radioactive decay. This calculator simplifies the process of determining this value, making it easier for researchers, students, and professionals to analyze nuclear stability.
Formula of Binding Energy Per Nucleon Calculator
The formula used to calculate the binding energy per nucleon is:
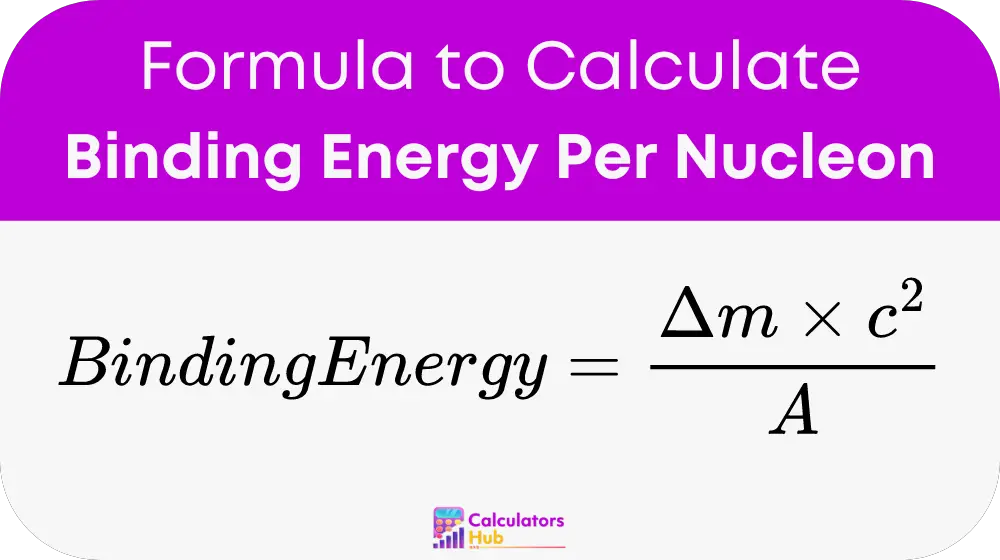
Where:
- Δm is the mass defect, which is the difference between the total mass of the separate nucleons (protons and neutrons) and the actual mass of the nucleus. This mass defect is typically measured in atomic mass units (u) or kilograms (kg).
- c is the speed of light in a vacuum, approximately 3.00 × 10⁸ meters per second.
- A is the mass number, which is the total number of nucleons (protons and neutrons) in the nucleus.
This formula provides a direct calculation of the binding energy per nucleon, allowing for a clear comparison between different nuclei in terms of their stability.
General Reference Values
Here’s a table that provides general reference values for the binding energy per nucleon of common isotopes. These values can help you quickly estimate the binding energy per nucleon for different elements without needing to perform the calculation each time.
| Isotope | Mass Number (A) | Binding Energy Per Nucleon (MeV) | Description |
|---|---|---|---|
| Helium-4 (He-4) | 4 | 7.07 | Stable nucleus, commonly used as a reference. |
| Carbon-12 (C-12) | 12 | 7.68 | Another stable nucleus, widely studied in nuclear physics. |
| Uranium-235 (U-235) | 235 | 7.59 | A commonly used isotope in nuclear reactors and weapons. |
| Iron-56 (Fe-56) | 56 | 8.79 | Has one of the highest binding energies per nucleon, indicating high stability. |
These reference values offer a quick understanding of the binding energy per nucleon across different isotopes, helping in the analysis of nuclear stability.
Example of Binding Energy Per Nucleon Calculator
Let’s walk through an example to see how the Binding Energy Per Nucleon Calculator works in practice.
Scenario:
You are calculating the binding energy per nucleon for an isotope of Carbon-12. The mass of the Carbon-12 nucleus is 12 atomic mass units (u), and the total mass of the separate nucleons (6 protons and 6 neutrons) is 12.0989 u. The mass defect (Δm) is the difference between these two values.
Calculation:
First, calculate the mass defect:
Δm = 12.0989 u - 12 u = 0.0989 u
Next, convert this mass defect into energy using the formula:
Binding Energy = Δm * c²
Since 1 atomic mass unit (u) is equivalent to 931.5 MeV/c², the binding energy is:
Binding Energy = 0.0989 u * 931.5 MeV/u = 92.13 MeV
Finally, calculate the binding energy per nucleon:
Binding Energy Per Nucleon = 92.13 MeV / 12 = 7.68 MeV
This result indicates that the binding energy per nucleon for Carbon-12 is approximately 7.68 MeV, reflecting its stability.
Most Common FAQs
Binding energy per nucleon provides insight into the stability of a nucleus. A higher value typically means that the nucleons are more tightly bound, making the nucleus more stable and less likely to undergo radioactive decay.
In nuclear fusion, lighter nuclei combine to form a heavier nucleus, releasing energy because the binding energy per nucleon in the resulting nucleus is higher. In nuclear fission, a heavy nucleus splits into lighter nuclei, also releasing energy due to the increase in binding energy per nucleon in the fission products.
Yes, the Binding Energy Per Nucleon Calculator can be used for any element or isotope, provided the mass defect and mass number are known. It is a versatile tool applicable across the periodic table, aiding in the study of nuclear physics and chemistry.