The Beam Equation Calculator helps engineers and designers determine the deflection, bending moment, and stress in a beam subjected to various loads. By inputting the beam’s material properties, cross-sectional dimensions, and loading conditions, the calculator provides accurate results that are critical for structural analysis. This tool is invaluable for ensuring that beams are designed to carry the expected loads without excessive deflection or failure, thereby contributing to the safety and reliability of structures.
Formula of Beam Equation Calculator
The Beam Equation Calculator primarily uses the following formula to calculate the deflection of a beam:
General Beam Equation:
Deflection (y) at a point x along the beam:
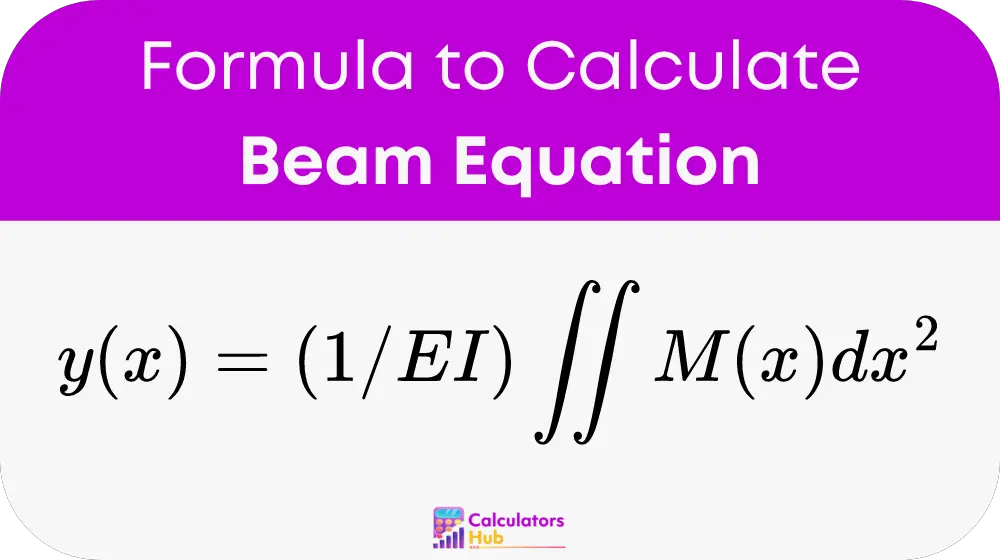
Explanation:
- y(x): The deflection at position x along the length of the beam.
- E: Young’s modulus of the material, which measures the stiffness of the material.
- I: The second moment of area (also known as the moment of inertia) of the beam’s cross-section, which reflects the beam’s resistance to bending.
- M(x): The bending moment at position x along the beam, which is the moment of force causing the beam to bend.
How It Works:
- E (Young’s Modulus): This value depends on the material of the beam, such as steel, concrete, or wood. It represents the material’s stiffness.
- I (Moment of Inertia): This value is determined by the cross-sectional shape of the beam (e.g., rectangular, circular). It indicates the beam’s resistance to bending.
- M(x) (Bending Moment): This value is calculated based on the loads applied to the beam, including point loads, distributed loads, and moments.
By integrating the bending moment along the length of the beam and considering the material properties, the Beam Equation Calculator determines the beam’s deflection at any point.
Table for General Terms
To provide a clearer understanding of the concepts involved in beam analysis, here’s a table of key terms:
| Term | Definition |
|---|---|
| Deflection (y) | The displacement of a beam under load, measured at a specific point along its length. |
| Young’s Modulus (E) | A measure of a material’s stiffness, indicating how much it will deform under a given load. |
| Moment of Inertia (I) | A geometric property of the beam’s cross-section that reflects its resistance to bending. |
| Bending Moment (M) | The moment of force causing the beam to bend at a specific point along its length. |
| Load | The forces applied to a beam, including point loads, distributed loads, and moments. |
Example of Beam Equation Calculator
Let’s walk through an example to demonstrate how the Beam Equation Calculator works:
Scenario
Suppose you are designing a simply supported steel beam with a rectangular cross-section. The beam has a span of 10 meters and is subjected to a uniform distributed load of 5 kN/m. You need to calculate the maximum deflection of the beam.
Inputs:
- Young’s Modulus (E): 200 GPa (for steel)
- Moment of Inertia (I): 0.0002 m^4 (for the given cross-section)
- Length of Beam (L): 10 meters
- Load (w): 5 kN/m
Calculation:
For a simply supported beam with a uniform distributed load, the bending moment (M) at a distance x from the left support is:
- M(x) = (w * x * (L – x)) / 2
The deflection equation y(x) for the beam can be determined by integrating the moment equation twice and applying boundary conditions. However, the Beam Equation Calculator simplifies this process by directly providing the deflection values.
Using the Beam Equation Calculator, we find the maximum deflection at the center of the beam (x = L/2):
- y(max) ≈ 10.42 mm
Interpretation:
The maximum deflection of the beam under the given load is approximately 10.42 mm. This deflection is within the acceptable limits for steel beams, ensuring that the beam will not experience excessive bending or failure.
Most Common FAQs
Calculating beam deflection is crucial because excessive deflection can lead to structural instability, damage to finishes, and discomfort for occupants. Ensuring that deflection is within acceptable limits helps maintain the safety and serviceability of the structure.
Yes, the Beam Equation Calculator can be use for various types of beams, including simply supported, cantilevered, and continuous beams. The calculator can also accommodate different loading conditions, such as point loads, distributed loads, and moments.
The moment of inertia (I) reflects the beam’s resistance to bending. A higher moment of inertia indicates that the beam is more resistant to bending under a given load. It is determined by the shape and size of the beam’s cross-section.