Angular velocity to linear velocity calculator is a practical tool designed to convert angular velocity, typically measured in radians per second, into linear velocity, expressed in meters per second. This conversion is crucial in various scientific and engineering applications where understanding the movement of an object along a circular path is required. The calculator simplifies complex calculations, ensuring accuracy and efficiency in projects and studies related to circular motion.
Formula
To convert angular velocity to linear velocity, the following mathematical formula is use:
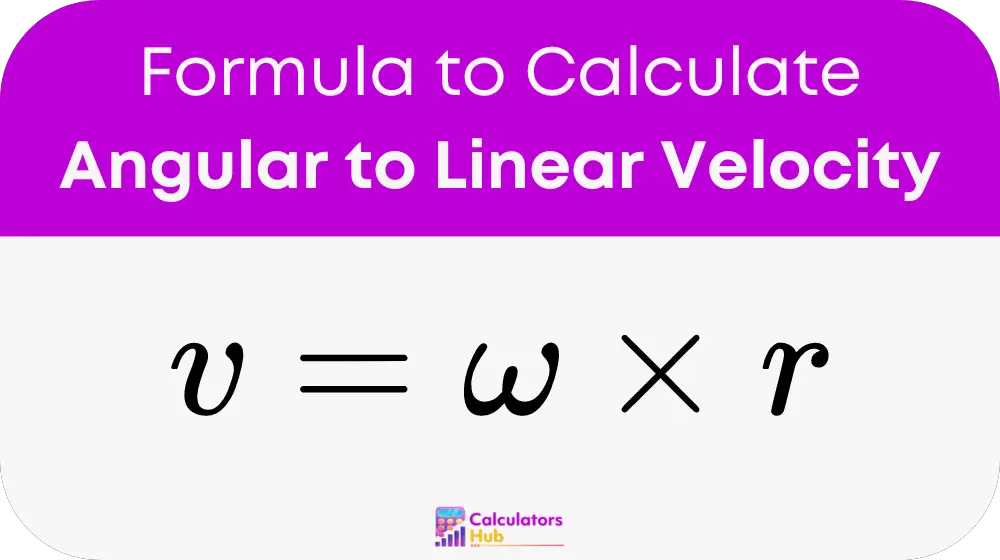
Where:
- v is the linear velocity.
- ω (omega) is the angular velocity.
- r is the radius of the circular path.
Steps to Use the Formula:
- Determine the Angular Velocity (ω): This value is typically given in radians per second (rad/s).
- Measure the Radius (r): This is the distance from the center of the circular path to the point of interest, usually provided in meters (m).
- Multiply Angular Velocity by Radius: Apply the formula v = ω * r to compute the linear velocity.
General Terms Table
The table below provides general terms and their definitions to assist users in better understanding the calculations:
| Term | Definition |
|---|---|
| Angular Velocity (ω) | Speed of rotation around a central point, measured in rad/s |
| Linear Velocity (v) | Speed in a straight line, calculated using v = ω * r |
| Radius (r) | Distance from the center to the edge of a circular path |
Example
To illustrate how the calculator works, consider a wheel rotating at 10 rad/s (ω) with a radius of 0.5 meters (r):
Calculation:
- Linear Velocity (v) = Angular Velocity (ω) × Radius (r)
- v = 10 rad/s × 0.5 m = 5 m/s
Thus, the linear velocity of the wheel's edge is 5 meters per second.
Most Common FAQs
Angular velocity refers to the rate of rotation around a central axis, expressed in radians per second (rad/s).
To convert, multiply the angular velocity (ω) by the radius (r) of the motion's circular path using the formula v = ω * r.
Understanding linear velocity is essential for applications in physics and engineering where motion characteristics need precise control and analysis, such as in robotics and automotive industries.