The Angle of Deviation Calculator is a vital tool in the field of optics, used to determine the angle by which a light ray is deviated as it passes through a prism. This tool is essential for applications in spectroscopy, optical engineering, and various scientific research areas where precise light manipulation is required.
Formula of Angle Of Deviation Calculator
The angle of deviation (δ) can be calculated using the following formula, which involves the prism’s refractive index (n), the angle of incidence (i), and the angle of the prism (A).
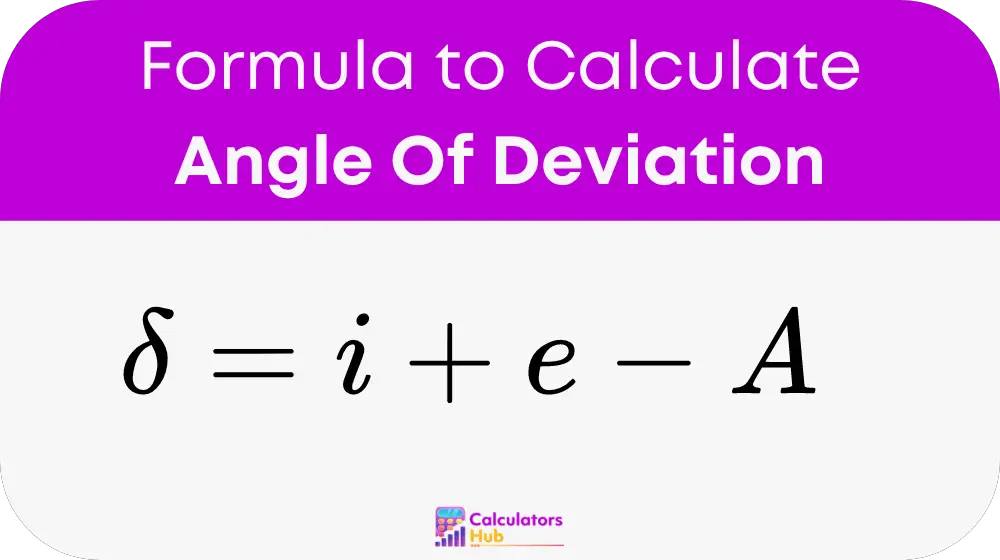
Where:
- δ is the angle of deviation.
- i is the angle of incidence.
- e is the angle of emergence.
- A is the angle of the prism.
In terms of refractive index: sin((A + δ) / 2) = n * sin(A / 2)
Steps for Calculation:
- Measure the angle of incidence (i).
- Measure the angle of emergence (e).
- Identify the angle of the prism (A).
- Use the formula to calculate the angle of deviation (δ): δ = i + e – A
Detailed Process:
- Measure the angle of incidence (i) where the light enters the prism.
- Measure the angle of emergence (e) where the light exits the prism.
- Identify the angle of the prism (A), which is the apex angle of the prism.
- Sum the angles of incidence and emergence:
- Sum = i + e
- Subtract the angle of the prism from the sum:
- δ = Sum – A
For a detailed calculation involving refractive index (n):
- Measure the angle of the prism (A).
- Calculate the refractive index (n) of the prism material.
- Calculate the sine of half the sum of the angle of incidence and angle of deviation:
- sin((A + δ) / 2)
- Multiply the refractive index (n) by the sine of half the prism angle:
- n * sin(A / 2)
- Equate and solve for the angle of deviation:
- sin((A + δ) / 2) = n * sin(A / 2)
Table of General Terms and Calculations
Here is a table that outlines common terms and their definitions, which are useful for understanding and performing angle of deviation calculations:
| Term | Definition |
|---|---|
| Angle of Deviation (δ) | The angle by which a light ray is deviated as it passes through a prism |
| Angle of Incidence (i) | The angle at which light enters the prism |
| Angle of Emergence (e) | The angle at which light exits the prism |
| Angle of the Prism (A) | The apex angle of the prism |
| Refractive Index (n) | A measure of how much the speed of light is reduced inside a medium |
This table provides a quick reference for understanding the essential terms and their roles in the angle of deviation calculations.
Example of Angle Of Deviation Calculator
Consider an example where light passes through a prism with an angle of 60 degrees. The angle of incidence (i) is measured at 40 degrees, and the angle of emergence (e) is measured at 50 degrees. The refractive index (n) of the prism material is 1.5.
- Identify the given values:
- i = 40 degrees
- e = 50 degrees
- A = 60 degrees
- Sum the angles of incidence and emergence:
- Sum = i + e = 40 + 50 = 90 degrees
- Subtract the angle of the prism from the sum:
- δ = Sum – A = 90 – 60 = 30 degrees
- In terms of refractive index:
- sin((A + δ) / 2) = n * sin(A / 2)
- sin(45) = 1.5 * sin(30)
- 0.707 = 1.5 * 0.5
- 0.707 = 0.75 (approx)
This example shows the practical application of the angle of deviation calculation in determining how much a light ray is bent as it passes through a prism.
Most Common FAQs
The angle of deviation is crucial for designing optical instruments like spectrometers and understanding how light interacts with different materials, which is vital for various scientific and industrial applications.
The refractive index determines how much light bends when entering a new medium. A higher refractive index indicates a greater bending of light, resulting in a larger angle of deviation.
Yes, the calculator can be use for all types of prisms as long as the necessary measurements (angle of incidence, angle of emergence, and angle of the prism) are accurately provided.