The Amplitude to Acceleration Calculator is a vital tool for engineers and scientists, particularly in fields dealing with vibrational analysis and dynamic systems. This calculator transforms the amplitude of sinusoidal motion—essentially the peak or maximum displacement from a neutral position—into peak acceleration values. This conversion is crucial for understanding how different frequencies and amplitudes affect the dynamics of a system.
Formula of Amplitude to Acceleration Calculator
To convert amplitude to acceleration, the calculator uses the following equation:
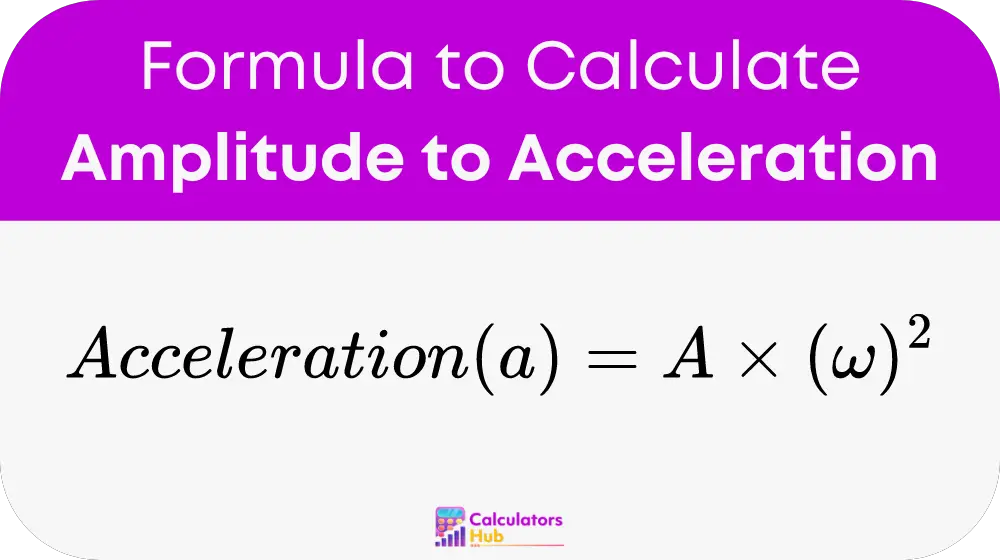
Variables:
- Amplitude (A): The maximum displacement from the mean position, measured in meters (m).
- Angular Frequency (ω): The rate of change of the phase of the sinusoidal wave, measured in radians per second (rad/s).
Detailed Calculation:
- Identify the Amplitude (A): Measure or determine the maximum displacement from the mean position in meters.
- Determine the Angular Frequency (ω): Calculate or identify the angular frequency in radians per second.
- Calculate the Acceleration (a): Multiply the square of the angular frequency by the amplitude to find the peak acceleration.
Table for General Terms
The table below provides a quick reference for common terms related to amplitude and acceleration calculations, including basic conversions and related physical concepts:
| Term | Description | Formula/Conversion |
|---|---|---|
| Amplitude (A) | Maximum displacement from the mean position. | Measured in meters (m) |
| Angular Frequency (ω) | Rate of change of the phase of a sinusoidal wave. | ω = 2π × frequency (Hz) |
| Frequency (f) | Number of cycles per second. | f = ω / (2π), measured in Hertz (Hz) |
| Period (T) | Time it takes to complete one cycle. | T = 1 / f, measured in seconds (s) |
| Acceleration (a) | Peak acceleration of the motion. | a = A × ω^2, measured in meters/second^2 (m/s^2) |
This table aims to assist users in quickly understanding and applying the key variables and formulas without in-depth calculation, enhancing both learning and practical application.
Example of Amplitude to Acceleration Calculator
Imagine a vibrating system with an amplitude of 0.5 meters and an angular frequency of 10 radians per second. Using our formula:
Acceleration = 0.5 × (10)^2 = 50 m/s^2
This example clearly demonstrates how to apply the formula to calculate the acceleration from given amplitude and angular frequency values.
Most Common FAQs
Amplitude refers to the maximum extent of a vibration or oscillation, measured from the position of equilibrium.
Angular frequency is calculated by multiplying the frequency of the system (in cycles per second or Hz) by 2π.
Understanding acceleration helps predict the forces involved in vibrating systems, which is crucial for designing safe and effective mechanical structures.