The Blower RPM Calculator is a tool used to determine the revolutions per minute (RPM) of a blower in a mechanical system. This calculation is essential in various industrial applications where blowers are used for ventilation, cooling, heating, or any other process requiring air movement. The RPM of a blower directly affects the airflow rate and efficiency of the system. By accurately calculating the blower's RPM, operators can ensure optimal performance, reduce energy consumption, and prevent potential mechanical failures.
Formula of Blower Rpm Calculator
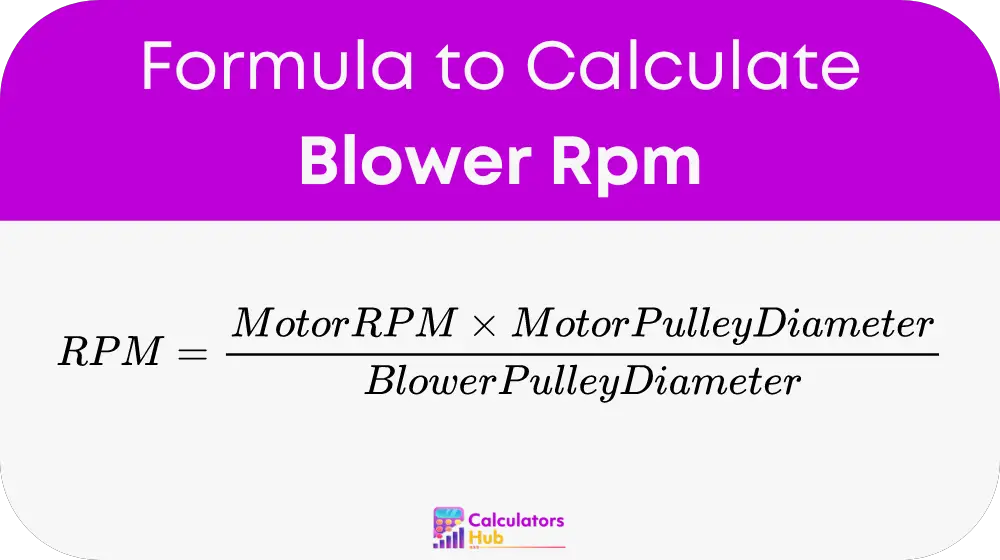
The formula to calculate Blower RPM is as follows:
Detailed Calculation Steps:
- Identify Motor RPM: This is the revolutions per minute of the motor driving the blower. It can usually be find on the motor's nameplate or in its technical specifications.
- Measure Motor Pulley Diameter: This is the diameter of the pulley attach to the motor shaft. It determines how fast the motor's rotational speed is transferred to the blower.
- Measure Blower Pulley Diameter: This is the diameter of the pulley attached to the blower shaft. It controls how the motor's speed is adjusted to drive the blower.
- Multiply Motor RPM by Motor Pulley Diameter: This step gives you a value that represents the product of the motor's speed and the size of the pulley connected to it.
- Divide the Result by the Blower Pulley Diameter: This final step adjusts the motor speed by the ratio of the pulleys to calculate the blower's RPM.
By following these steps, you can accurately determine the blower's RPM, ensuring that it operates within the desired performance range.
Conversion Table
Here’s a table that provides common combinations of motor RPMs and pulley diameters, along with the calculated blower RPM:
| Motor RPM | Motor Pulley Diameter (inches) | Blower Pulley Diameter (inches) | Blower RPM |
|---|---|---|---|
| 1750 | 5 | 10 | 875 |
| 1750 | 6 | 12 | 875 |
| 3450 | 4 | 8 | 1725 |
| 3450 | 5 | 10 | 1725 |
| 1750 | 7 | 14 | 875 |
This table serves as a quick reference for technicians and engineers who need to adjust or verify blower speeds based on different motor and pulley configurations.
Example of Blower Rpm Calculator
Let's walk through an example to demonstrate how the Blower RPM Calculator works.
Suppose you have the following setup:
- Motor RPM: 3450 RPM
- Motor Pulley Diameter: 4 inches
- Blower Pulley Diameter: 8 inches
To calculate the blower's RPM, you would perform the following calculation:
- Blower RPM = (3450 * 4) / 8 = 13800 / 8 = 1725 RPM
In this example, the blower RPM is 1725, which would be use to determine the appropriate settings for the blower to ensure efficient operation.
Most Common FAQs
Accurately calculating blower RPM is crucial because it directly affects the airflow rate, efficiency, and overall performance of the system. Incorrect RPM can lead to reduced efficiency, increased energy consumption, or even mechanical failure.
Yes, changing the sizes of the motor or blower pulleys will directly impact the blower RPM. Larger motor pulleys increase the blower speed, while larger blower pulleys decrease it.
If the blower RPM is too high, it may cause excessive wear, noise, and energy consumption. If it’s too low, the system may not provide sufficient airflow, leading to reduced efficiency or inadequate performance.
A squirrel cage blower has a 1625 rpm motor which ran for years and finally failed. A 1725 rpm motor of the same HP, running voltage, etc. is available. What is the difference in pressure, air velocity, etc. generated by the 1725 rpm motor vs the old 1625 rpm motor and how is that calculated? Is this a (new rpm/old rpm) ^2 relationship?
Your understanding is correct. The change in airflow, pressure, and power follows the fan affinity laws.
For Air Flow (CFM): It increases linearly with the speed ratio
New Flow = Old Flow × (1725/1625) = Old Flow × 1.062
So you’ll get about 6.2% more airflow.
For Pressure: It increases with the square of the speed ratio
New Pressure = Old Pressure × (1725/1625)² = Old Pressure × 1.127
So you’ll see about 12.7% higher pressure.
For Power Required: It increases with the cube of the speed ratio
New Power = Old Power × (1725/1625)³ = Old Power × 1.196
This means approximately 19.6% more power consumption.
Keep in mind that the increased power requirement might impact the motor’s efficiency and operational limits. If your system was already near capacity, you should verify that it can handle the additional load.