The ml to cm calculator is designed to convert the volume of a substance, provided in milliliters, into the equivalent cubic dimension—specifically, the length of a cube’s side in centimeters. This conversion is particularly useful in visualizing the space that a certain volume would occupy in a three-dimensional cube, aiding in various practical and educational applications.
Formula of ml to cm Calculator
To understand how the ml to cm calculator works, it’s important to grasp the underlying formula:
Conversion Basis: 1 milliliter (ml) is equivalent to 1 cubic centimeter (cm³).
Volume to Length Relationship: For a cube, the volume (cm³) equals the cube of the side length (Length in cm³).
Formula for Conversion:
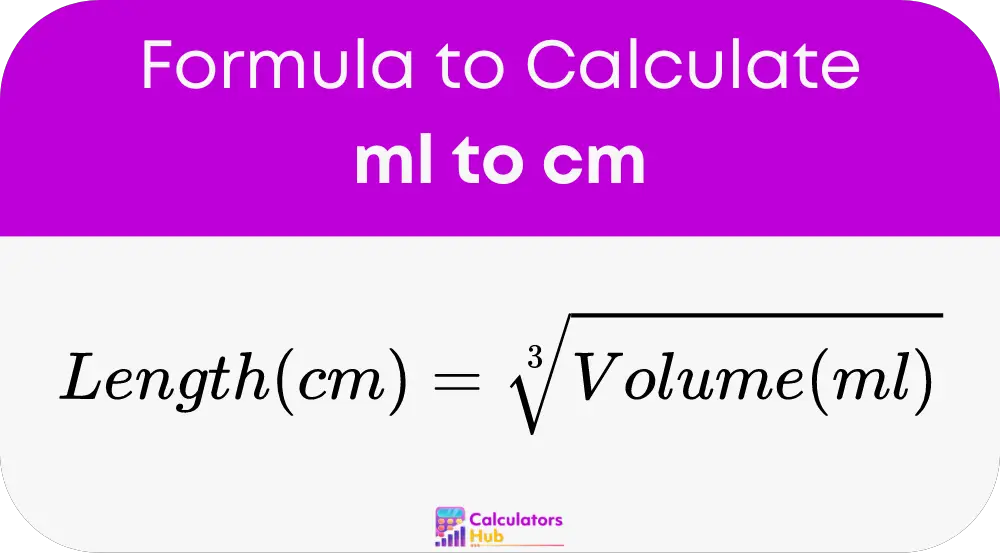
This formula is the cornerstone of converting a given volume in milliliters to the corresponding side length of a cube in centimeters.
Conversion Table
Here is a handy conversion table that provides quick reference values from milliliters to centimeters:
| Milliliters (ml) | Length of Cube’s Side (cm) |
|---|---|
| 1 | 1 |
| 10 | 2.15 |
| 50 | 3.68 |
| 100 | 4.64 |
| 500 | 7.94 |
| 1000 | 10 |
This table can be used to bypass calculations for frequently encountered values, providing a convenient reference.
Example of ml to cm Calculator
Let’s demonstrate the use of the ml to cm calculator with an example:
- Scenario: Converting the volume of a 250 ml water bottle to the length of a cube’s side.
- Application of Formula: Length = 250^(1/3) ≈ 6.30 cm
- Interpretation: A cube that has a volume of 250 ml would have each side measuring approximately 6.30 cm.
Most Common FAQs
Milliliters and cubic centimeters are equivalent units for measuring volume, commonly used in different contexts.
This conversion is valuable for tasks that require knowledge of volume in a spatial context, such as filling containers or understanding ingredient quantities in cooking.
While the conversion is mathematically accurate for cubes, real-world applications may vary due to the shape and distribution of the volume.