The Diagonal Depth Calculator is designed to compute the diagonal length across the three-dimensional space of a rectangular prism. This calculation is essential in numerous applications, from determining the correct packaging dimensions to ensuring that components fit perfectly in machine assembly.
Formula of Diagonal Depth Calculator
To find the diagonal depth of any rectangular prism, the formula is:
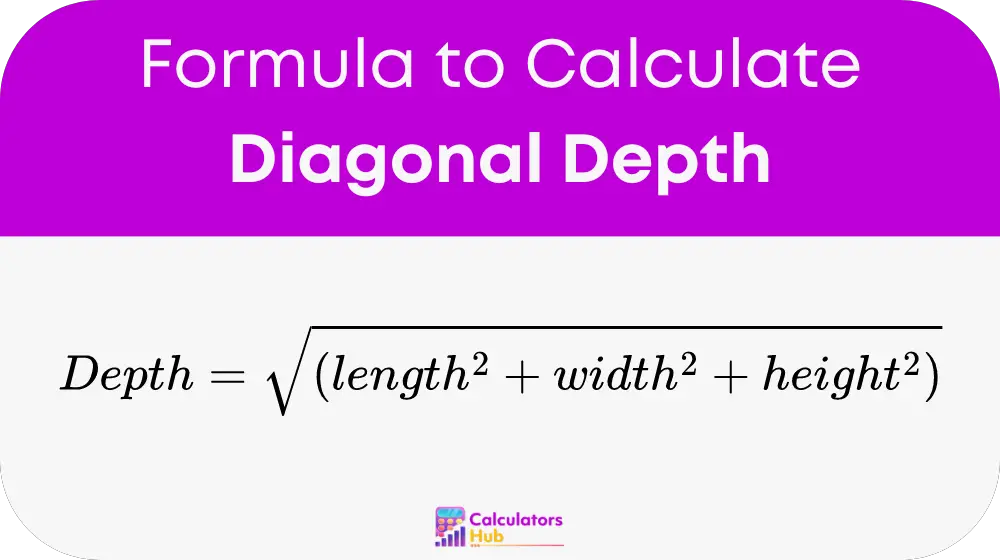
This formula incorporates:
- Length: the longest side of the rectangle.
- Width: the shorter side of the rectangle adjacent to the length.
- Height: the vertical measurement from the base to the top of the rectangle.
Understanding and using this formula allows for precise dimensional analysis and helps in optimizing design and construction processes.
Conversion Table and Related Calculators
Below is a table showing common dimensions and their resulting diagonal depths, providing a quick reference for frequent measurements. Additionally, related calculators for volume and surface area can offer a more comprehensive suite of tools for our users, aiding in a variety of necessary computations.
| Length | Width | Height | Diagonal Depth |
|---|---|---|---|
| 10 cm | 10 cm | 10 cm | 17.32 cm |
| 20 cm | 20 cm | 20 cm | 34.64 cm |
| 50 cm | 30 cm | 40 cm | 70.71 cm |
| (Values are approximations for illustrative purposes.) |
Example of Diagonal Depth Calculator
Consider a box with dimensions 30 cm in length, 40 cm in width, and 50 cm in height. Using our formula:
Diagonal Depth = √(30^2 + 40^2 + 50^2) = √(900 + 1600 + 2500) = √5000 ≈ 70.71 cm
This example demonstrates the ease with which the calculator can be used to derive necessary measurements efficiently.
Most Common FAQs
Diagonal depth refers to the longest possible straight line that can be drawn from one corner of a rectangular prism to the opposite corner, traversing through the body of the prism.
Calculating diagonal depth is vital for space optimization, fitting components, and ensuring that parts align correctly in construction and design.
The calculator is highly accurate, relying on the mathematical precision of the Pythagorean theorem, making it reliable for critical decisions and calculations.