A 3D Spiral, often referred to as a helix, is a curve in three-dimensional space characterized by its unique coiling pattern. The 3D Spiral Length Calculator is a specialized tool designed to compute the total length of this spiral based on several key parameters: the radius of the base circle, the height of each coil, and the number of coils. This tool is invaluable for various fields, including mechanical engineering, construction, and physics, where precise calculations are critical for successful project execution.
Formula of 3D Spiral Length
To calculate the length of a 3D spiral, you'll need to know the following parameters:
- Radius of the base circle (r)
- Height of each coil (h)
- Number of coils (n)
Assuming the spiral is made up of circular coils, the formula to calculate the length of the spiral is:
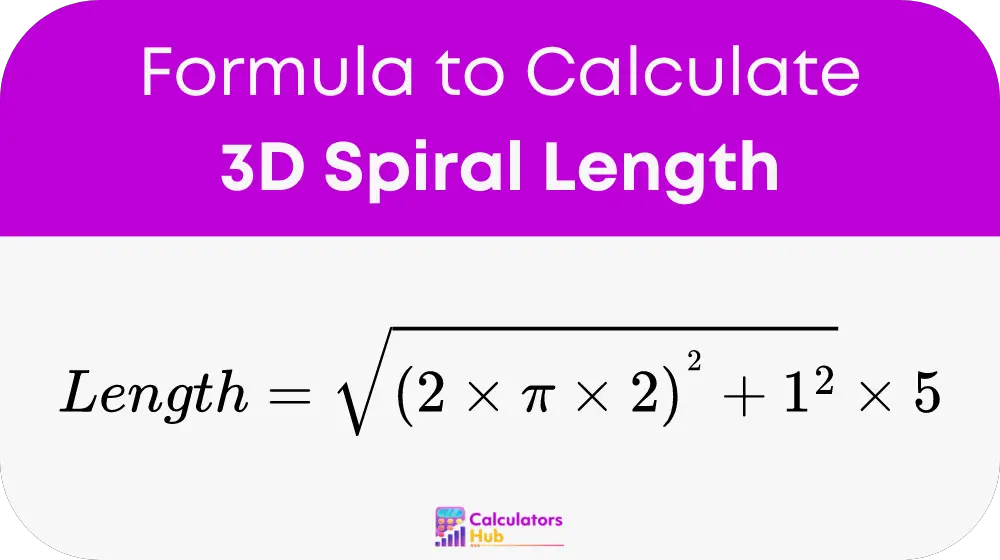
This formula combines the circumference of each coil (which is 2πr) with the vertical distance travel in each coil (h). The total length is the sum of the hypotenuse form by the radius and height in each coil, multiplied by the number of coils.
Useful Table for Quick Reference
Here is a table providing preset calculations for different dimensions of spirals to aid users in quick reference without needing to calculate each time manually:
| Radius (r) | Height (h) | Number of Coils (n) | Approx. Length |
|---|---|---|---|
| 1 cm | 0.5 cm | 10 | 31.4 cm |
| 2 cm | 1 cm | 5 | 44.7 cm |
| 3 cm | 1.5 cm | 3 | 54.8 cm |
This table facilitates users in estimating spiral lengths for common configurations, enhancing the calculator's utility and user-friendliness.
Example of 3D Spiral Length
Consider a spiral with a radius of 2 cm, a coil height of 1 cm, and 5 coils. Using our formula:
Length = sqrt(2×π×2)2+12×5 = 44.7 cm
This example clarifies how the calculator functions and demonstrates its accuracy and reliability in practical scenarios.
Most Common FAQs
A1: The calculator is frequently use in mechanical engineering, architecture, and construction to design springs, coils, and spiral staircases, ensuring precise measurements for optimal functionality.
A2: The calculator is highly accurate, provided the input parameters are correct. It uses a mathematical formula based on geometric principles, ensuring minimal error in the computed length.
A3: Yes, the calculator is versatile and can be use with any units of measurement, as long as all inputs are consistent (e.g., all in centimeters or all in inches).