The Venn Diagram Shader Calculator is an innovative tool that helps users compute the overlapping areas in Venn Diagrams with precision. This calculator is particularly useful for statisticians, data analysts, and educators who require accurate intersection values for two or more sets. By inputting the sizes and relationships of the sets, the calculator provides instant results, saving time and increasing accuracy in data interpretation.
Formula of Venn Diagram Shader Calculator
To understand the functionality of the Venn Diagram Shader Calculator, it’s essential to grasp the formula it uses. The calculator employs the following mathematical expression:
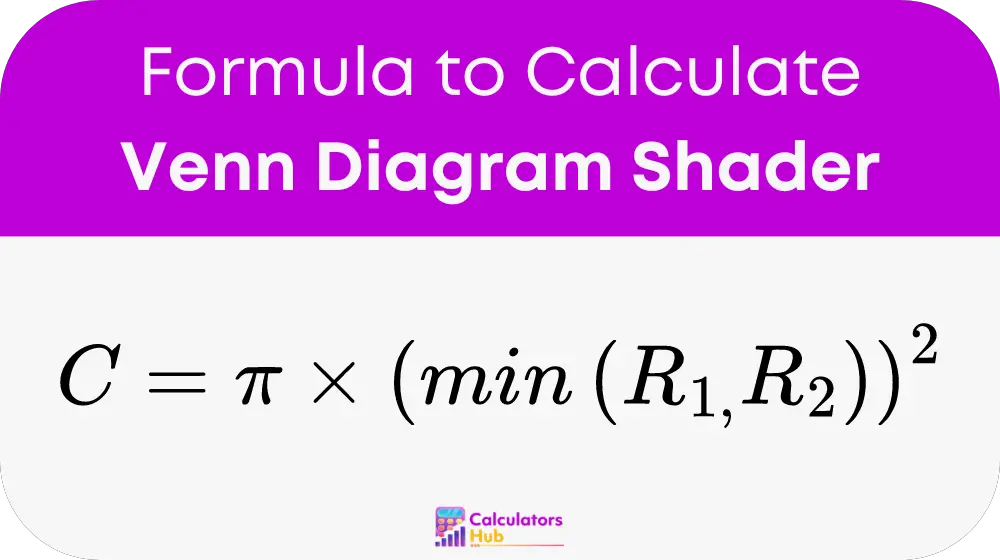
Where:
- C is the area of overlap between two circles.
- R1 and R2 are the radii of the two circles, respectively.
This formula assumes that the circles are perfectly round and the overlapping area is also a perfect circle, which simplifies the calculation but is sufficiently accurate for most practical purposes.
Table of General Terms and Pre-calculated Values
| R1 (Radius of Circle 1) | R2 (Radius of Circle 2) | Overlap Area (C) Calculated |
|---|---|---|
| 2 cm | 2 cm | 12.57 cm² |
| 3 cm | 3 cm | 28.27 cm² |
| 3 cm | 4 cm | 28.27 cm² |
| 4 cm | 4 cm | 50.27 cm² |
| 4 cm | 5 cm | 50.27 cm² |
| 5 cm | 5 cm | 78.54 cm² |
Example of Venn Diagram Shader Calculator
Consider a scenario where a teacher uses the Venn Diagram Shader Calculator for an educational activity on set theory.
Given Data:
- Radius of circle for sports enthusiasts (Group A), R1 = 3 cm.
- Radius of circle for music enthusiasts (Group B), R2 = 4 cm.
Steps:
- Input the radii into the calculator: 3 cm for Group A and 4 cm for Group B.
- Calculate the overlap area using the formula C = pi * (min(R1, R2))^2.
Calculation:
- Minimum radius, min(R1, R2) = 3 cm.
- Overlap area, C = pi * (3)^2 = 28.27 cm².
Most Common FAQs
A1: The calculator is highly accurate for standard uses, assuming the circles are perfect. For more complex or irregular shapes, the results should be used as an approximation.
A2: Currently, the calculator is optimized for two sets. For multiple sets, adjustments and approximations might be necessary.