The Tangent Circle Calculator computes the radius of a circle that is tangent to two other given circles. This tool is particularly useful in complex geometric designs where precise calculations are necessary to ensure that elements are correctly align and proportioned.
Formula of Tangent Circle Calculator
The radius of a tangent circle, r, can be calculate using the formula:
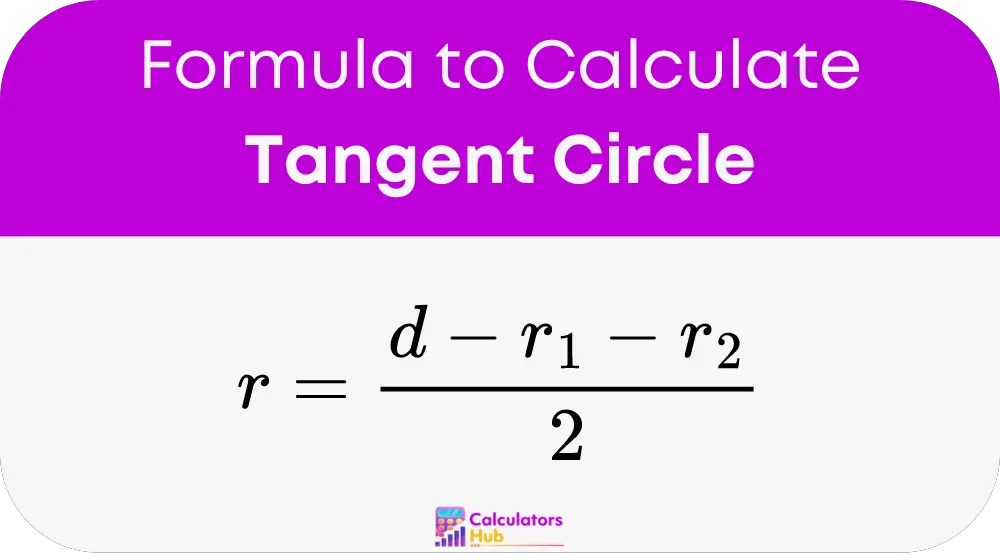
Where:
- r represents the radius of the tangent circle.
- d is the distance between the centers of the two given circles.
- r1 and r2 are the radii of the two given circles.
This formula is based on the assumption that the two given circles are externally tangent. For internally tangent circles, the approach remains consistent, but d represents the difference in radii instead of the sum.
Table of Common Calculations
For ease of use, below is a table with commonly sought combinations of r1, r2, and d, along with their resulting tangent circle radius:
| r1 (cm) | r2 (cm) | d (cm) | Tangent Circle Radius (r) (cm) |
|---|---|---|---|
| 5 | 3 | 16 | 4 |
| 7 | 2 | 20 | 5.5 |
| 4 | 4 | 18 | 5 |
This table serves as a quick reference to avoid manual calculations every time, enhancing the efficiency of design processes.
Example of Tangent Circle Calculator
Consider two circles with radii 5 cm and 3 cm, and the distance between their centers is 16 cm. Using our formula:
r = (16 - 5 - 3) / 2 = 4 cm
The radius of the tangent circle is thus 4 cm.
Most Common FAQs
A1: The formula provided assumes the circles do not overlap. For overlapping circles, adjustments in the formula are require based on specific geometric conditions.
A2: Yes, the Tangent Circle Calculator is design to handle both integer and decimal inputs, ensuring accurate calculations regardless of the units.
A3: Yes, online versions are available and provide interactive interfaces for quick calculations without manual input errors.