A square root calculator with fractions is designed to simplify the process of finding the square root of a fraction, which is a common requirement in various fields of mathematics, science, and engineering. This tool helps in breaking down complex problems involving fractions into simpler steps, thereby providing accurate results quickly and efficiently.
Formula of Square Root Calculator with Fractions
To calculate the square root of a fraction, the formula used is:
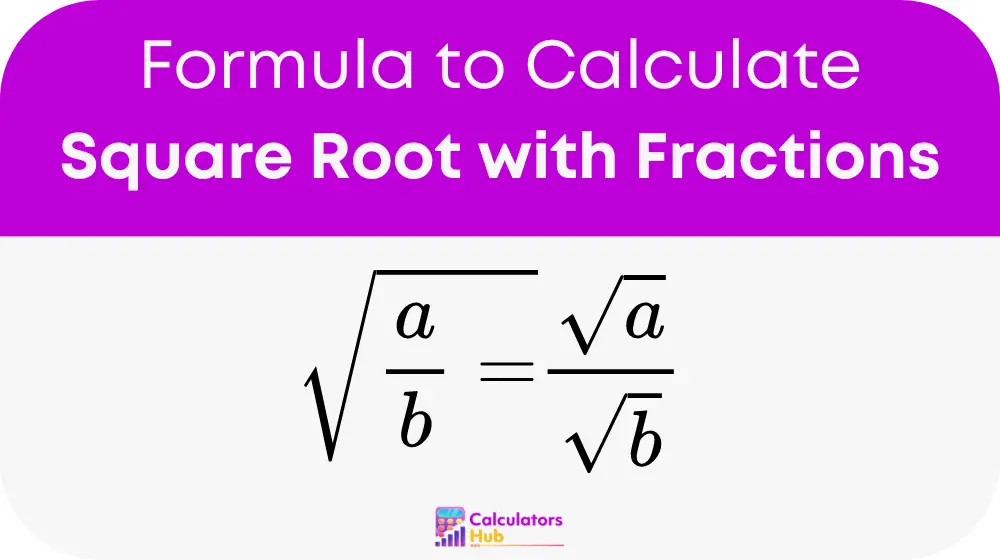
Where:
- sqrt(a/b) represents the square root of the fraction a over b.
- sqrt(a) represents the square root of the numerator.
- sqrt(b) represents the square root of the denominator.
Example:
To find the square root of 4/9:
sqrt(4/9) = sqrt(4) / sqrt(9)
Since the square root of 4 is 2 and the square root of 9 is 3:
sqrt(4/9) = 2/3
Thus, the square root of 4/9 is 2/3.
Quick Reference Table for Common Fractional Square Roots
This table provides the square roots for commonly used fractional values, enabling users to quickly find the results without manual calculations:
| Fraction | Square Root |
|---|---|
| 1/4 | 1/2 |
| 1/9 | 1/3 |
| 1/16 | 1/4 |
| 4/9 | 2/3 |
| 9/16 | 3/4 |
| 25/36 | 5/6 |
How to Use the Square Root Calculator with Fractions
- Input the Fraction: Start by entering the fraction for which the square root needs to be calculated into the calculator.
- Calculation Process: The calculator uses the formula sqrt(a/b) = sqrt(a) / sqrt(b) to compute the square root of the input fraction.
- View the Result: The result is displayed as a fraction, providing a precise and easy-to-understand output.
Most Common FAQs
A fractional square root involves finding the square root of the numerator and the denominator separately, then expressing the result as a fraction.
These calculations are highly accurate as they use straightforward arithmetic operations, which are systematically execute by the calculator to avoid errors.
Not all fractional square roots result in perfect squares; however, the calculator can simplify the square root to its lowest fractional form.