The Solve the Absolute Value Inequality Calculator is a specialized tool aimed at solving inequalities that involve absolute values. Absolute value inequalities are mathematical expressions that describe a range of values for which the absolute value of a variable expression is within certain bounds. This calculator not only simplifies calculations but also ensures accuracy, which is essential for educational purposes and critical decision-making scenarios.
Formula of Solve the Absolute Value Inequality Calculator
Step-by-Step Formula
Isolate the Absolute Value Expression
Start by subtracting ‘d’ from both sides of the inequality to isolate the absolute value expression:
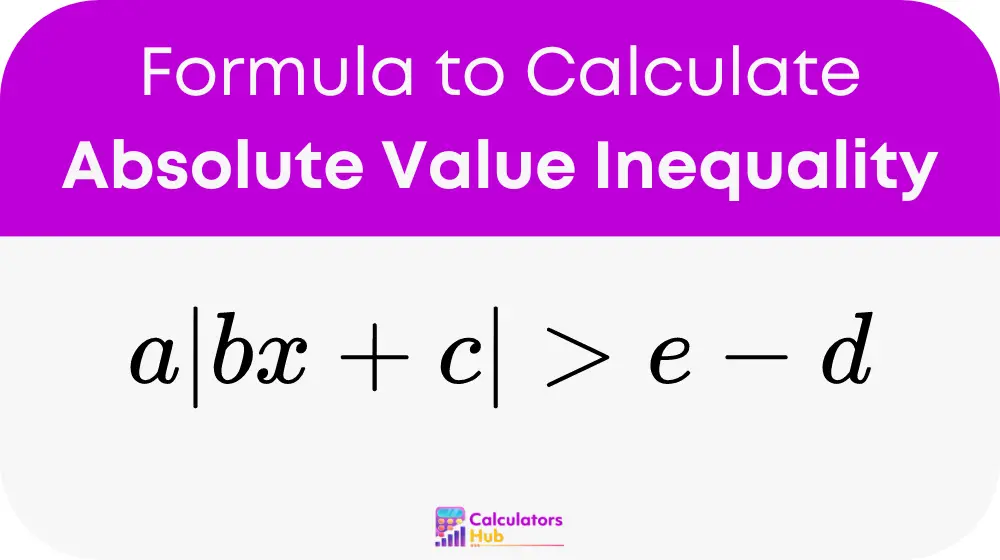
Isolating the absolute value is crucial as it simplifies the complexity of solving these inequalities.
Remove the Absolute Value
To effectively remove the absolute value, consider two cases based on the sign of the expression within the absolute value:
- Case 1: bx + c ≥ 0
- Case 2: bx + c < 0
These cases help in transforming the complex absolute value inequality into simpler, manageable forms.
Solve Each Case
For each case, reformulate the inequality:
- Case 1:
a (bx + c) > e – d
Case 2:
a (-1)(bx + c) > e - d
Each inequality is then solved for ‘x’, providing solutions that are specific to the constraints of each case.
Combine Solutions
After solving both cases, the next step is to combine the solutions to establish the final solution set that satisfies the original inequality.
Check for Extraneous Solutions
It’s essential to substitute the solutions back into the original inequality to verify their validity, as some might be extraneous or do not satisfy the inequality conditions.
General Table for Common Terms in Absolute Value Inequalities
| Term | Definition | Example Input | Example Calculation | Result Explanation |
|---|---|---|---|---|
| Isolation | Subtract/add a constant to isolate the absolute value | ` | 3x – 5 | <= 10` |
| Positive Case | When the expression inside is non-negative | ` | x + 2 | > 4` |
| Negative Case | When the expression inside is negative | ` | x + 2 | > 4` |
| Combining Solutions | Union of solutions from positive and negative cases | Positive: x > 2, Negative: x < -6 | Combine solutions | Solution set is x < -6 or x > 2 |
| Verification | Plugging solutions back to check correctness | x = -7 fits ` | x + 2 | > 4` |
| Non-Linear Terms | Involves squares or higher powers inside absolute value | ` | x^2 – 9 | < 4` |
| Extraneous Solutions | Solutions that do not satisfy the original inequality | ` | 2x + 1 | = 3solved asx = 1` |
| Interval Notation | Expression of solution sets in interval format | Combine x < -6 or x > 2 | Write in interval | Solution set in interval notation: (-∞, -6) U (2, ∞) |
| Absolute Value Removal | Replace absolute value with positive and negative parts | ` | x – 4 | <= 7` |
Example of Solve the Absolute Value Inequality Calculator
Consider the inequality 3 |2x + 5| > 15. Using the calculator:
- Subtract 15 from both sides:
3 |2x + 5| – 15 > 0
Isolate the absolute value:
|2x + 5| > 5
Solve for both cases (2x + 5 ≥ 0 and 2x + 5 < 0), and combine the solutions:
x > 0 or x < -5
This example demonstrates the calculator’s practical application and its effectiveness in simplifying complex calculations.
Most Common FAQs
An absolute value inequality like |x| < a describes all values x that are within the distance ‘a’ from zero on the number line. It combines understanding of both magnitude and distance, which are foundational in solving these problems.
Always check your solutions by substituting them back into the original inequality to ensure they satisfy the given conditions. The calculator is designed to minimize errors, but manual verification is recommended.
While the calculator is equipped to handle a broad range of absolute value inequalities, there might be complex expressions or higher-level calculations that require professional mathematical software or manual intervention.
