Interval set builder notation simplifies the process of describing sets by specifying the range of values they contain. This calculator assists users by automating the calculation process, ensuring accuracy and saving time. It is especially useful in educational settings, research, and whenever precise mathematical modeling is required.
Formula of Interval Set Builder Notation Calculator
Set builder notation is elegantly simple yet powerful. It is represent as:
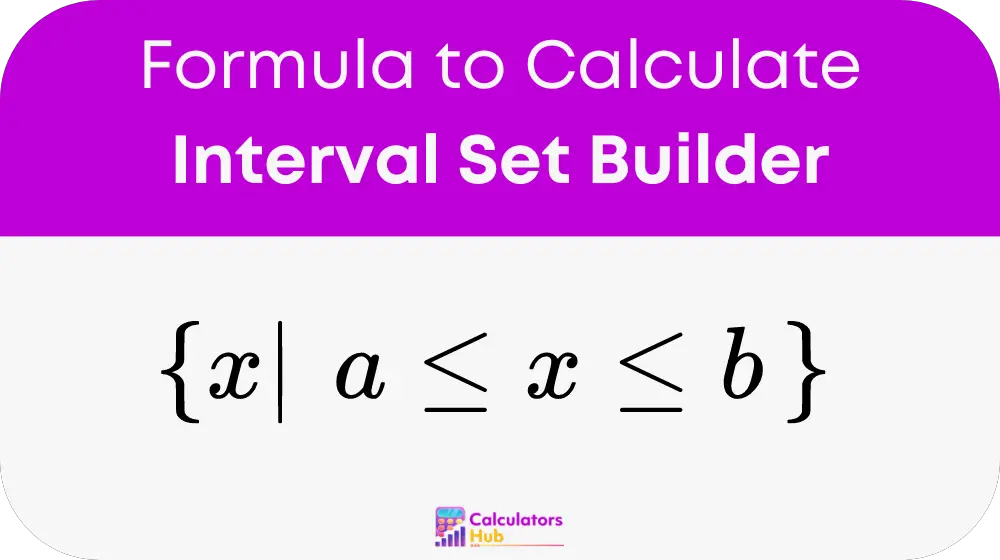
Where:
- x represents the variable or elements of the set.
- a is the lower bound (inclusive) of the interval.
- b is the upper bound (inclusive) of the interval.
This notation is fundamental in expressing mathematical ideas and solutions clearly and efficiently.
Table for General Terms
The following table includes general terms associated with interval set builder notation:
| Term | Definition |
|---|---|
| Interval | The range between two points, including both points |
| Bounds | The smallest and largest values in the set |
| Set | A collection of distinct elements |
These terms help in understanding and using the notation effectively.
Example of Interval Set Builder Notation Calculator
Consider the set of all x such that 5 ≤ x ≤ 10. Using the interval set builder notation:
{x | 5 ≤ x ≤ 10}
This defines a set from 5 to 10, inclusive of both bounds, which the calculator can quickly compute and verify.
Most Common FAQs
Set builder notation defines a set by a specific condition or rule, while interval notation directly lists the bounds of the set.
It is widely use in problem-solving where conditions are specific within a range, such as in programming, engineering, and statistics.
While highly efficient, the calculator is bind by the conditions explicitly state in the notation and does not handle ambiguous ranges.
