The fundamental counting principle is a cornerstone of combinatorial mathematics. It states that if one event can occur in n1 ways and a second can occur independently of the first in n2 ways, then the two events can occur in n1 x n2 ways. The calculator built around this principle allows users to input the number of ways each event can occur and quickly computes the total number of possible outcomes, eliminating the need for manual multiplication and reducing the margin for error.
Formula of Fundamental Counting Principle Calculator
At the heart of the fundamental counting principle calculator is the formula:
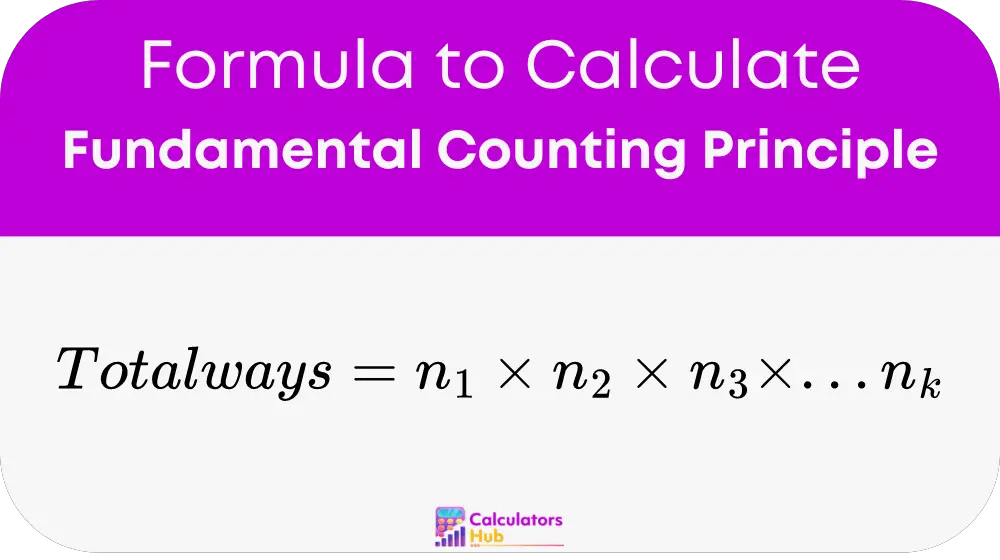
Where:
n1, n2, n3, ..., nk are the number of ways each respective operation or event can occur. This straightforward calculation forms the backbone of the calculator's functionality, providing rapid answers to complex combinatorial problems.
Utility Table for Common Calculations
To enhance understanding and utility, here is a table of common scenarios where the fundamental counting principle is applicable:
| Scenario | Description | Calculation | Result |
|---|---|---|---|
| Choosing outfits | Number of shirts (4), pants (3), shoes (2) | 4 x 3 x 2 | 24 |
| Meal options | Types of starters (3), mains (5), desserts (2) | 3 x 5 x 2 | 30 |
| Project teams | Team leaders (2), Developers (4), Designers (3) | 2 x 4 x 3 | 24 |
This table provides a quick reference for users to understand how to apply the calculator to everyday situations.
Example of Fundamental Counting Principle Calculator
Imagine planning a three-course meal where you can choose from three starters, five main courses, and two desserts. Using the fundamental counting principle, the total number of different meals you can create is calculated as follows:
- Total meal combinations = 3 (starters) x 5 (mains) x 2 (desserts) = 30 unique meals.
Most Common FAQs
The fundamental counting principle helps in calculating the total number of ways multiple independent events can occur. It is crucial for accurate predictions in probability and efficient planning in everyday decisions.
Input the number of ways each independent event can occur into the calculator. The tool will multiply these figures to provide the total number of possible outcomes.
Absolutely. By providing quick calculations on potential outcomes, it aids in making informed decisions in business, event planning, and educational activities.