A Focal Length Calculator for Parabolas is a tool designed to simplify the process of determining the focal length of a parabola. The focal length is a critical parameter in the design and analysis of parabolic mirrors, lenses, and various other optical devices. It represents the distance from the vertex of the parabola to its focus, a point where parallel rays incident towards the parabola converge after reflection.
Formula of Focal Length Calculator Parabola
The foundational equation for calculating the focal length (f) of a parabola is:
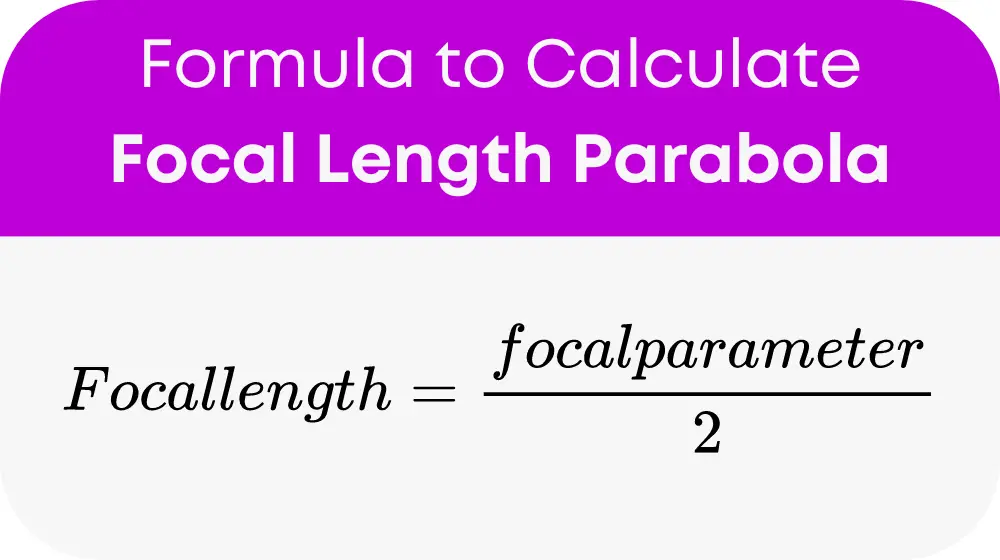
This formula becomes a practical utility once you ascertain the focal parameter (p) from the parabola’s equation. Different forms of the equation provide various methods to determine p, which then facilitates the computation of f.
Standard form (for vertical parabolas):
y = 1/(4f) (x – h)^2 + k
In this configuration, where (h, k) denotes the vertex, the focal parameter (p) is directly equivalent to twice the focal length (f).
Vertex form:
y = a(x – h)^2 + k
Here, the focal parameter (p) equals 1 / |a|. Having determined p, you can easily calculate f using the initial formula.
General form (requires conversion):
Ax^2 + Bxy + Cy^2 + Dx + Ey + F = 0
In cases where the parabola’s equation appears in its general form, a conversion to either standard or vertex form is necessary to utilize the focal length formula effectively.
General terms that people search
| Parabola Equation Form | Parameter Description | Focal Length Calculation | Example Equation | Calculated Focal Length |
|---|---|---|---|---|
| Standard Form (Vertical Parabolas) | a in y = (1/(4a))(x – h)^2 + k where (h, k) is the vertex | f = 1/(4 | a | ) |
| Vertex Form | a in y = a(x – h)^2 + k where (h, k) is the vertex and a determines the width and direction of the parabola | f = 1/(4 | a | ) |
| General Form (needs conversion) | Ax^2 + Bxy + Cy^2 + Dx + Ey + F = 0 (convert to another form to apply focal length calculation) | Convert to standard or vertex form first | Ax^2 + Bxy + Cy^2 + Dx + Ey + F = 0 | Convert and calculate |
Example of Focal Length Calculator Parabola
Consider a parabola with the vertex form equation y = 2(x – 3)^2 + 4. To find its focal length, we first identify a = 2, which means the focal parameter p = 1/|a| = 1/2. Applying our formula, the focal length (f) equals p/2 = 1/2/2 = 1/4 units from the vertex.
Most Common FAQs
The focal length determines how a parabola reflects light or radio waves. Influencing the design and functionality of telescopes, satellite dishes, and solar panels.
No, the focal length is always a positive value. Representing a physical distance from the vertex to the focus of a parabola.
The orientation (vertical or horizontal) does not affect the calculation of the focal length. However, it influences the parabola’s equation form, which is used in the calculation process.