The Earth Curvature Calculator provides a mathematical way to estimate the distance from a viewer to the horizon based on the viewer's height above sea level. It uses basic geometry to give us a clearer picture of how the Earth's curvature affects what we can see from different heights.
Formula of Earth Curvature Calculator
To understand the distance to the horizon, we can use a simple geometric principle involving the Pythagorean theorem. Consider a right triangle formed by:
- The Earth's radius, approximately 6,371 km, as one leg.
- Your elevation above sea level as the other leg (h).
- The hypotenuse extending from your viewing point to the horizon (a).
The formula to calculate the distance to the horizon (a) is:
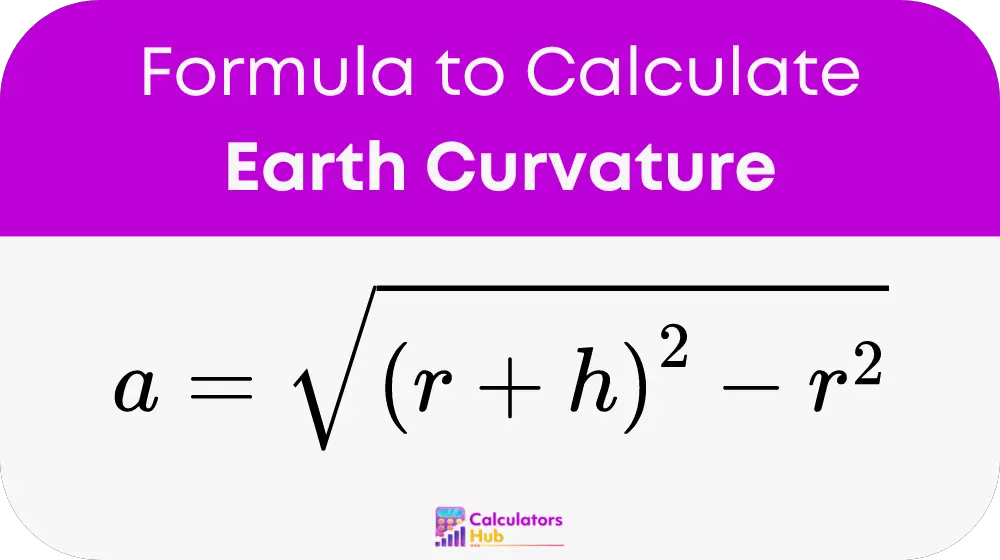
Where 'r' is the radius of the Earth and 'h' is your height above sea level. This formula helps calculate how far away the horizon is from your viewpoint, enhancing our understanding of Earth's vast scale.
Useful Tables and Tools
For practical purposes, here's a table that provides pre-calculated horizon distances for different heights, assuming standard conditions and Earth's average radius:
| Height Above Sea Level (m) | Distance to Horizon (km) |
|---|---|
| 1 | 3.57 |
| 10 | 11.3 |
| 100 | 35.7 |
| 1000 | 113 |
These values help users quickly reference the distance to the horizon without performing calculations each time. For interactive calculations, online Earth curvature calculators are available that can provide more precise measurements based on specific inputs.
Examples of Earth Curvature Calculator
For instance, a surveyor working on a coastal project needs to understand how far they can see to effectively plan their measurements. If they are standing 20 meters above sea level, using our formula, the horizon would be approximately 16 kilometers away.
Most Common FAQs
The Earth's radius is crucial as it forms the base measurement from which we derive our calculations of the curvature. It determines how quickly the surface falls away from a viewer.
The accuracy largely depends on the precision of the input values and the assumption that the Earth is a perfect sphere. For most practical purposes, it is sufficiently accurate.
Yes, the calculator can be used for high altitudes, but keep in mind that the higher you go, atmospheric effects and the increased radius might require adjustments to the basic formula.