The Divide Circle into Equal Parts Calculator is an innovative tool designed to simplify geometry calculations, specifically for dividing a circle into multiple, equal segments. This calculator eliminates the need for manual computation, making it an invaluable resource for students, educators, designers, engineers, and anyone involved in geometrical designs or projects. By inputting the desired number of divisions, users receive precise measurements instantly, ensuring accuracy and saving time.
Formula of Divide Circle into Equal Parts Calculator
To achieve its function, the calculator utilizes a straightforward formula:
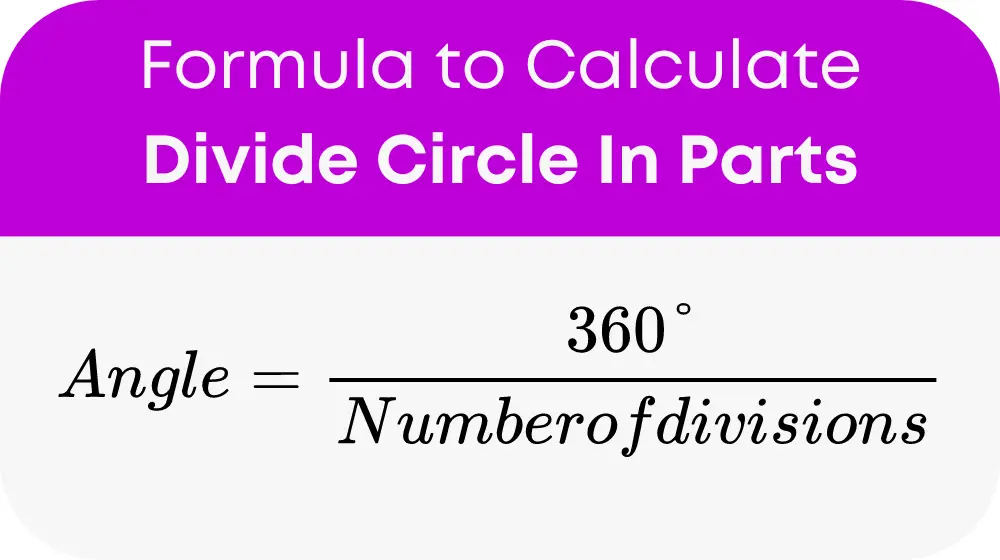
- Angle between divisions is the angle form between each division in degrees.
- 360° represents the total angle in a circle.
- Number of divisions is the number of equal parts you want to divide the circle into.
This formula is the cornerstone of the calculator’s operation, providing a quick and accurate method to partition a circle into equal sections without the complexity traditionally associated with such tasks.
General Terms and Conversions Table
Below is a table design to assist in understanding general terms and applying the calculator without the need for calculations each time. It also includes conversions and other relevant information to enhance the utility of the calculator.
| Number of Divisions | Angle Between Divisions (Degrees) |
|---|---|
| 2 | 180 |
| 3 | 120 |
| 4 | 90 |
| 5 | 72 |
| 6 | 60 |
| 7 | Approximately 51.43 |
| 8 | 45 |
| 9 | 40 |
| 10 | 36 |
This table serves as a quick reference, allowing users to easily visualize and plan the division of a circle into equal parts without directly using the calculator for common division numbers.
Example of Divide Circle into Equal Parts Calculator
Let’s consider a practical example to illustrate how the Divide Circle into Equal Parts Calculator works. Assume you’re a designer working on a project that requires you to divide a circle into 5 equal parts. By entering the number 5 into the calculator, you would use the formula as follows:
Angle between divisions = 360° / 5 = 72°
Thus, each division must be made at an angle of 72° to ensure equal parts. This example demonstrates the calculator’s ability to provide quick and accurate results, streamlining the design process.
Most Common FAQs
The calculator is highly accurate, utilizing a simple yet effective formula to ensure precision in dividing a circle into equal parts. Its accuracy is dependent on the correct input of the number of divisions.
Yes, the calculator is versatile and can be use for circles of any size. The size of the circle does not affect the calculation of the angles between divisions.
The calculator is design to handle any number of divisions. If the desire number of divisions is not list in the general terms table, simply input the number into the calculator to obtain the specific angle needed for your project.